Question

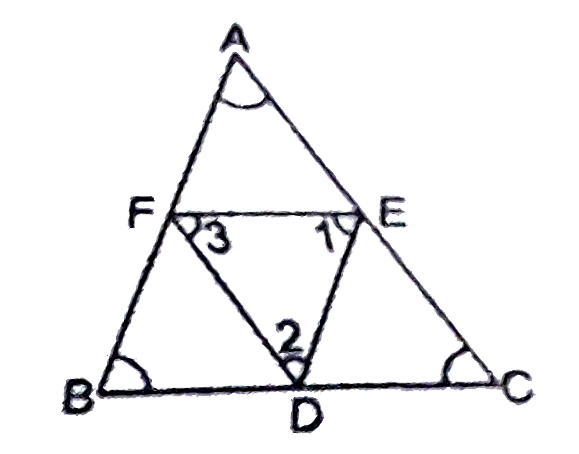
If $D,E,F$
are the mid-points of the sides $BC,CAandAB$
respectively of a triangle $ABC,$
prove by vector method that
$AreaofDEF=\frac{1}{4}\left(areaofABC\right)\stackrel{.}{}$

Explanation

Given: `triangleABC` in which , D E and F are mid-point if the sides, BC, CA and AB respectively.
To porve: To determine the ratio of the areas of `triangleDEF and triangleABC`
proof: Since D,E,F are the mid-point
EF||BC and `EF= 1/2 BC`
EF=BD
Similarly, DE= BF ( ` DE||AB and 1/2 AB) `
So, EFBD is a parallelogram.
`angleB= angle1`
Similarly , AFDE and EFDC and parallelogram
` Rightarrow angleA = angle2 and angleC= angle3`
`i.e. triangleABC and triangleDEF` are equiangular
` Rightarrow triangleDEF ~ triangleABC`
`(ar(triangleDEF))/(ar(triangleABC))= (DE^(2))/((AB^(2)))= (FB^(2))/(AB^(2))= ((AB//2)^(2))/(AB^(2)) = 1/4`
Hence ((ar(triangleDEF))/((ar(trangleABC))= 1/4`