Dr. Bansal needs to determine the distance PQ across a river in and east-west direction as shown in the adjoining figure. He can't measure this distance directly over the water. So, he selects the point S from where a straight line to point Q stays on land so he can mesure distance. he then moves eastward a distance of 400 m from point S to T , so that the line of sight from point T to P cuts the previous line SQ at R. finally with a long measuring tape. he determines that. SR = 250 m, QR= 1250 m
Determine if this is enough information to calculate the distance PQ and if so, find PQ and hence find the time taken by a swimmer to cross the river PQ with a uniform speed of 800 m/hr.
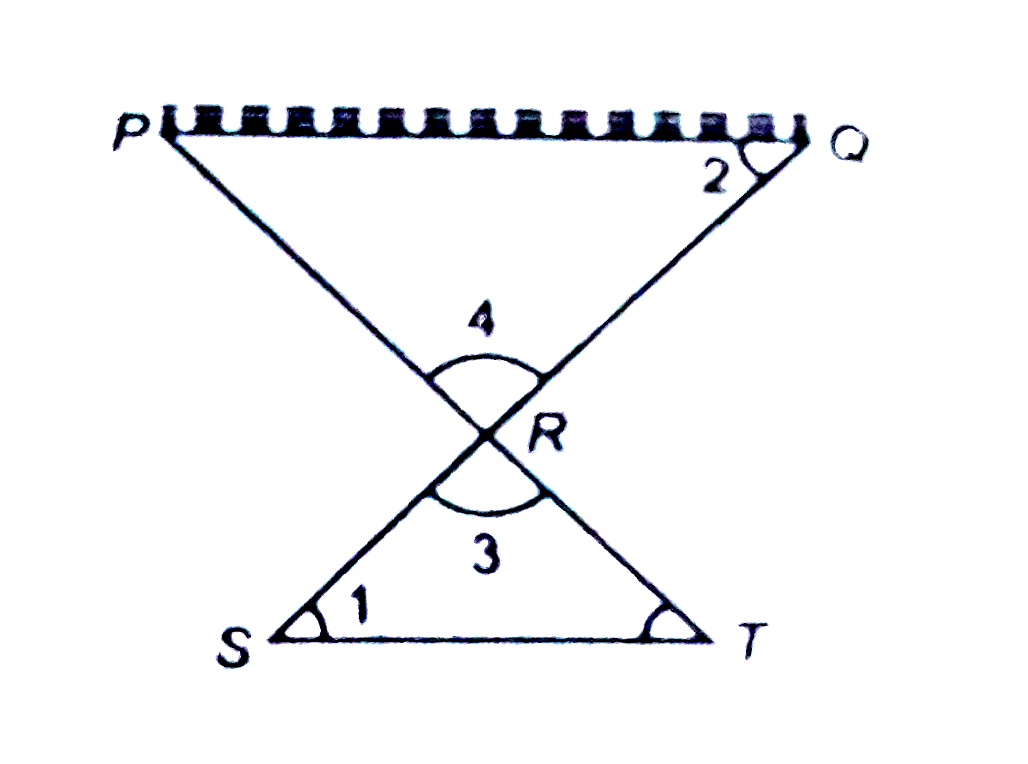
In △SRTand△PQR
∠3=∠4 ( vertically oppostie angles)
∠1=∠2 ( alternate angles)
△SRT~△QRP ( AA corollary)
SRQR=RTRP=STQP (corresponding sides of similar triangles are proportional)
 ( since he moves eastward, a distance of 400 m from point S )
( since he moves eastward, a distance of 400 m from point S )
⇒PQ=400×1250250=2000m
Hence, width of the river= 2000 m
A swimmer swims 800 m in 60min.
A swimmer swims 1 m in 60800 min
A swimmer swims 2000 m in 60800×2000min
150 min = 2 hr and 30 minutes.
Topper's Solved these Questions
- TRIANGLES Book:NAGEEN PRAKASHAN ENGLISHChapter:TRIANGLES Exercise:Problems From NCERT/ Exemplar
Explore 14 Videos
- TRIANGLES Book:NAGEEN PRAKASHAN ENGLISHChapter:TRIANGLES Exercise:Exercise 6a
Explore 24 Videos
- STATISTICS Book:NAGEEN PRAKASHAN ENGLISHChapter:STATISTICS Exercise:Revision Exercise Long Answer Questions
Explore 4 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBook:NAGEEN PRAKASHAN ENGLISHChapter:VOLUME AND SURFACE AREA OF SOLIDSExercise:Revisions Exercise Long Answer Questions
Explore 5 Videos
Similar Questions
Knowledge Check
The speed-time graph of a particle moving along a fixed direction as shown in the figure. The distance traversed by the particle between t = 0 s to t = 10 s is
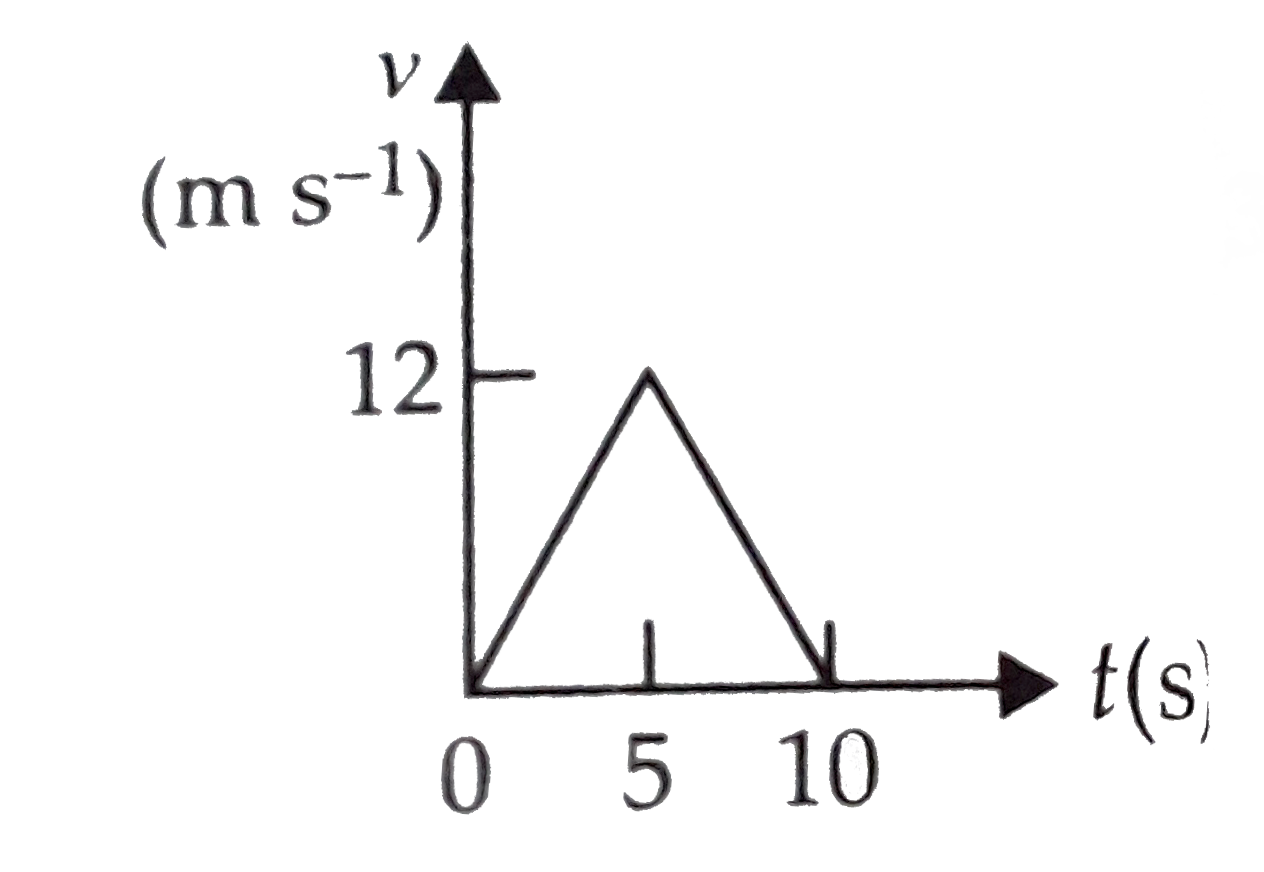 A20 mB40 mC60 mD80 m
A20 mB40 mC60 mD80 m
A point moves such teat its distance from the point (4,0) is half that of its distance from the line x=16, find its locus.
View SolutionA point moves so that its distance from the point (−2,3) is always three times its distance from the point (0,3). Find the equation to its locus.
View SolutionA man wants to reach point B on the opposite bank of a river flowing at a speed as shown in figure. What minimum speed relative to water should the man have so that he can reach point B? In which direction should he swim?
View SolutionFind the direction in which a straight line must be drawn through the point (1,2) so that its point of intersection with the line x+y=4 may be at a distance of 3 units from this point.
View SolutionFind the direction in which a straight line must be drawn through the point (1,2) so that its point of intersection with the line x+y = 4 may be at a distance of 3 units from this point.
View SolutionThe locus of a point which moves so that the difference of the squares of its distance from two given points is constant, is a
View SolutionFind the locus of a point which moves so that its distance from the x-axis is twice its distance from the y-axis.
View Solution