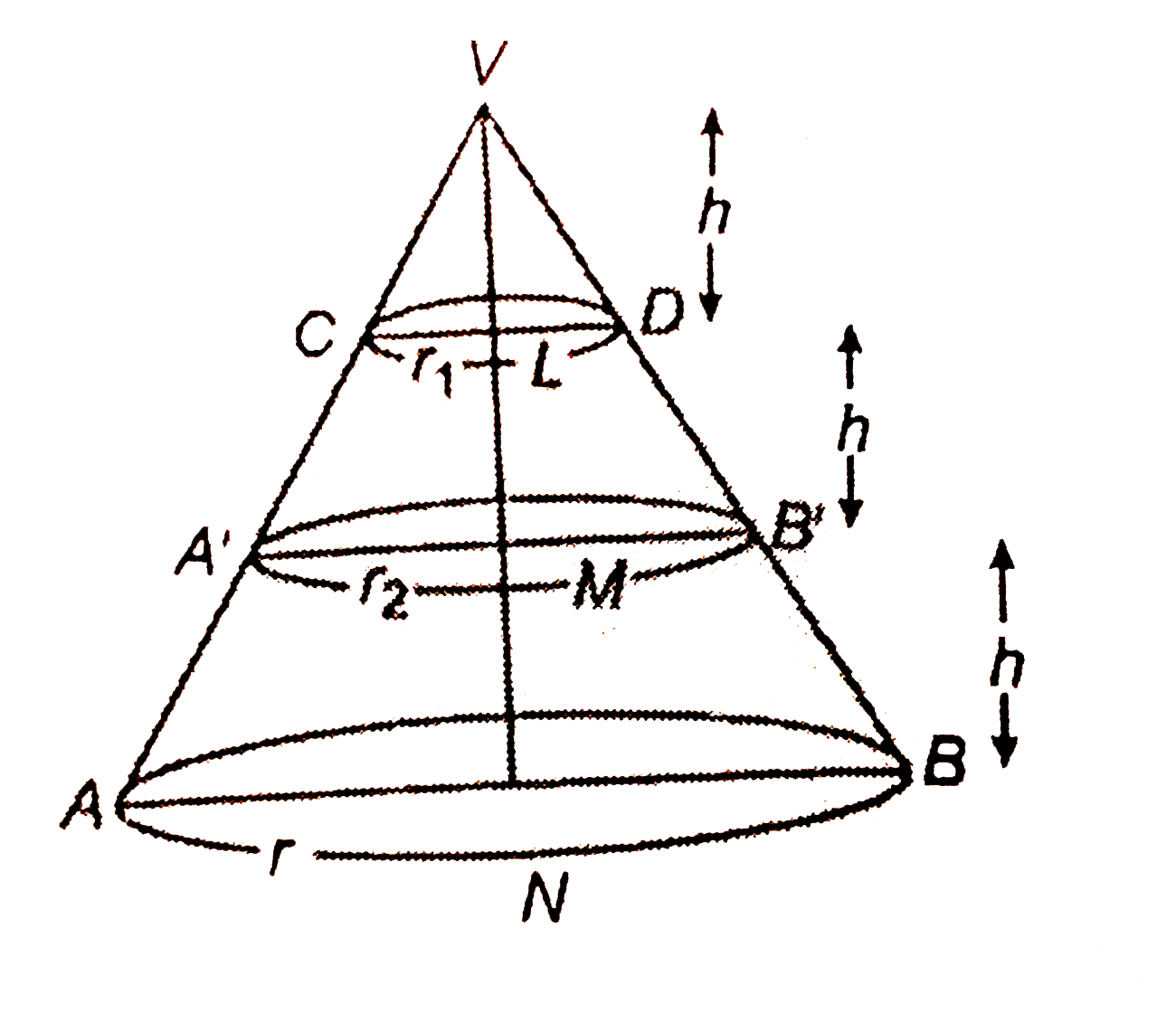
The height of a right circular cone is trisected by two planes drawn parallel to the base. Show that the volumes of the three portions starting from the top are in the ratio 1:7:19.
Since height is trisected , therefore by basic proportionality there m, base radii of three cones VCD,VA'B' and VAB are also in the ratio 1:2:3.
Let volume of cone VCD=V =13πr22h
Volume of cone VAB=13πr21(2h)=8×13πr22h=8V ltbRgt and volume of cone VAB=13πr2(3h)=13π(3r2)2(3h)=27×13×13πr22h=27V
Ratio of volume of 3 portions
=volume (VCD): volume (CDB'A'): volume (A'B'BA)
=V:8V:27V-8V=:19
Topper's Solved these Questions
- VOLUME AND SURFACE AREA OF SOLIDSBook:NAGEEN PRAKASHAN ENGLISHChapter:VOLUME AND SURFACE AREA OF SOLIDSExercise:Problems From NCERT/exemplar
Explore 10 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBook:NAGEEN PRAKASHAN ENGLISHChapter:VOLUME AND SURFACE AREA OF SOLIDSExercise:Exercise
Explore 68 Videos
- TRIANGLES Book:NAGEEN PRAKASHAN ENGLISHChapter:TRIANGLES Exercise:Revision Exercise Long Questions
Explore 1 Video
Similar Questions
A right circular cone is divided by a plane parallel to its base in two equal volumes. In what ratio will the plane divide the axis of the cone?
View SolutionThe height of a right circular cone is 6 cm and area of its base is 18.5cm2. Find its volume.
View SolutionA solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. The ratio of the volume of the smaller cone to the whole cone is:
View SolutionThe radius of the base of a right circular cone is r. It is cut by a plane parallel to the base at aheight h from the base. The slant height of the frustum is √h2+49r2. Show that the volume of13the frustum is 1327πr2h.
View SolutionThe base radii of two right circular cones of the same height are in the ratio 3:5. Find the ratio of their volumes.
View SolutionThe base radii of two right circular cones of the same height are in the ratio 3:5. Find the ratio of their volumes.
View SolutionThe base radii of two right circular cones of the same height are in the ratio 3:5. Find the ratio of their volumes.
View SolutionThe vertical height of a right circular cone is 9 cm and radius of its base is 4 cm. Find its volume.
View SolutionThe heights of two right circular cones are in the ratio 1 : 2 and the perimeters of their bases are in the ratio 3 : 4. Find the ratio of their volumes.
View SolutionA solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. The ratio of the volume of the smaller cone to the whole cone is: 1:2 (b) 1:4 (c) 1:6 (d) 1:8
View Solution