In the following figure O is the centre of the circel. If ∠ACB=60∘ and DA=DB then prove that ΔADB is an equilateral triangle.
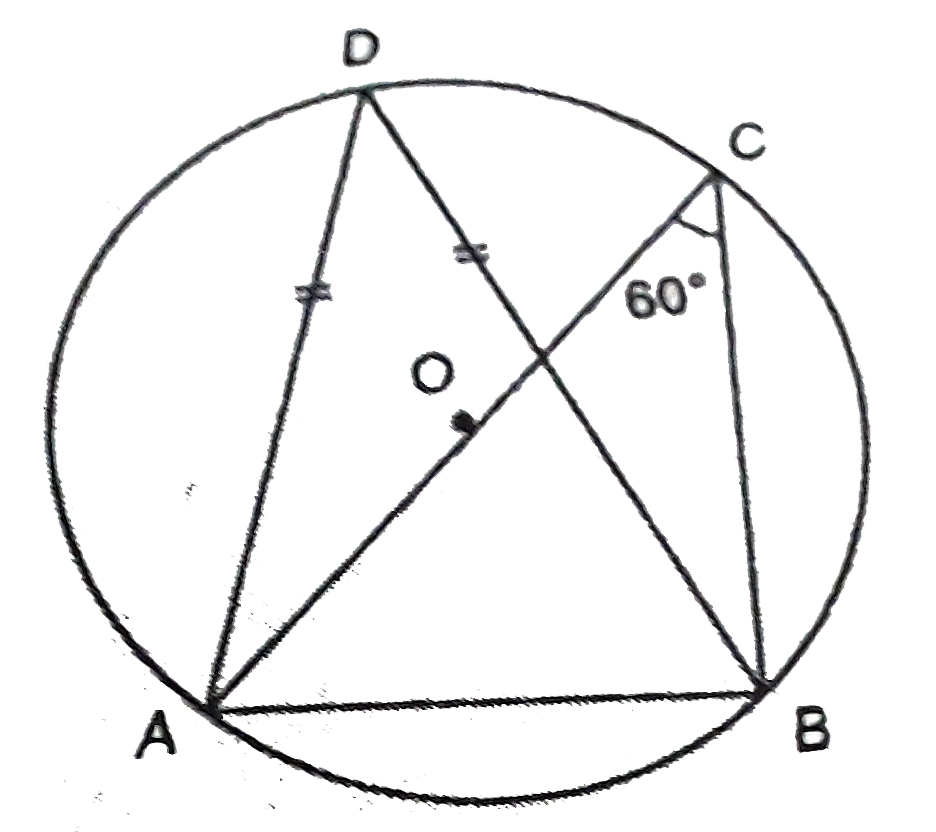
∠ADB=∠ACB (angles of same segment)
∠ADB=60∘
⇒
In ΔADB,
AD=DB
AD=DB
⇒∠ABD=∠BAD
and ∠ABD+∠BAD+∠ADB=180∘
⇒∠ABD+∠ABD+60∘=180∘
⇒2∠ABD=120∘
⇒∠ABD=60∘
∴∠ABD=∠BAD=∠ADB=60∘
⇒ΔABD is an equilateral triangle.
Topper's Solved these Questions
- CIRCLE BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise 10a
22 Videos
- CIRCLE BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise 10b
19 Videos
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Revision Exercise (long Answer Question)
5 Videos
- CO-ORDINATE GEOMETRY BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRY EXERCISE - Exercise
8 Videos
Similar Questions
Prove that each angle of an equilateral triangle is 600
View SolutionIn the adjoining figure , O is the centre of the circle. If ∠ACB=25∘, then find ∠AOB.
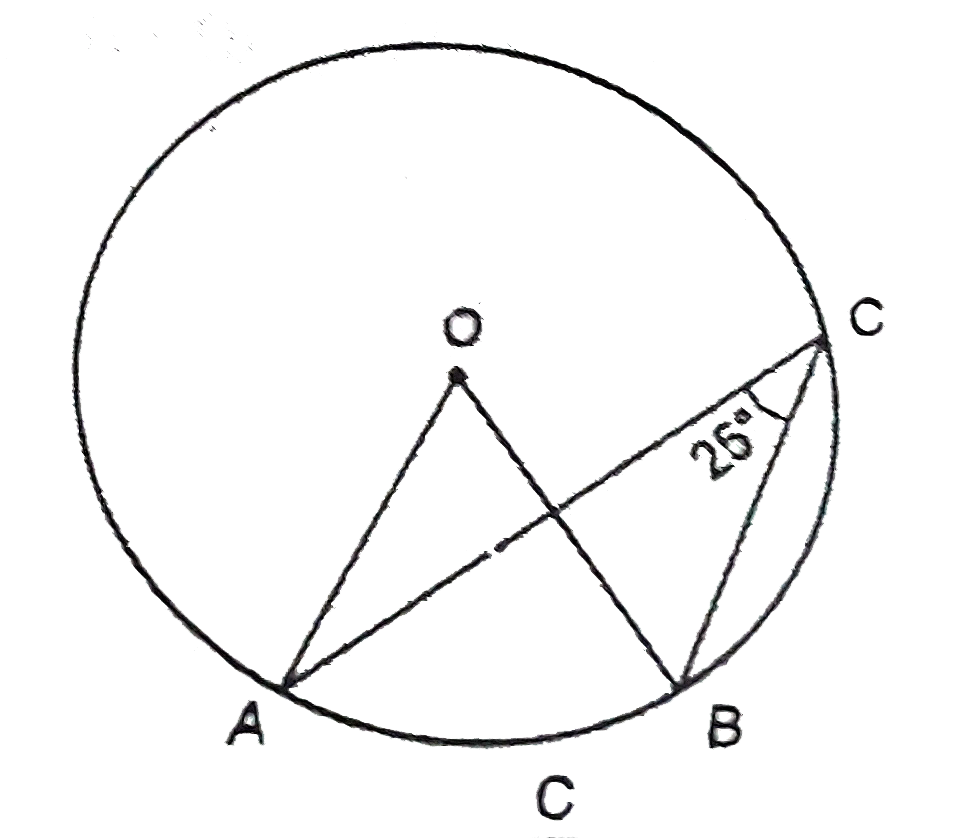
View SolutionIn the adjoining figure , O is the centre of the circle and ∠OAB=60∘. Find ∠APC.

View SolutionProve that the medians of an equilateral triangle are equal.
View SolutionProve that the medians of an equilateral triangle are equal.
View SolutionProve that measure of each of an equilateral triangle is 600
View SolutionIn the adjoining figure, O is the centre of the circle, Prove that ∠x=∠y+∠z.
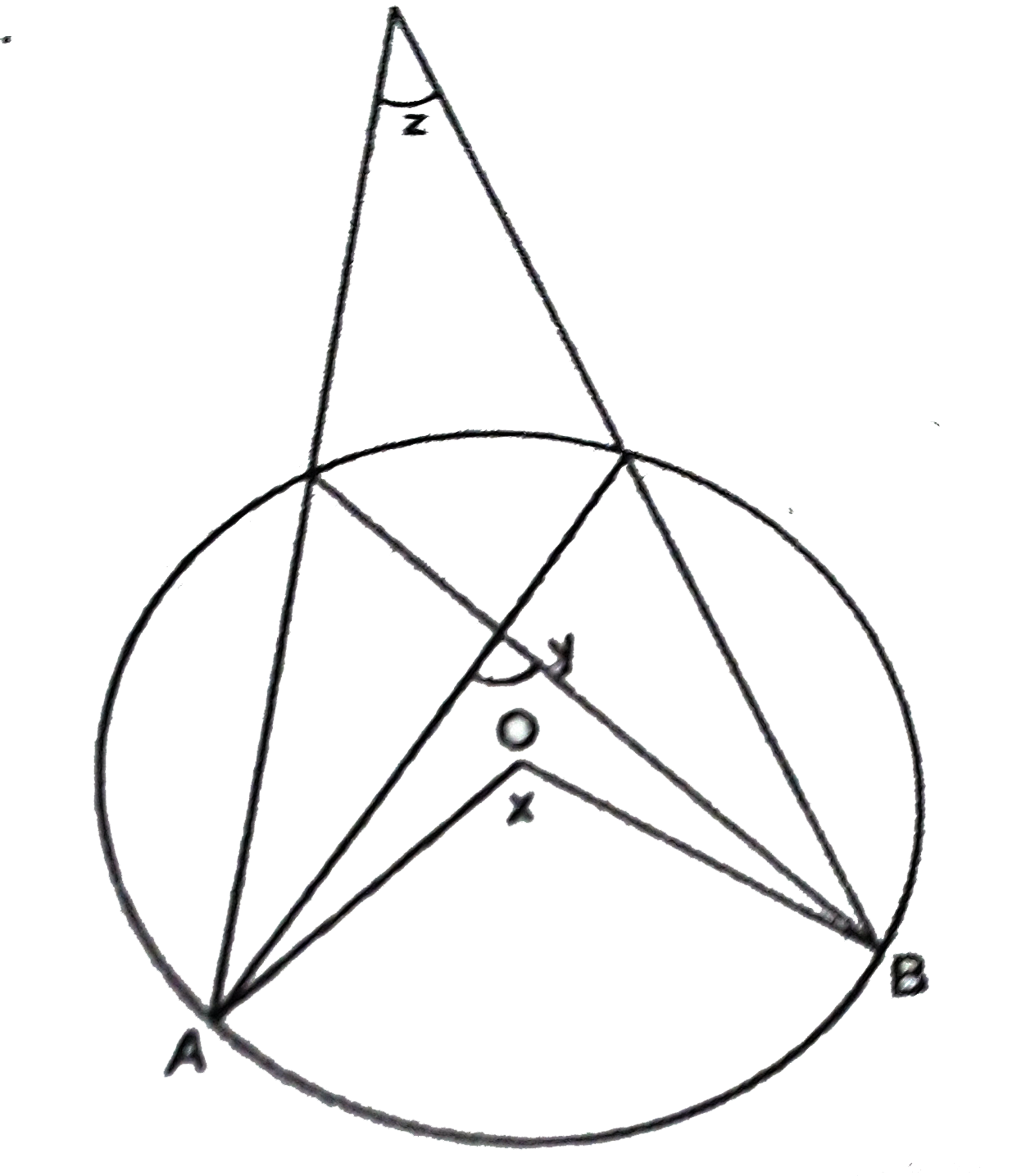
View SolutionIn figure O is the centre of the circle, prove that ∠z=∠x+∠y.

View SolutionIn the following figure ABC is a right angled triangle with ∠BAC=90∘,andAD⊥BC.
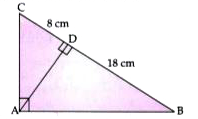
(i) Prove ΔADB~ΔCDA.
View SolutionIn Figure, O is the centre of the circle, prove that ∠x=∠y+∠z
View Solution
