Find a point on the line through A(5,−4)andB(−3,2), that is, twice as far from A as from B.
To find a point on the line through A(5,−4) and B(−3,2) that is twice as far from A as from B, we can use the section formula. Let's go through the solution step by step.
Step 1: Understand the Ratio
We are given that the distance from point P to point A is twice the distance from point P to point B. This can be expressed as:
PAPB=2
This implies that PA=2⋅PB.
Step 2: Use the Section Formula
Since PA=2⋅PB, we can express the distances in terms of a ratio:
PAPB=21
This means that point P divides the line segment AB in the ratio 2:1.
Step 3: Apply the Section Formula
The section formula states that if a point P divides the line segment joining points A(x1,y1) and B(x2,y2) in the ratio m:n, then the coordinates of point P are given by:
P(mx2+nx1m+n,my2+ny1m+n)
In our case, A(5,−4), B(−3,2), m=2, and n=1.
Step 4: Calculate the Coordinates of Point P
Using the section formula for the x-coordinate:
x=2(−3)+1(5)2+1=−6+53=−13
Now for the y-coordinate:
y=2(2)+1(−4)2+1=4−43=03=0
Thus, the coordinates of point P are:
P(−13,0)
Step 5: Consider the Second Case
Point P can also lie on the opposite side of point B such that PA=2⋅PB. In this case, we can express the distances as:
PBPA=12
This means point P divides the line segment AB in the ratio 1:1 (since B is the midpoint).
Step 6: Calculate the Midpoint
Using the midpoint formula, the coordinates of point B are:
B(−3,2)=(x1+x22,y1+y22)
Setting the coordinates:
−3=5+x2and2=−4+y2
From the x-coordinate:
−6=5+x⟹x=−11
From the y-coordinate:
4=−4+y⟹y=8
Thus, the coordinates of point P in this case are:
P(−11,8)
Final Answer
The two points on the line through A and B that are twice as far from A as from B are:
1. P(−13,0)
2. P(−11,8)
We are given that the distance from point P to point A is twice the distance from point P to point B. This can be expressed as:
PAPB=2
This implies that PA=2⋅PB.
Since PA=2⋅PB, we can express the distances in terms of a ratio:
PAPB=21
This means that point P divides the line segment AB in the ratio 2:1.
The section formula states that if a point P divides the line segment joining points A(x1,y1) and B(x2,y2) in the ratio m:n, then the coordinates of point P are given by:
P(mx2+nx1m+n,my2+ny1m+n)
In our case, A(5,−4), B(−3,2), m=2, and n=1.
Using the section formula for the x-coordinate:
x=2(−3)+1(5)2+1=−6+53=−13
y=2(2)+1(−4)2+1=4−43=03=0
P(−13,0)
Point P can also lie on the opposite side of point B such that PA=2⋅PB. In this case, we can express the distances as:
PBPA=12
This means point P divides the line segment AB in the ratio 1:1 (since B is the midpoint).
Using the midpoint formula, the coordinates of point B are:
B(−3,2)=(x1+x22,y1+y22)
Setting the coordinates:
−3=5+x2and2=−4+y2
−6=5+x⟹x=−11
4=−4+y⟹y=8
P(−11,8)
The two points on the line through A and B that are twice as far from A as from B are:
1. P(−13,0)
2. P(−11,8)
Topper's Solved these Questions
- CO-ORDINATE GEOMETRYBook:NAGEEN PRAKASHAN ENGLISHChapter:CO-ORDINATE GEOMETRYExercise:Miscellaneous Examples
Explore 8 Videos
- CO-ORDINATE GEOMETRYBook:NAGEEN PRAKASHAN ENGLISHChapter:CO-ORDINATE GEOMETRYExercise:Problems Of NCERT/exemplar
Explore 14 Videos
- CIRCLESBook:NAGEEN PRAKASHAN ENGLISHChapter:CIRCLESExercise:Long Answer Questions
Explore 2 Videos
- CONSTRUCTIONSBook:NAGEEN PRAKASHAN ENGLISHChapter:CONSTRUCTIONSExercise:Exercise 11 B
Explore 10 Videos
Similar Questions
Knowledge Check
The slope of the line through points A(3,-2) and B(-2,-3) is
A−5B−15C15D1
Find the slope of the line through the points: (3,−2)and(3,4)
View SolutionWhat are the coordinates for the point on the line AB (see figure) that is three times as far from A as from B, and that is in between point A and B?
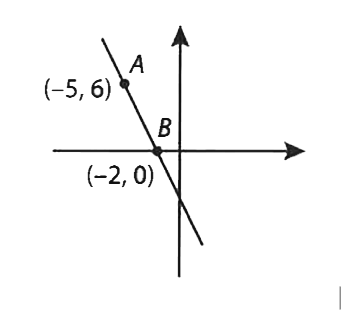
View SolutionFind the two points on the line through the points A(1,2,3)andB(3,5,9) at a distance of 14 units from the mid point of AB.
View SolutionP is a point on the line joining A (4, 3) and B (-2, 6) such that 5AP = 2BP. Find the coordinates of P.
View SolutionFind the slope of the line through the points (4,−6)(−2,−5)
View SolutionThe coordinatse of two points A and B are (−1,4)and(5,1), respectively. Find the coordinates of the point P which lie on extended line AB such that it is three times as far from B as from A.
View SolutionFind the point on the x-axis whose distances from the points A(7, 6) and B(-3, 4) are in the ratio 1 : 2.
View Solution