Find the area of the region between the two concentric circles, if the length of a chord of the outer circle just touching the inner circle at a particular point on it is 10 cm (Take, π=227)
To find the area of the region between two concentric circles, we can follow these steps:
1. Understand the Problem: We have two concentric circles (one inside the other). A chord of the outer circle touches the inner circle at a single point, and the length of this chord is given as 10 cm.
2. Identify the Geometry: Let:
- O be the center of both circles.
- R be the radius of the outer circle.
- r be the radius of the inner circle.
- AB be the chord of the outer circle, which touches the inner circle at point P.
3. Divide the Chord: Since the chord AB touches the inner circle at point P, it divides the chord into two equal segments. Therefore, if the length of the chord AB is 10 cm, then:
AP=PB=102=5 cm
4. Use Right Triangle Properties: In triangle OAP:
- OA is the radius of the outer circle, which is R.
- OP is the radius of the inner circle, which is r.
- AP is half the length of the chord, which we found to be 5 cm.
5. Apply the Pythagorean Theorem: According to the Pythagorean theorem:
OA2=OP2+AP2
Substituting the known values:
R2=r2+52
R2=r2+25
6. Calculate the Area of Both Circles:
- The area of the outer circle is:
Areaouter=πR2
- The area of the inner circle is:
Areainner=πr2
7. Find the Area Between the Two Circles:
Areabetween=Areaouter−Areainner=πR2−πr2
From step 5, we know:
R2−r2=25
Therefore:
Areabetween=π(R2−r2)=π⋅25
8. Substitute the Value of π:
Given that π=227:
Areabetween=227⋅25
9. Calculate the Final Area:
Areabetween=22⋅257=5507≈78.57 cm2
Final Answer:
The area of the region between the two concentric circles is approximately 78.57 cm2.
- O be the center of both circles.
- R be the radius of the outer circle.
- r be the radius of the inner circle.
- AB be the chord of the outer circle, which touches the inner circle at point P.
AP=PB=102=5 cm
- OA is the radius of the outer circle, which is R.
- OP is the radius of the inner circle, which is r.
- AP is half the length of the chord, which we found to be 5 cm.
OA2=OP2+AP2
Substituting the known values:
R2=r2+52
R2=r2+25
- The area of the outer circle is:
Areaouter=πR2
- The area of the inner circle is:
Areainner=πr2
Areabetween=Areaouter−Areainner=πR2−πr2
From step 5, we know:
R2−r2=25
Therefore:
Areabetween=π(R2−r2)=π⋅25
Given that π=227:
Areabetween=227⋅25
Areabetween=22⋅257=5507≈78.57 cm2
The area of the region between the two concentric circles is approximately 78.57 cm2.
Topper's Solved these Questions
- AREA RELATED TO CIRCLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - AREA RELATED TO CIRCLESEXERCISE - Problems From NCERT /exemplar
25 Videos
- AREA RELATED TO CIRCLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - AREA RELATED TO CIRCLESEXERCISE - Exercise 12 A
24 Videos
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Revision Exercise Long Answer Question
5 Videos
Similar Questions
In two concentric circles prove that all chords of the outer circle which touch the inner circle are of equal length.
View SolutionProve that in two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact.
View SolutionFind the area of a ring shaped region enclosed between two concentric circles of radii 20 cm and 15 cm.
View SolutionFind the area of a ring shaped region enclosed between two concentric circles of radii 20 cm and 15 cm.
View SolutionThe area enclosed between the concentric circles is 770 cm2 . If the radius of the outer circle is 21 cm, find the radius of the inner circle.
View SolutionTwo concentric circles are of radii 13 cm and 5 cm. Find the length of the chord of the larger circle which touches the inner circle.
View SolutionIf radii of two concentric circles are 4 cm and 5 cm, then length of each chord of one circle which is tangent to the other circle, is
View SolutionTwo concentric circle of radii 3cm and 5cm are given. Find the chord BC which touches the inner circle at P.
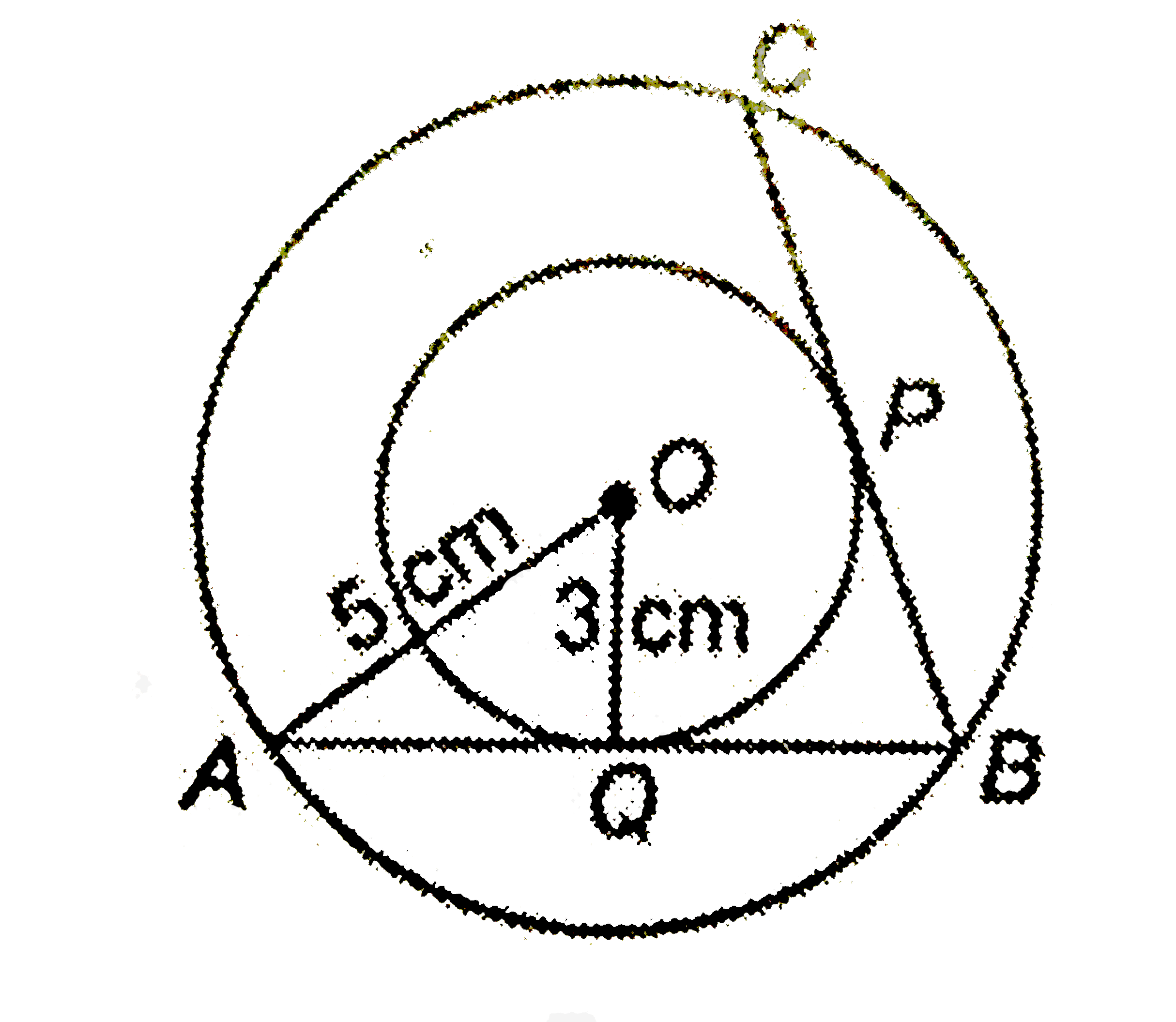
View SolutionFind the circumference of a circle of diameter 21 cm. Take π=227.
View SolutionIf the radii of two concentric circles are 4 cm and 5 cm, then find the length of each chord of one circle which is tangent to the other circle.
View Solution
