A manufacturer produces three products x, y, z which he sells in two markets. Annual sales are indicated below: Market Products I 10.000 2.000 18.000 II 6.000 20.000 8.000 (a) If unit sale prices of x, y and z are Rs 2.50, Rs 1.50 and Rs 1.0
Let the matrix A respesents the production .
∴A=[100002000180006000200008000]
(A) Let the matrix B respesents the selling price per unit ,
∴B=⎡⎢⎣2.501.501.00⎤⎥⎦
Now,AB=[100002000180006000200008000]⎡⎢⎣2.501.501.00⎤⎥⎦
=[25000+3000+1800015000+30000+8000]=[4600053000
∴ Totoal revenue of market I=Rs.46000
Total revenuse of market II =Rs. 53000
(b) Let the matrix C represents the cost per unit.
∴C=⎡⎢⎣2.001.000.50⎤⎥⎦
Now,AC=[100002000180006000200008000]⎡⎢⎣2.001.000.50⎤⎥⎦
=[20000+2000+900012000+20000+4000]=[3100036000]
profit in market I=Rs.46000−Rs.31000=Rs.15000
profitr in market II=Rs.53000−Rs.36000=Rs.17000
Total profit =Rs.15000+Rs.17000=Rs.32000
Topper's Solved these Questions
- MATRICESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - MATRICESEXERCISE - Exercise 3.4
18 Videos
- LINEAR PROGRAMMINGBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR PROGRAMMINGEXERCISE - Miscellaneous Exercise
9 Videos
- PROBABIILITYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - PROBABIILITYEXERCISE - Miscellaneous Exercise
19 Videos
Similar Questions
[1−123] find the inverse of matrix
View Solution(Diet problem) A dietician has to develop a special diet using two foods P and Q. Each packet (containing 30g) of food P contains 12 units of calcium, 4 units of iron, 6 units of cholesterol and 6 units of vitamin A. Each packet of the same quantity of food Q contains 3 units of calcium, 20 units of iron, 4 units of cholesterol and 3 units of vitamin A. The diet requires atleast 240 units of calciums atleast 460 units of iron and at most 300 units of cholesterol. How many packets of each food should be used to minimise the amount of vitamin A in the diet? What is the minimum amount of vitamin A? How many packets of each food should be used to maximise the amount of vitamin A in the diet? What is the maximum amount of vitamin A in the diet?
View SolutionA and B are two events such that P(A)≠0. Find P(B∣A) , if (i) A is a subset of B (ii) A∩B=φ
View SolutionA manufacturer produces three products x, y, z which he sells in two markets. Annual sales are indicated below:
Market Products
I 10.000 2.000 18.000
II 6.000 20.000 8.000
(a) If unit sale prices of x, y and z are Rs 2.50, Rs 1.50 and Rs 1.00, respectively, find the total revenue in each market with the help of matrix algebra.(b) If the unit costs of the above three commodities are Rs 2.00, Rs 1.00 and 50paise respectively. Find the gross profit.
View SolutionA manufacturer produces three products x,y,z which he sells in two markets ,Annual sales are indicated below:
MarketproductsI10,0002,00018,000II6,00020,0008,000
(A) if unit sale prices of x,y and z are Rs. 2.50, Rs. 1.50 and Rs. 1.00, respectively , find the total revenue algebra .
(b) if the unit costs of the above three commodities are Rs. 2.00,Rs. 1.00 and 50 paise respectively, find the gross profit.
View Solutionएक निर्माता तीन प्रकार की वस्तुएँ x, y तथा z का उत्पादन करता है जिन का वह दो बाजारों में विक्रय करता है । वस्तुओ की वर्षिक आय बिक्री नीचे सूचित (निर्देशित) है :
बाजारउत्पाद I10,0002,00018,000 II6,00020,0008,000
(a) यदि x, y तथा z के इकाई विक्रय मूल्य क्रमश: 2.50 रु., 1.50 रु. तथा 1.00 रु. हो तो आव्यूह बीजगणित की सहायता से ज्ञात कीजिए की प्रत्येक बाजार से कुल राजस्व कितना प्राप्त होगा ।
(b) यदि उपरोक्त तीनो उत्पादों का इकाई लागत मूल्य क्रमश: 2.00 रु., 1.00 रु. तथा 50 पैसे हो, तो कुल लाभ ज्ञात करे ।
View SolutionA manufacturer produces three products x, y, z which he sells in two markets. Annual sales are indicated below:
MarketProductsI10,0002,00018,000II6,00020,0008,000
(a) If unit sale prices of x, y and z are Rs. 2.50, Rs. 1.50 and Rs. 1.00, respectively, find the total revenue in each market with the help of matrix algebra.
(b) If the unit costs of the above three commodities are Rs. 2.00, Rs. 1.00 and 50 paise respectively. Find the gross profit.
View SolutionA manufacturer produces three products x, y, z which he sells in two markets. Annual sales are indicated below:
MarketProductsI10,0002,00018,000II6,00020,0008,000
(a) If unit sale prices of x, y and z are Rs. 2.50, Rs. 1.50 and Rs. 1.00, respectively, find the total revenue in each market with the help of matrix algebra.
(b) If the unit costs of the above three commodities are Rs. 2.00, Rs. 1.00 and 50 paise respectively. Find the gross profit.
View SolutionA manufacturer produces three products x, y, z which he sells in two markets. Annual sales are indicated below:
MarketProductsI10,0002,00018,000II6,00020,0008,000
(a) If unit sale prices of x, y and z are Rs. 2.50, Rs. 1.50 and Rs. 1.00, respectively, find the total revenue in each market with the help of matrix algebra.
(b) If the unit costs of the above three commodities are Rs. 2.00, Rs. 1.00 and 50 paise respectively. Find the gross profit.
View SolutionA manufacturer produces three products x, y, z which he sells in two markets. Annual sales are indicated below:
MarketProductsI10,0002,00018,000II6,00020,0008,000
(a) If unit sale prices of x, y and z are Rs. 2.50, Rs. 1.50 and Rs. 1.00, respectively, find the total revenue in each market with the help of matrix algebra.
(b) If the unit costs of the above three commodities are Rs. 2.00, Rs. 1.00 and 50 paise respectively. Find the gross profit.
View SolutionA manufacturer produces three products x, y, z which he sells in two markets. Annual sales are indicated below:
Market Products
I 10.000 2.000 18.000
II 6.000 20.000 8.000
(a) If unit sale prices of x, y and z are Rs 2.50, Rs 1.50 and Rs 1.00, respectively, find the total revenue in each market with the help of matrix algebra.(b) If the unit costs of the above three commodities are Rs 2.00, Rs 1.00 and 50paise respectively. Find the gross profit.
View SolutionA manufacture produces three products x,y,z which he sells in two markets . Annual sales are indicated below :
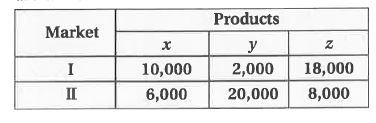
(a) If unit sale prices of x,yand z are Rs. 2.50, Rs. 1.50 and Rs. 1.00 , respectively, find the total revenue in each market with the help of matrix algebra.
(b) If the unit costs of the above three commodities are Rs. 2.00, Rs. 1.00 and 50 paise repectively .Find the gross profit.
View SolutionA manufacturer produces three products A,B and C which he sells in market I and II. The annual sales are as shown below.(I) If the unit sale prices of A,B,C are Rs. 3, Rs. 2 and Rs. 4 respectively in market I and they are Rs. 4, Rs. 3 and Rs. 6 in market II. Find the total revenue in each market using matrix algebra.
View SolutionA manufacturer produces three products A,B and C which he sells in market I and II. The annual sales are as shown below.(I) If the unit sale prices of A,B,C are Rs. 3, Rs. 2 and Rs. 4 respectively in market I and they are Rs. 4, Rs. 3 and Rs. 6 in market II. If the unit prices of A,B,C are Rs. 2.50, Rs. 1.50 and Rs. 3 respectively, then find the gross profit, using matrix algebra.
View SolutionA manufacture produces three stationery products Pencil, Eraser and Sharpener which he sells in two markets. Annual sales are indicated below

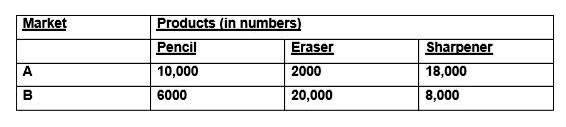
If the unit Sale price of Pencil, Eraser and Sharpener are Rs. 2.50, Rs. 1.50 and Rs. 1.00 respectively, and unit cost of the above three commodities are Rs. 2.00, Rs. 1.00 and Rs. 0.50 respectively, then,
Based on the above information answer the following:
Total revenue of market A
View SolutionA manufacture produces three stationery products Pencil, Eraser and Sharpener which he sells in two markets. Annual sales are indicated below

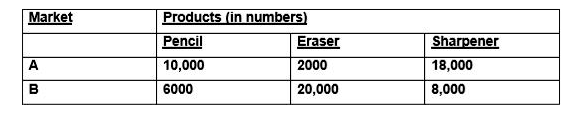
If the unit Sale price of Pencil, Eraser and Sharpener are Rs. 2.50, Rs. 1.50 and Rs. 1.00 respectively, and unit cost of the above three commodities are Rs. 2.00, Rs. 1.00 and Rs. 0.50 respectively, then,
Based on the above information answer the following:
Total revenue of market B
View Solution
