Question

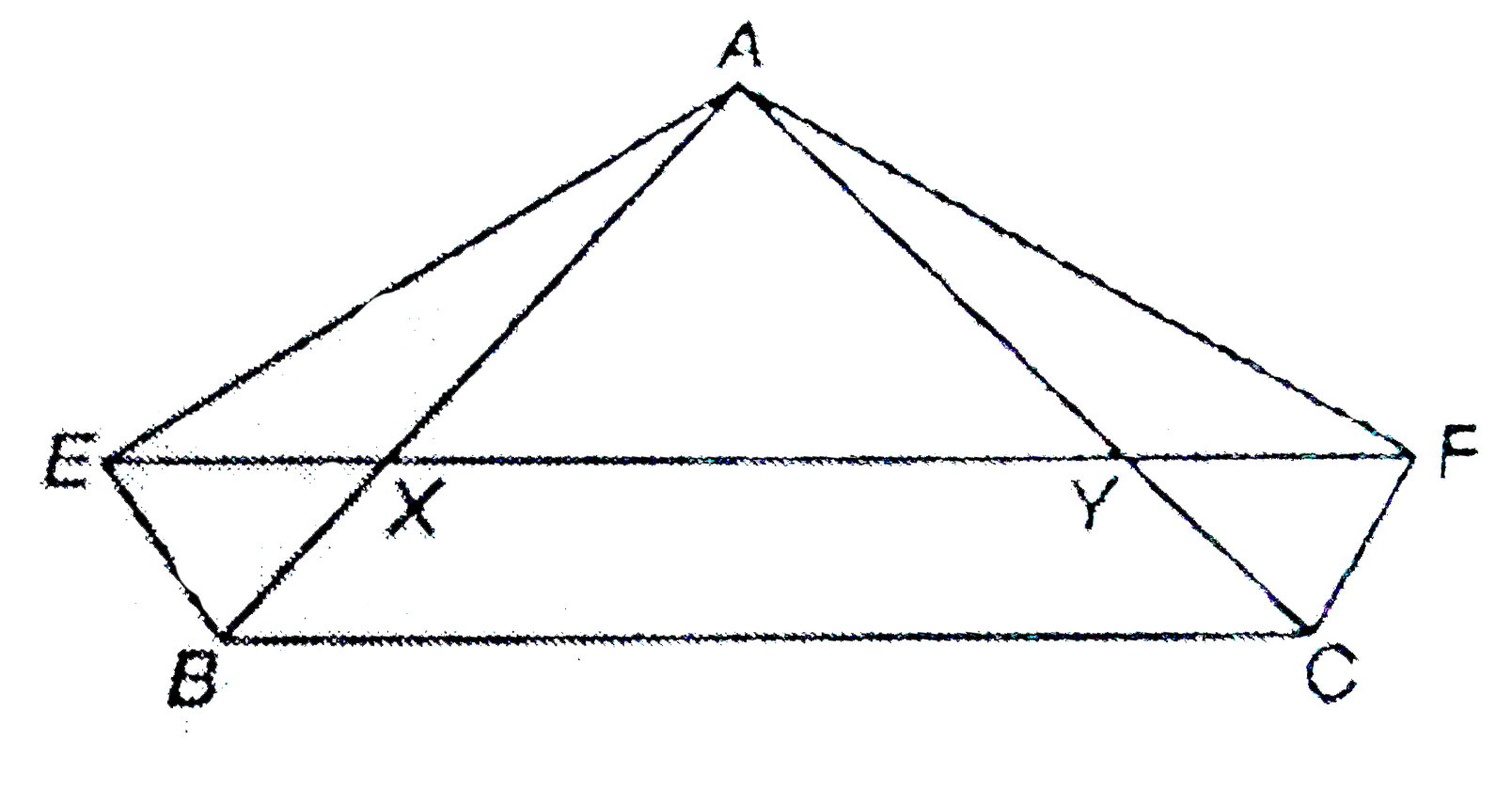
$XY$ is a line parallel to side $BC$ of a triangle $ABC$. If $BE\mid \mid AC$ and $CF\mid \mid AB$ meet $XY$ at $E$ and $F$ respectively, show that $ar\left(ABE\right)=ar\left(ACF\right)$

Explanation

Since, `BE||AC rArr BE ||YC`...(1)
and `BC || EY` (given)...(2)
`:. squareBCYE` is a parallelogram (`:.` pairs of opposite sides are parallel)
Now, since `DeltaABE` and parallelogram BCYE are on the same
base EB and between the same parallels EB and AC,
`:. ar(DeltaABE) = (1)/(2) ar(||gm BCYE)`...(3)
Also, since `CF ||AB rArr CF ||BX`
and `BC||XF` (given)
`:. square BCFX` is a paralleogram (`:.` pairs of opposite sides are parallel)
Now, since `DeltaACF` and parallelogram BCFX are on the same base CF and between the same parallels CF and AB,
`:. ar(DeltaACF) = (1)/(2) ar(||gm BCFX)`....(4)
But `ar(||gm BCYE) = ar(||gm BCFX)`...(5)
(`:.` parallelograms are on the same base BC and between same parallels BC and EF)
`:.` From (3), (4) and (5), we have
`ar(DeltaABE) = ar(DeltaACF)`