Find the standard deviation of 8,11,14,17,20,23,26.
To find the standard deviation of the given data set 8,11,14,17,20,23,26, we will follow these steps:
Step 1: Calculate the Mean (¯x)
The mean is calculated using the formula:
¯x=∑xin
where ∑xi is the sum of all observations and n is the number of observations.
Calculating the sum of observations:
∑xi=8+11+14+17+20+23+26=119
Number of observations:
n=7
Calculating the mean:
¯x=1197=17
Step 2: Calculate the Deviations from the Mean
Next, we calculate the deviations of each observation from the mean (xi−¯x).
- For x1=8: 8−17=−9
- For x2=11: 11−17=−6
- For x3=14: 14−17=−3
- For x4=17: 17−17=0
- For x5=20: 20−17=3
- For x6=23: 23−17=6
- For x7=26: 26−17=9
Step 3: Calculate the Squared Deviations
Now, we square each of the deviations calculated in the previous step.
- For −9: (−9)2=81
- For −6: (−6)2=36
- For −3: (−3)2=9
- For 0: (0)2=0
- For 3: (3)2=9
- For 6: (6)2=36
- For 9: (9)2=81
Step 4: Sum of Squared Deviations
Now, we sum the squared deviations:
∑(xi−¯x)2=81+36+9+0+9+36+81=252
Step 5: Calculate the Standard Deviation
Finally, we use the formula for standard deviation:
σ=√∑(xi−¯x)2n
Substituting the values we calculated:
σ=√2527=√36=6
Conclusion
The standard deviation of the data set 8,11,14,17,20,23,26 is 6.
---
The mean is calculated using the formula:
¯x=∑xin
where ∑xi is the sum of all observations and n is the number of observations.
∑xi=8+11+14+17+20+23+26=119
n=7
¯x=1197=17
Next, we calculate the deviations of each observation from the mean (xi−¯x).
- For x2=11: 11−17=−6
- For x3=14: 14−17=−3
- For x4=17: 17−17=0
- For x5=20: 20−17=3
- For x6=23: 23−17=6
- For x7=26: 26−17=9
Now, we square each of the deviations calculated in the previous step.
- For −6: (−6)2=36
- For −3: (−3)2=9
- For 0: (0)2=0
- For 3: (3)2=9
- For 6: (6)2=36
- For 9: (9)2=81
Now, we sum the squared deviations:
∑(xi−¯x)2=81+36+9+0+9+36+81=252
Finally, we use the formula for standard deviation:
σ=√∑(xi−¯x)2n
Substituting the values we calculated:
σ=√2527=√36=6
The standard deviation of the data set 8,11,14,17,20,23,26 is 6.
Topper's Solved these Questions
- STATISTICSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - EXERCISE
20 Videos
- STATISTICSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - NCERT QUESTIONS
27 Videos
- SETSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SETSEXERCISE - MISC Exercise
16 Videos
- STRAIGHT LINESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Exercise
207 Videos
Similar Questions
Standard deviation of 5,8,11,9,11 is
View SolutionIf the standard deviation of 0,1,2,3...9 is K, then the standard deviation of 10,11,12,13....19 is
View SolutionIf standard deviation of 1,2,3,4,….,10 is σ then standard deviation of 11, 12, …20 is
View SolutionThe standard deviation of 26, 27, 31, 32, 35 is
View SolutionIf standard deviation of 1,2,3,4….,10 is σ then, show that standard deviation of 11, 12,…. , 20 is σ
View SolutionFind the standard deviation for the following data

View SolutionFind the standard deviation for the following data
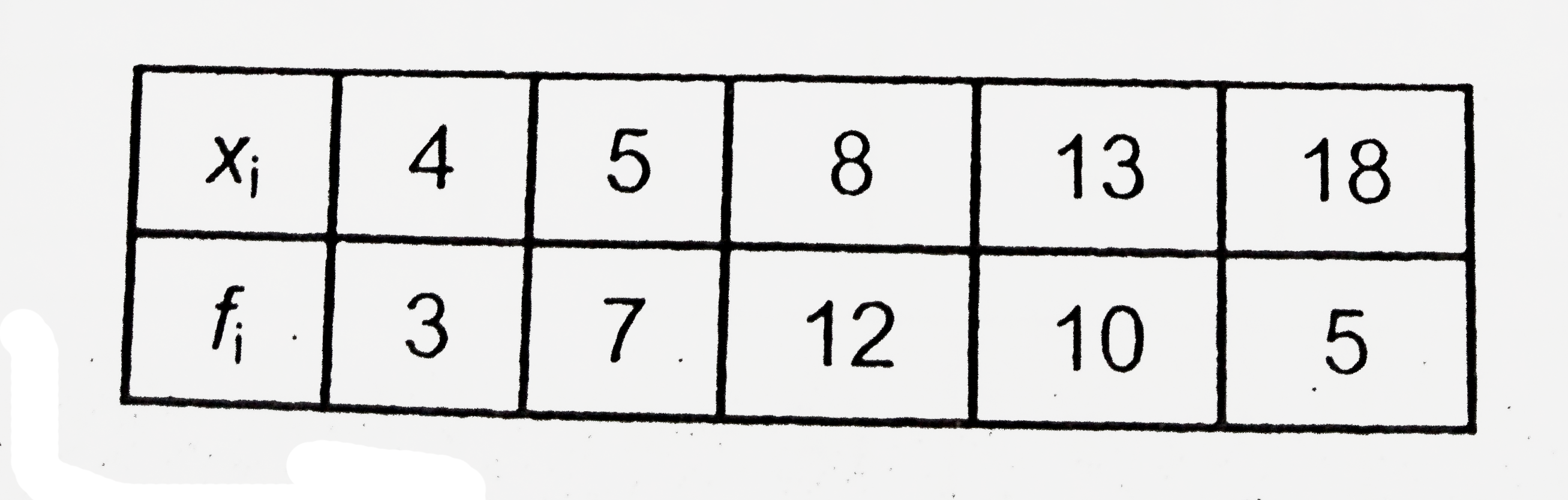
View SolutionThe following table gives the number of finished articles turned out per day be different number of workers in a factors. Find the standard deviation of the daily output of finished articles. Find the variance and standard deviation of the following frequency distribution: Number of article:, 18, 19, 20, 21, 22, 23, 24, 25, 26, 27 No. of workers:, 3, 7, 11, 14, 18, 17, 13, 8, 5, 4
View SolutionThe following table gives the number of finished articles turned out per day be different number of workers in a factors. Find the standard deviation of the daily output of finished articles. Find the variance and standard deviation of the following frequency distribution: Number of article:, 18, 19, 20, 21, 22, 23, 24, 25, 26, 27 No. of workers:, 3, 7, 11, 14, 18, 17, 13, 8, 5, 4
View SolutionFind the standard deviation from the following observations:
15,18,13,20,17,10,16,19,22,20.
View Solution
