Prove by vector method that the line segment joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides and equal to half of their difference.
Let □ ABCD is a trapezium in which AB||DC.PandQ are the mid-points of the diagonals AC and BD respectively. Join DP and produced upto R.
∠1=∠2
(alternate interior angles,AB||DC...(1)
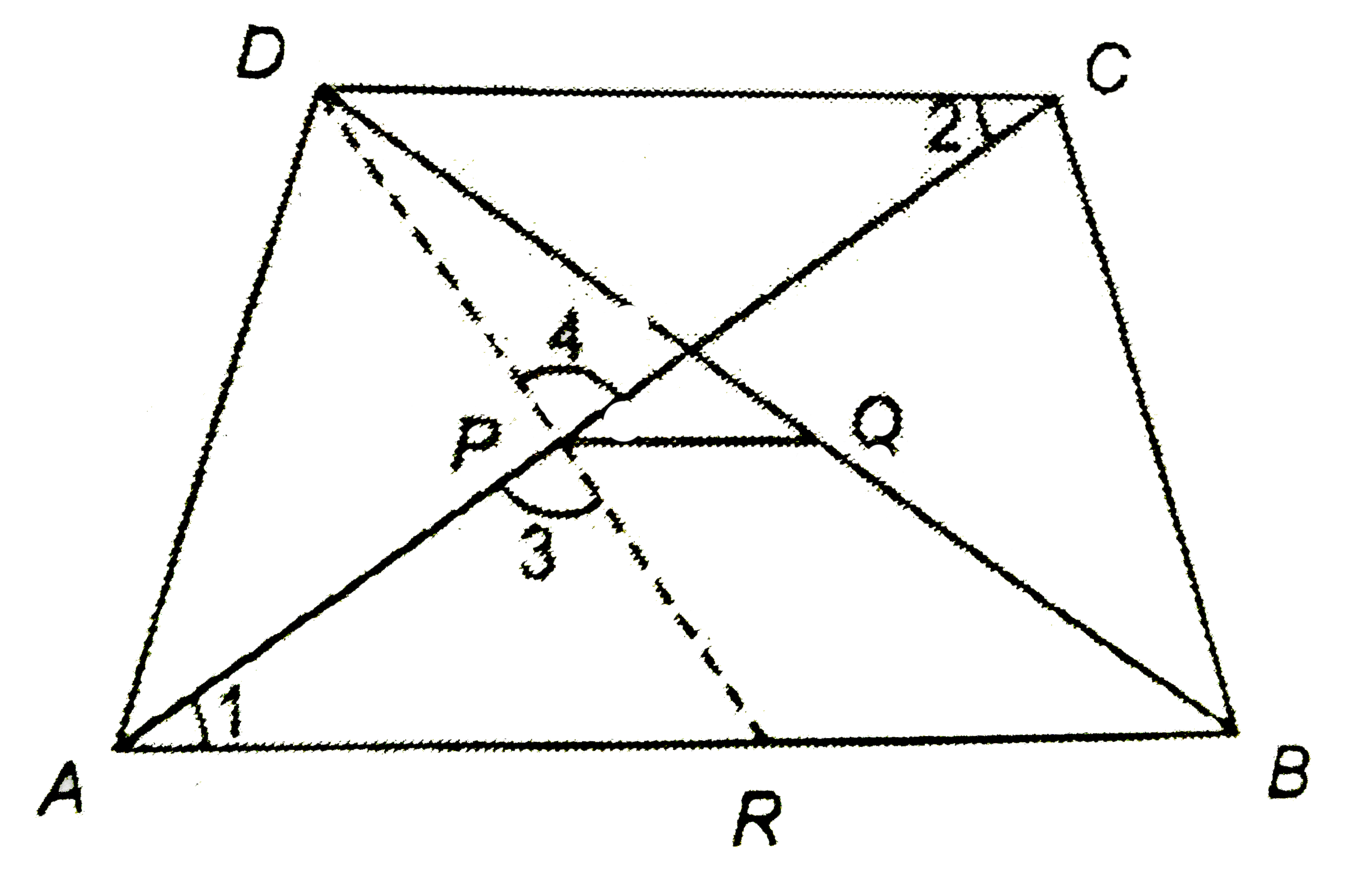
InΔAPRandΔDPC,
∵⎧⎪⎨⎪⎩∠1=∠2[from(1)]∠3=∠4(vertically opposite angles)AP=CP(given)
∴ ΔAPR≅ΔCPD (ASA theorem)
⇒ AR=CDand PR=DR (c.p.c.t.)...(2)
∴ P is the mid-point of DR.
Now, in ΔDRB,
Since P and Q are the mid-points of DR and DB respectively,
∴ PQ||RBandPQ=12RB (mid-point theorem)
⇒ PQ||ABandPQ=12(AB−AR)
⇒ PQ||AB||CDandPQ=12(AB−CD) [from(2)]
Topper's Solved these Questions
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Problems From NCERT/exemplar
11 Videos
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Exercise 8a
29 Videos
- PROBABILITYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - PROBABILITYEXERCISE - Revision Exercise (very Short Answer /short Answer Questions)
10 Videos
- STATISTICSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - Revision Exercise
12 Videos
Similar Questions
Prove that the line segment joining the mid-points of the diagonals of a trapezium is parallel to each of the parallel sides and is equal to half the difference of these sides.
View SolutionProve that the line segment joining the mid-points of the diagonals of a trapezium is parallel to each of the parallel sides and is equal to half the difference of these sides.
View SolutionProve that the line joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides of trapezium and is half of their difference.
View SolutionProve that the segment joining the middle points of two non-parallel sides of a trapezium is parallel to the parallel sides and half of their sum.
View SolutionProve that the line segment joining the mid points of two side of a triangle is parallel to the third side and equal to half of it.
View SolutionProve that the line segment joining the mid points of two side of a triangle is parallel to the third side and equal to half of it.
View SolutionThe line segment joining the mid-points of any two sides of a triangle in parallel to the third side and equal to half of it.
View SolutionProve that the line joining the mid-points of the two sides of a triangle is parallel to the third side.
View SolutionShow that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other
View SolutionShow that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other.
View Solution
