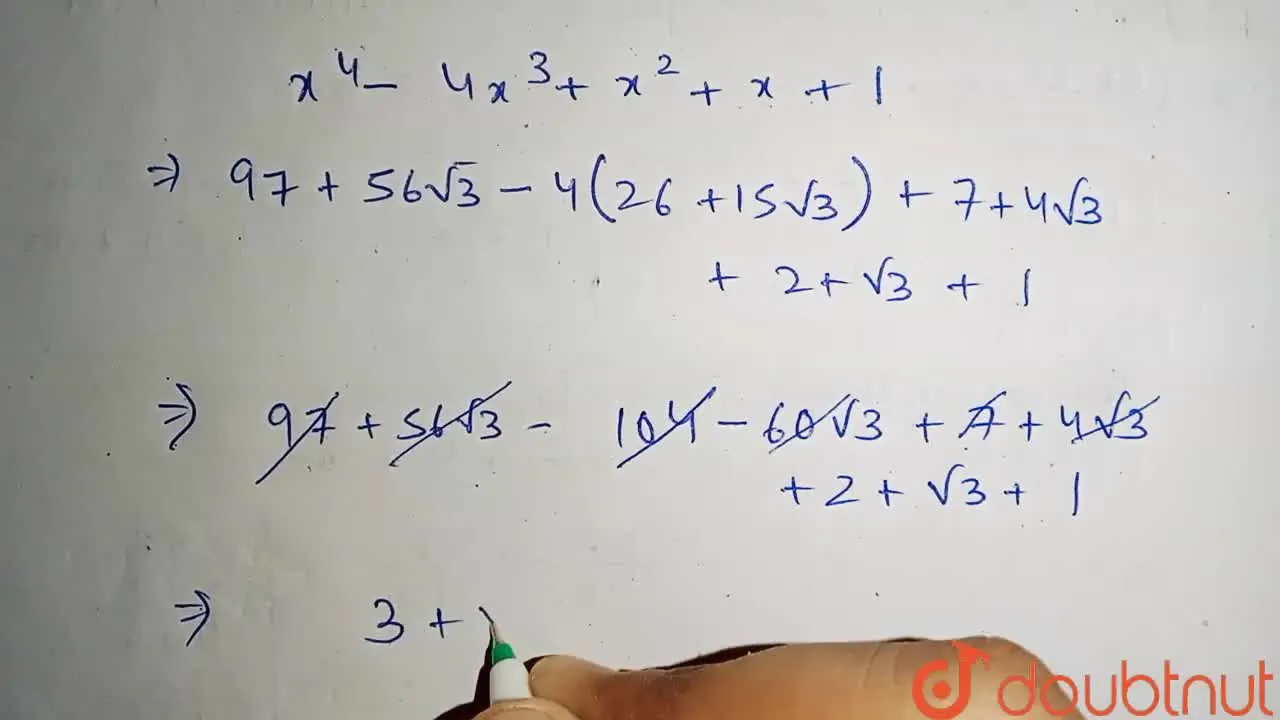If x=2+√3, then find the value of x4−4x3+x2+x+1.
Video Solution
Text Solution
The correct Answer is:3+√3
N/a
|
Updated on:21/07/2023
Related Playlists
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - NUMBER SYSTEMEXERCISE - Revision Exercise (very Shortanswer Questions)10videos
NUMBER SYSTEM
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - NUMBER SYSTEMEXERCISE - Revision Exercise (short Answer Questions)10videos
NUMBER SYSTEM
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - NUMBER SYSTEMEXERCISE - Exercise 1d7videos
NUMBER SYSTEM
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINES AND ANGLESEXERCISE - Revision Exercise (long Answer Questions )6videos
LINES AND ANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - POLYNOMIALSEXERCISE - Revision Exercise (short Answer Questions)16videos
POLYNOMIALS
Similar Questions
If x=2+ √3, find the value of x+1x
View SolutionIf x=√2−1 then find the value of (1x−x)3:-
View SolutionIf x = √3−√2 find the value of
x+1xView SolutionIf x=1−√2 , find the value of (x−1x)3
View SolutionIf x=12−√3, find the value of x3−2x2−7x+5
View SolutionIf x=12−√3, find the value of x3−2x2−7x+5
View SolutionIf x=12−√3, find the value of x3−2x2−7x+5
View SolutionIf x=1−√2,f∈dthevalueof(x−1x)3
View SolutionIf x=−5+√−16, find the value of x4+9x3+35x2−x+4
View SolutionIf x−1x=3+2√2 , find the value of x3−1x3
View Solution