The locus of the point of intersection of tangents to the ellipse x2a2+y2b2=1 at the points whose eccentric angles differ by π/2, is
x2+y2=a2
x2+y2=b2
x2+y2=a2+b2
x2a2+y2b2=2
The correct Answer is:D
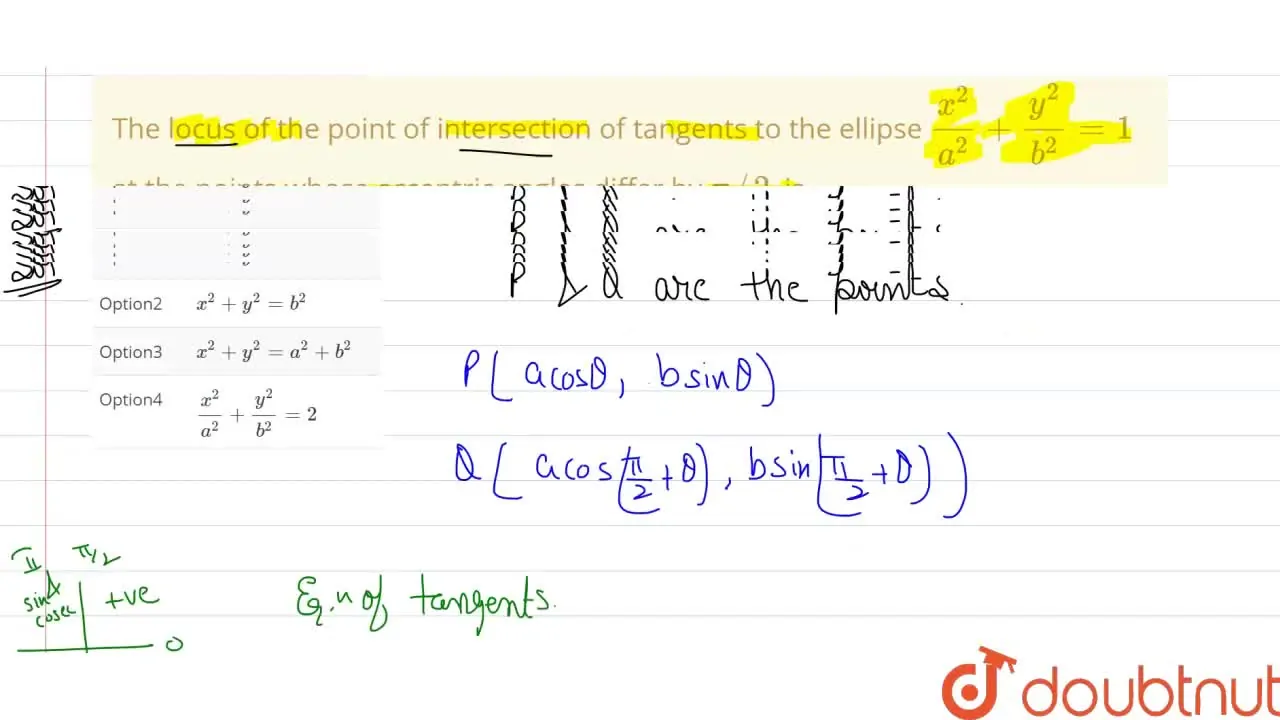
Let P(acosθ,bsinθ) and
Q(acos(π/2+θ),bsin(π/2+θ)) be two points on the ellipse. The equations of tangents to the ellipse at points P and Q are xacosθ+ybsinθ=1
and , −xasinθ+ybcosθ=1
Let P (h, k) be the point of intersection of (i) and (ii). Then,
hacosθ+kbsinθ=1and−hasinθ+kbcosθ=1
⇒(hacosθ+kbsinθ)2+(−hasinθ+kbcosθ)2=2
⇒h2a2+k2b2=2
Hence, the locus of (h, k) is x2a2+y2b2=2.
ALITER–––––––––– The coordinates of the point of intersection of tangents at points having eccentric angles aαandβ are
⎛⎜
⎜⎝acos(α+β2)cos(α+β2),bsin(α+β2)cos(α+β2)⎞⎟
⎟⎠
Thus, if (h, k) is the point of intersection of tangents at points whose eccentric angles angles differ by π2 i.e. α−β=π/2, then
h=√2acos(α+β2)andk=√2bsin(α+β2)
⇒h22a2+k22b2=1
Hence, the locus of (h, k) is x22a2+y22b2=1.
Related Playlists
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ELLIPSEEXERCISE - Section II - Assertion Reason Type7videos
ELLIPSE
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ELLIPSEEXERCISE - Exercise80videos
ELLIPSE
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ELLIPSEEXERCISE - Chapter Test29videos
ELLIPSE
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DIFFERENTIATIONEXERCISE - Chapter Test30videos
DIFFERENTIATION
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - EXPONENTIAL AND LOGARITHMIC SERIESEXERCISE - Chapter Test20videos
EXPONENTIAL AND LOGARITHMIC SERIES
Similar Questions
The locus of the point of intersection of tangents to the ellipse x2a2+y2b2=1 which meet at right , is
View SolutionThe locus of the point of intersection of tangents to the ellipse x2a2+y2b2=1, which make complementary angles with x - axis, is
View SolutionThe locus of point of intersection of tangents to an ellipse x2a2+y2b2=1 at two points the sum of whose eccentric angles is constant is
View SolutionThe locus of point of intersection of tangents to an ellipse x2a2+y2b2=1 at two points the sum of whose eccentric angles is constant is
View SolutionFind the locus of the points of the intersection of tangents to ellipse x2a2+y2b2=1 which make an angle theta.
View SolutionThe locus of the point of intersection of two prependicular tangents of the ellipse x29+y24=1 is
View SolutionThe locus of the point of intersection of the perpendicular tangents to the ellipse 2x2+3y2=6 is
View SolutionThe locus of the point of intersection of the tangents to the circle x2+y2=a2 at points whose parametric angles differ by π3.
View SolutionThe locus of the point of intersection of the tangents to the circle x=acosθ,y=asinθ at the points, whose parametric angles differ by π3 is
View SolutionFind the locus of the point of intersection of tangents to the circle x=acosθ,y=asinθ at the points whose parametric angles differ by (i)π3,
View Solution