If 4sin−1x+cos−1x=π, then x is equal to
A
12
B
√32
C
−12
D
none of these
Video Solution
Text Solution
The correct Answer is:A
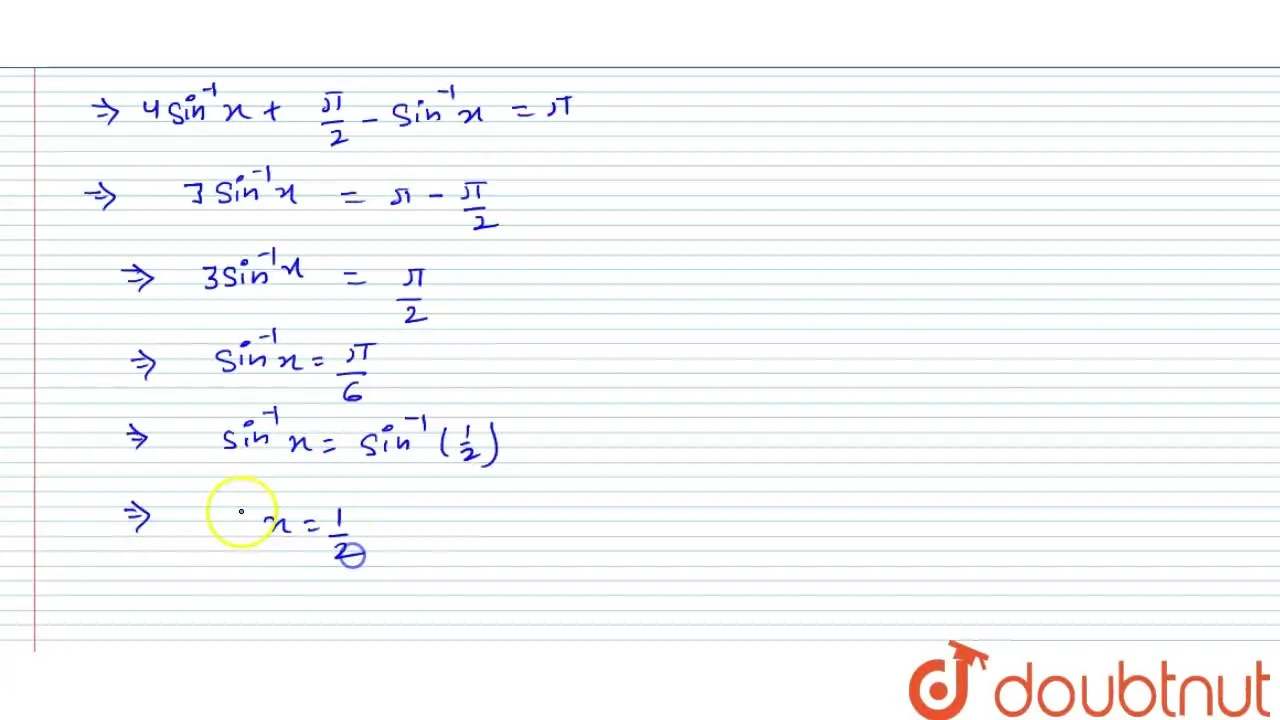
We have
4sin−1x+cos−1x=π
→4sin−1x+π2−sin−1x=π
→3sin−1x=π2→x=sin(π)6=12
|
Updated on:21/07/2023
Related Playlists
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONS EXERCISE - Section I - Solved Mcqs71videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONS EXERCISE - Section II - Assertion Reason Type11videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - INDEFINITE INTEGRALSEXERCISE - Chapter Test30videos
INDEFINITE INTEGRALS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - LIMITSEXERCISE - Chapter Test58videos
LIMITS
Similar Questions
If ∣∣sin−1x∣∣+∣∣cos−1x∣∣=π2, then x∈
View SolutionIf 4 sin−1x+cos−1x=π , then what is the value of x ?
View SolutionLet f(x)=sin−1x+cos−1x. Then π2 is equal to
View SolutionIf sin−1(1−x)−2sin−1x=π2, then x is equal to
View SolutionSolve : 4sin−1x=π−cos−1x
View SolutionSolve : 4sin−1x=π−cos−1x
View Solutionif tan−1(1x)+cos−1(2√5)=π4 then x equals
View SolutionThe soluation set of inequality (sinx+cos−1x)−(cosx−sin−1x)≥π2, is equal to
View Solution∫π−πsin4xsin2x+cos4xdx is equal to
View SolutionIf sin−1x+sin−1y=π3 and cos−1x−cos−1y=π6 , find the values of x and y .
View Solution