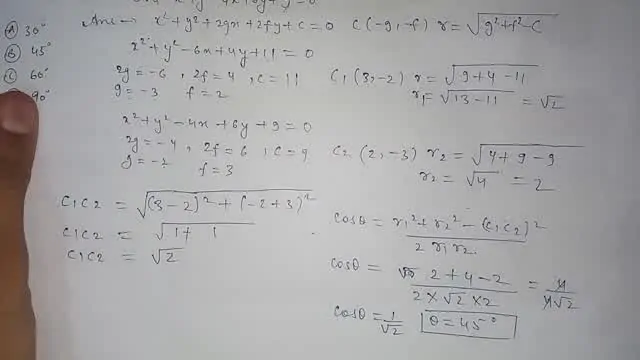Find the angle of intersection of the circles
The correct Answer is:B
The equations of the circles are
...(i)
...(ii)
The centres of these circles are respectively. Let be their radii. Then,
.
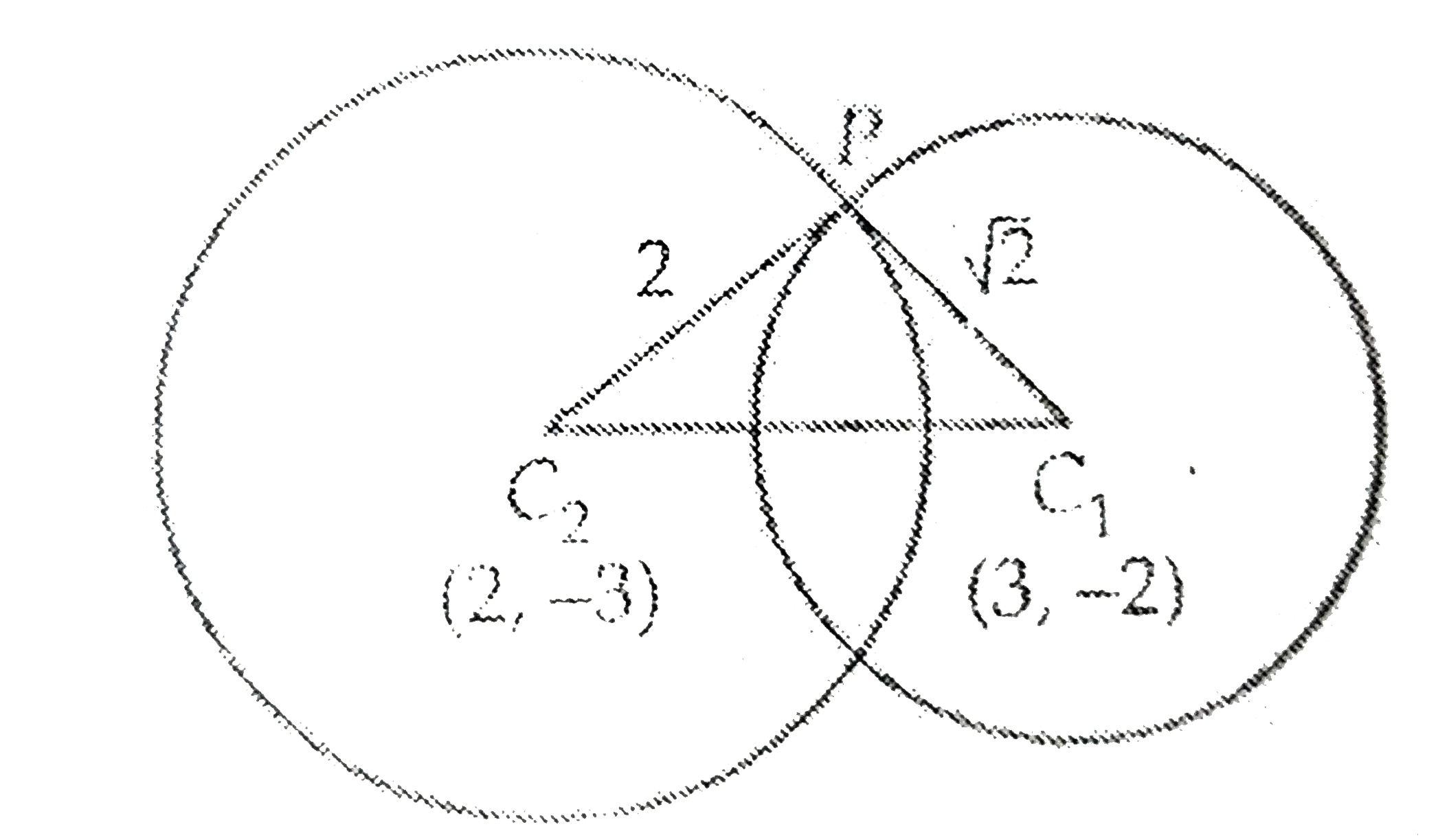
Also,
Suppose circles (i) and (ii) intersect at P. Then,
Thus, the angle of intersection of the given circles is of .
Related Playlists
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CIRCLESEXERCISE - Section I - Solved Mcqs108videos
CIRCLES
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CIRCLESEXERCISE - Section-I (Solved MCQs)1videos
CIRCLES
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test30videos
CARTESIAN PRODUCT OF SETS AND RELATIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test58videos
COMPLEX NUMBERS
Similar Questions
Find the angle of intersection of curve x2+y2−4x−1=0 and x2+y2−2y−9=0
View SolutionThe equation of the circle having its centre on the line x+2y−3=0 and passing through the points of intersection of the circles x2+y2−2x−4y+1=0andx2+y2−4x−2y+4=0 is x2+y2−6x+7=0 x2+y2−3y+4=0 c.x2+y2−2x−2y+1=0 x2+y2+2x−4y+4=0
View SolutionTangents drawn from P(1,8) to the circle x2+y2−6x−4y−11=0 touches the circle at the points A and B, respectively. The radius of the circle which passes through the points of intersection of circles x2+y2−2x−6y+6=0andx2+y2−2x−6y+6=0 the circumcircle of the and interse ΔPAB orthogonally is equal to
View SolutionFind the equation of the circle passing through the point of intersection of the circles x2+y2−6x+2y+4=0,x2+y2+2x−4y−6=0 and with its centre on the line y=x.
View SolutionThe circles x2+y2+6x+6y=0 and x2+y2−12x−12y=0
View SolutionFind the point of intersection of the circle x2+y2−3x−4y+2=0 with the x-axis.
View SolutionFind the equation of the circle passing through the points of intersections of circles x2+y2+6x+4y−12=0,x2+y2−4x−6y−12=0, and having radius √13.
View SolutionFind the equation of the circle which passes through the points of intersection of circles x2+y2−2x−6y+6=0andx2+y2+2x–6y+6=0 and intersects the circle x2+y2+4x+6y+4=0 orthogonally.
View SolutionFind the length of the common chord of the circles x2+y2+2x+6y=0 and x2+y2−4x−2y−6=0
View SolutionFind the angle between the circles S:x2+y2−4x+6y+11=0andS':x2+y2−2x+8y+13=0
View Solution