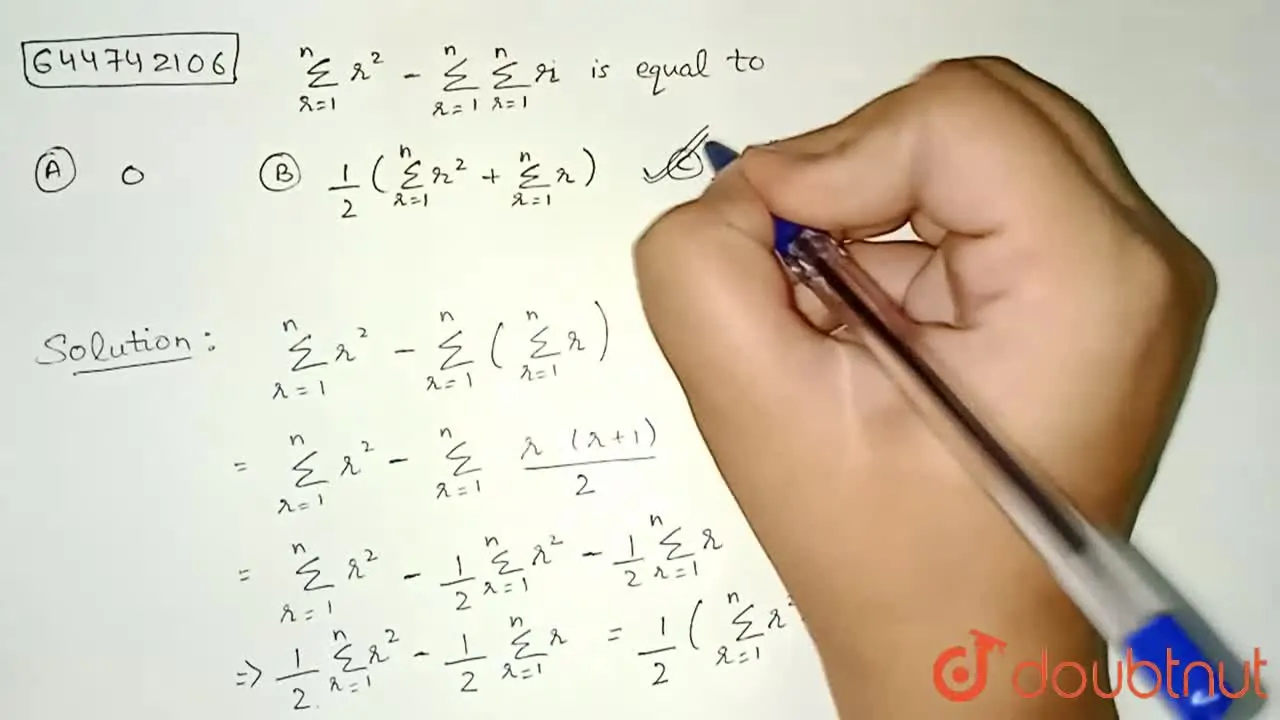
n∑r=1r2−n∑r=1n∑r=1 is equal to
A
0
B
12(n∑r=1r2+n∑r=1r)
C
12{n∑r=1r2−n∑r=1r}
D
none of these
Video Solution
Text Solution
The correct Answer is:C
We have,
n∑r=1r2−n∑r=1m∑r=1r
=n∑r=1r2−n∑m=1m(m+1)2
=n∑r=1r2−12n∑m=1m2−12n∑m=1m
12n∑r=1r2−12n∑r=1r=12{n∑r=1r2−n∑r=1r}
|
Updated on:21/07/2023
Related Playlists
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Section II - Assertion Reason Type13videos
SEQUENCES AND SERIES
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise129videos
SEQUENCES AND SERIES
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Chapter Test59videos
SEQUENCES AND SERIES
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - QUADRATIC EXPRESSIONS AND EQUATIONS EXERCISE - Chapter Test50videos
QUADRATIC EXPRESSIONS AND EQUATIONS
- BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SETSEXERCISE - Chapter Test30videos
SETS
Similar Questions
n∑r=1 r(n-r) is equal to :
View Solution∑nr=1r4∑nr=1r2 is equal to
View Solutionn∑r=11log2r4 is equal to
View Solutionn∑r=1r(n−r+1) is equal to :
View Solutionlimx→2∑nr=1xr−∑nr=12rx−2 is equal to
View SolutionThe value of n∑r=1(−1)r+1nCrr+1 is equal to
View SolutionThe value of 10∑r=1r.nCrnCr−1 is equal to
View SolutionLet n∑r=1r6=f(n), then n∑n=1(2r−1)6 is equal to
View SolutionIf n∑r=1r√103n∑r=1r2,n∑r=1r3 are in G.P., then the value of n, is
View SolutionThe sum of the series n∑r=1(−1)r−1.nCr(a−r),n>1 is equal to :
View Solution