A non-conducting spherical shell of radius R surrounds a point charge q (q at center). The electric flux through a cap of the shell of half angle θ is:
2πqθ∈0
q2∈0(1−cosθ)
q(2θ)4π∈0
qθ2π∈0
The correct Answer is:B
To solve the problem of finding the electric flux through a cap of a non-conducting spherical shell of radius R surrounding a point charge q at the center, we can follow these steps:
1. Understand the Geometry:
- We have a spherical shell of radius R with a point charge q at its center. We need to find the electric flux through a cap of the shell that subtends a half-angle θ at the center.
2. Electric Field Due to Point Charge:
- The electric field E due to a point charge q at a distance r from the charge is given by:
E=kqr2
- Here, k is Coulomb's constant, and for our case, r=R (the radius of the shell).
3. Determine the Area Element:
- Consider a small area element dA on the cap of the sphere. The area of a small ring at an angle ϕ from the vertical axis can be expressed as:
dA=R2sinϕdϕdθ
- The total area of the cap can be integrated from ϕ=0 to ϕ=θ.
4. Calculate the Electric Flux:
- The electric flux Φ through the cap is given by:
Φ=∫E⋅dA
- Since E is constant over the cap and directed outward, we can simplify this to:
Φ=E∫dA
- Substitute E=kqR2:
Φ=kqR2∫θ0R2sinϕdϕdθ
5. Integrate the Area:
- The integral of sinϕ from 0 to θ is:
∫θ0sinϕdϕ=−cosϕ∣∣∣θ0=1−cosθ
- Therefore, the total flux becomes:
Φ=kqR2R2(1−cosθ)=kq(1−cosθ)
6. Final Expression:
- Using k=14πϵ0, we can write the flux as:
Φ=q4πϵ0(1−cosθ)
Conclusion:
The electric flux through the cap of the spherical shell is:
Φ=q4πϵ0(1−cosθ)
- We have a spherical shell of radius R with a point charge q at its center. We need to find the electric flux through a cap of the shell that subtends a half-angle θ at the center.
- The electric field E due to a point charge q at a distance r from the charge is given by:
E=kqr2
- Here, k is Coulomb's constant, and for our case, r=R (the radius of the shell).
- Consider a small area element dA on the cap of the sphere. The area of a small ring at an angle ϕ from the vertical axis can be expressed as:
dA=R2sinϕdϕdθ
- The total area of the cap can be integrated from ϕ=0 to ϕ=θ.
- The electric flux Φ through the cap is given by:
Φ=∫E⋅dA
- Since E is constant over the cap and directed outward, we can simplify this to:
Φ=E∫dA
- Substitute E=kqR2:
Φ=kqR2∫θ0R2sinϕdϕdθ
- The integral of sinϕ from 0 to θ is:
∫θ0sinϕdϕ=−cosϕ∣∣∣θ0=1−cosθ
- Therefore, the total flux becomes:
Φ=kqR2R2(1−cosθ)=kq(1−cosθ)
- Using k=14πϵ0, we can write the flux as:
Φ=q4πϵ0(1−cosθ)
The electric flux through the cap of the spherical shell is:
Φ=q4πϵ0(1−cosθ)
Topper's Solved these Questions
Similar Questions
A point charge q is placed at a point on the axis of a non-conducting circular plate of radius r at a distance R(>>r) from its center. The electric flux associated with the plate is
View SolutionA point charge Q is placed inside a conducting spherical shell of inner radius 3R and outer radius 5R at a distance R from the centre of the shell. The electric potential at the centre of the shell will be
View SolutionA uniformly charged non-conducting spherical shell is given +q charge and a point charge -q is placed as shown. The electric field lines will be

View SolutionA conducting shell of radius R carries charge −Q. A point charge +Q is placed at the centre. The electric field E varies with distance r (from the centre of the shell) as
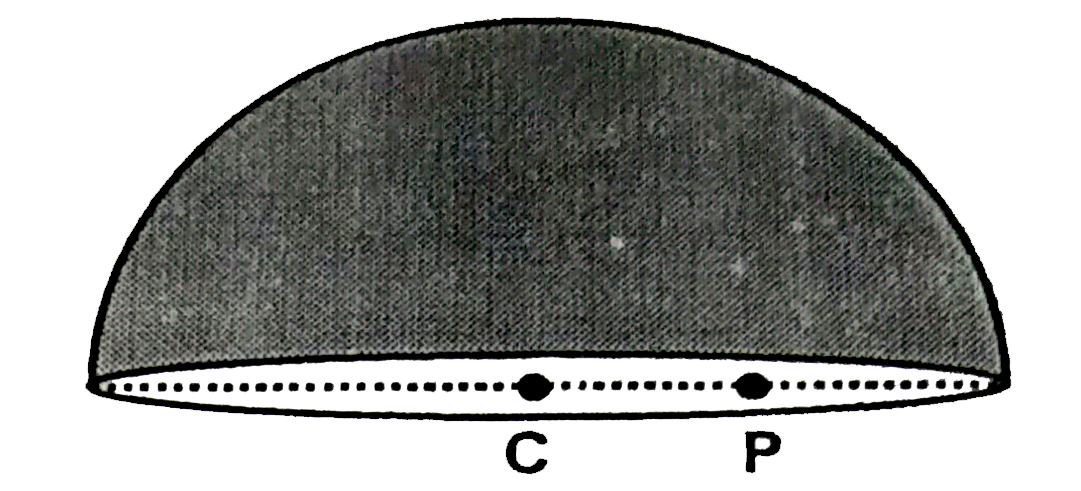
View SolutionA thin non-conducting hemispherical shell contains a positive charge q on it, which uniformly distributed on the shell. A point P lies on the diameter of shell as show in figure. Then the direction of electric field at the point 'P' is
View SolutionA conducting shell of radius R carries charge–Q. A point charge +Q is placed at the centre of shell. The electric field E varies with distance r (from the centre of the shell) as :
View SolutionA point charge q is placed inside a conducting spherical shell of inner radius 2R and outer radius 3R at a distance of R fro the centre of the shell. The electric potential at the centre of shell will (potential at infinity is zero).
View SolutionA point charge +q is placed at the centre of a conducting spherical shell of inner radius a and outer radius b. Find the charge appearing on the inner and outer surface of the shell.
View SolutionA spherical shell of radius R carries a uniformly distributed charge q. The electrical forces arising cause the ex-pansion of the shell. Find the mechanical stress in the shell.
View SolutionThere are two concentric hollow conducting spherical shells of radii r and R(R>r). The charge on the outer shell is Q. What charge should be given to the inner shell, so that the potential at a point P , at a distance 2R from the common centre is zero ?
View Solution
