A loop ABCA of straight edges has three corner points A (8, 0, 0), B (0, 8, 0 ) and C (0, 0, 8). The magnetic field in this region is →B=5(ˆi+ˆj+ˆk)T0 . The quantity of flux through the loop ABCA (in Wb) is _____.
The correct Answer is:480
To find the magnetic flux through the loop ABCA, we can follow these steps:
Step 1: Identify the coordinates of points A, B, and C
- Point A: A(8,0,0)
- Point B: B(0,8,0)
- Point C: C(0,0,8)
Step 2: Determine the area of the triangle ABC
The area A of triangle ABC can be calculated using the formula for the area of a triangle given its vertices:
A=12×base×height
In this case, we can use the lengths of the sides of the triangle. The lengths of the sides can be calculated using the distance formula:
- Length AB = √(8−0)2+(0−8)2=√64+64=8√2
- Length BC = √(0−0)2+(8−0)2+(0−8)2=8√2
- Length AC = √(8−0)2+(0−0)2+(0−8)2=8√2
Since triangle ABC is equilateral, we can use the formula for the area of an equilateral triangle:
A=√34s2
where s is the length of a side. Here, s=8√2:
A=√34(8√2)2=√34×128=32√3
Step 3: Calculate the magnetic flux
The magnetic flux Φ through the loop is given by:
Φ=→B⋅→A
where →B=5(^i+^j+^k) T and →A is the area vector of the triangle ABC. The area vector is perpendicular to the plane of the triangle and has a magnitude equal to the area of the triangle.
Assuming the area vector points in the direction of ^i+^j+^k:
→A=A^n=32√3^n
where ^n is the unit vector in the direction of ^i+^j+^k. The magnitude of ^n is:
|^n|=√12+12+12=√3
Thus, the unit vector is:
^n=1√3(^i+^j+^k)
Therefore, the area vector becomes:
→A=32√3⋅1√3(^i+^j+^k)=32(^i+^j+^k)
Step 4: Calculate the dot product
Now we can calculate the dot product:
Φ=→B⋅→A=5(^i+^j+^k)⋅32(^i+^j+^k)=5⋅32⋅(1+1+1)=5⋅32⋅3=480Wb
Final Answer
The quantity of flux through the loop ABCA is Φ=480Wb.
---
- Point A: A(8,0,0)
- Point B: B(0,8,0)
- Point C: C(0,0,8)
The area A of triangle ABC can be calculated using the formula for the area of a triangle given its vertices:
A=12×base×height
In this case, we can use the lengths of the sides of the triangle. The lengths of the sides can be calculated using the distance formula:
- Length AB = √(8−0)2+(0−8)2=√64+64=8√2
- Length BC = √(0−0)2+(8−0)2+(0−8)2=8√2
- Length AC = √(8−0)2+(0−0)2+(0−8)2=8√2
A=√34s2
where s is the length of a side. Here, s=8√2:
A=√34(8√2)2=√34×128=32√3
The magnetic flux Φ through the loop is given by:
Φ=→B⋅→A
where →B=5(^i+^j+^k) T and →A is the area vector of the triangle ABC. The area vector is perpendicular to the plane of the triangle and has a magnitude equal to the area of the triangle.
→A=A^n=32√3^n
where ^n is the unit vector in the direction of ^i+^j+^k. The magnitude of ^n is:
|^n|=√12+12+12=√3
Thus, the unit vector is:
^n=1√3(^i+^j+^k)
Therefore, the area vector becomes:
→A=32√3⋅1√3(^i+^j+^k)=32(^i+^j+^k)
Now we can calculate the dot product:
Φ=→B⋅→A=5(^i+^j+^k)⋅32(^i+^j+^k)=5⋅32⋅(1+1+1)=5⋅32⋅3=480Wb
The quantity of flux through the loop ABCA is Φ=480Wb.
Topper's Solved these Questions
- MOCK TEST 9BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 9EXERCISE - PHYSICS (SECTION 2)
5 Videos
- MOCK TEST 8BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 8EXERCISE - PHYSICS (SECTION 2)
5 Videos
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - IMPECCABLE
52 Videos
Similar Questions
A loop A(0,0,0)B(5,0,0)C(5,5,0)D(0,5,0)E(0,5,10) and F(0,0,10) of straight edges has six corner points and two sides. The magnetic field in this region is →B=(3ˆi+4ˆk) T. The quantity of flux through the loop ABCDEFA (in Wb) is
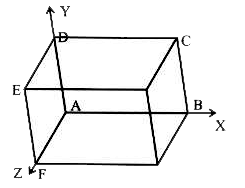
View SolutionA loop A(0,0,0)B(5,0,0)C(5,5,0)D(0,5,0)E(0,5,10) and F(0,0,10) of straight edges has six corner points and two sides. The magnetic field in this region is →B=(3ˆi+4ˆk) T. The quantity of flux through the loop ABCDEFA (in Wb) is

View Solution
Knowledge Check
A square of side x m lies in the x-y plane in a region, when the magntic field is given by →B=B0(3ˆi+4ˆj+5ˆk) T, where B0 is constant. The magnitude of flux passing through the square is
A5B0x2WbB3B0x3WbC2B0x2WbDB0x2Wb
The coordinates of the point equidistant from the points A(0, 0, 0), B(4, 0, 0), C(0, 6, 0) and D(0, 0, 8) is
View SolutionA loop made of straight edges has six corners at A(0,0,0),B(L,O,0),C(L,L,0),D(0,L,0),E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
View SolutionA loop made of straight edegs has six corners at A(0,0,0),B(L,O,0)C(L,L,0),D(0,L,0)E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
View SolutionA loop made of straight edegs has six corners at A(0,0,0),B(L,O,0)C(L,L,0),D(0,L,0)E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
View SolutionA loop made of straight edegs has six corners at A(0,0,0),B(L,O,0)C(L,L,0),D(0,L,0)E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
View SolutionThe magnetic field perpendicular to the plane of a loop of area 0.1m2 is 0.2 T. Calculate the magnetic flux through the loop (in weber)
View SolutionA loop has two semi-circular arcs of radii R(=5√πm) each in two mutually perpendicular planes xz & xy as shown in figure. A uniform & constant magnetic field →B=(3ˆi−4ˆj+5ˆk)T exists in the region of the loop. Radius R=5√πm. The quantity of magnetic flux through the loop ABCDA (in Wb) is ............
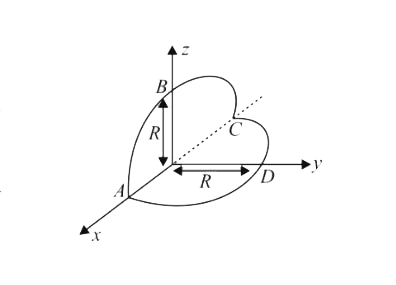
View Solution
