The energy change accompanying the equilibrium reaction A⇔B is −33.0kJmol−1. Calculate Energy of activation for forward and backward reaction (EfandEa) at 300 K. Given that EfandEb are in the ratio 20 : 31. Assume that pre-exponential factor is same for forward and backward reaction.
To solve the problem, we need to calculate the energy of activation for the forward reaction (E_f) and the backward reaction (E_b) using the information provided.
1. Understanding the Reaction and Given Data:
- The equilibrium reaction is A⇌B.
- The energy change (ΔH) for the reaction is given as −33.0kJ mol−1.
- The ratio of the activation energies for the forward and backward reactions is given as Ef:Eb=20:31.
2. Setting Up the Variables:
- Let Ef=20x and Eb=31x where x is a common multiplier.
3. Using the Relationship Between Activation Energies and Enthalpy Change:
- The relationship between the activation energies and the enthalpy change is given by:
ΔH=Ef−Eb
- Substituting the expressions for Ef and Eb:
−33=(20x)−(31x)
- Simplifying this gives:
−33=−11x
4. Solving for x:
- Rearranging the equation:
x=3311=3
5. Calculating Ef and Eb:
- Now substituting x back into the expressions for Ef and Eb:
Ef=20x=20×3=60kJ mol−1
Eb=31x=31×3=93kJ mol−1
6. Final Values:
- The activation energy for the forward reaction Ef is 60kJ mol−1.
- The activation energy for the backward reaction Eb is 93kJ mol−1.
Summary of Results:
- Ef=60kJ mol−1
- Eb=93kJ mol−1
- The equilibrium reaction is A⇌B.
- The energy change (ΔH) for the reaction is given as −33.0kJ mol−1.
- The ratio of the activation energies for the forward and backward reactions is given as Ef:Eb=20:31.
- Let Ef=20x and Eb=31x where x is a common multiplier.
- The relationship between the activation energies and the enthalpy change is given by:
ΔH=Ef−Eb
- Substituting the expressions for Ef and Eb:
−33=(20x)−(31x)
- Simplifying this gives:
−33=−11x
- Rearranging the equation:
x=3311=3
- Now substituting x back into the expressions for Ef and Eb:
Ef=20x=20×3=60kJ mol−1
Eb=31x=31×3=93kJ mol−1
- The activation energy for the forward reaction Ef is 60kJ mol−1.
- The activation energy for the backward reaction Eb is 93kJ mol−1.
- Ef=60kJ mol−1
- Eb=93kJ mol−1
Similar Questions
The energy change accompanying the equilibrium reaction A⇔B is −33.0kJmol−1. Calculate Equilibrium constant Ka for the reaction at 300 K
View SolutionThe energy change accompanying the equilibrium reaction A⇔B is −33.0kJmol−1.
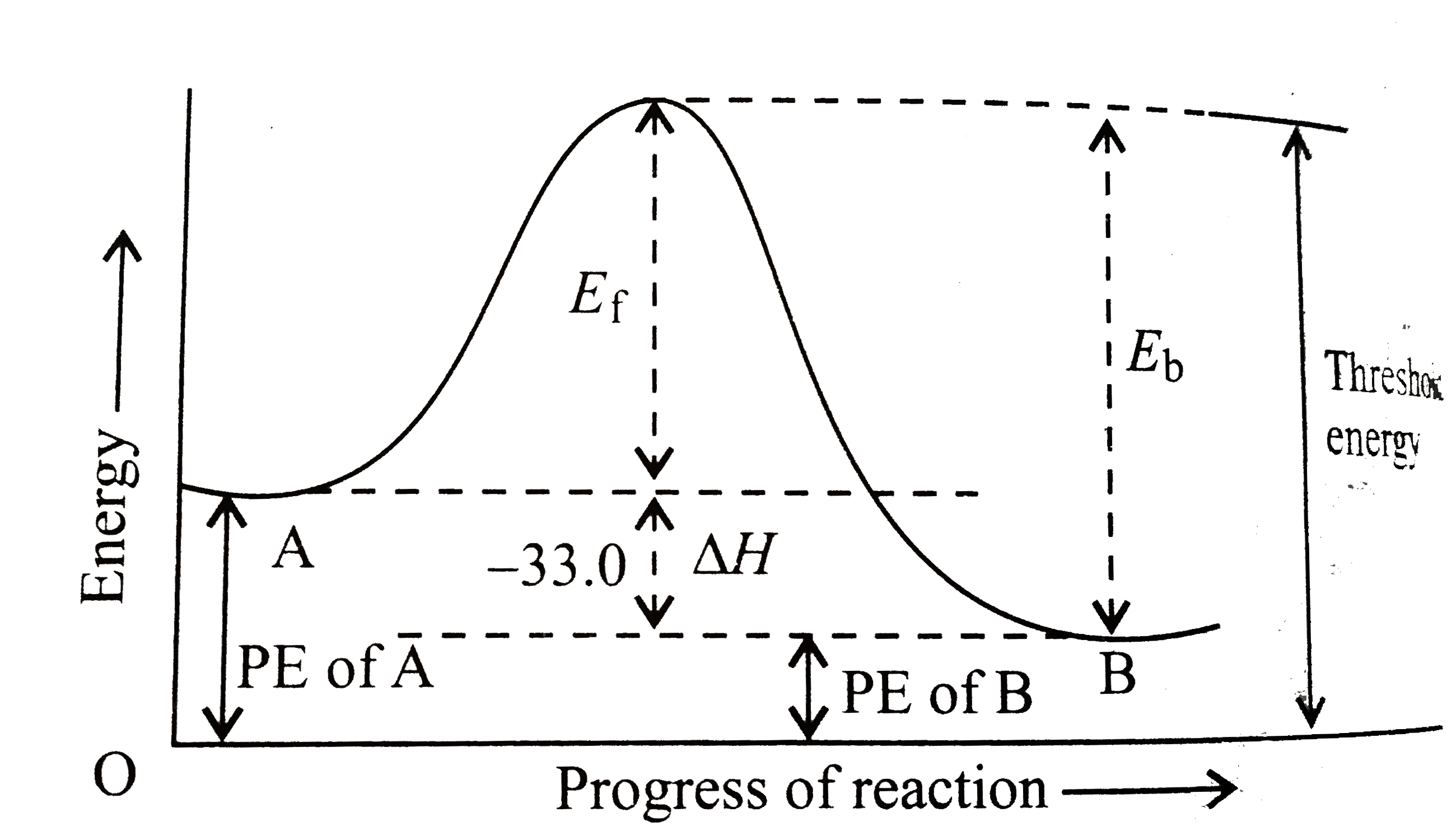
Assuming that pre-exponential factor is same for forward and backward reaction answer the following:
The equilibrium constant k for the reaction at 300K
View SolutionThe energy change accompanying the equilibrium reaction A⇔B is −33.0kJmol−1.
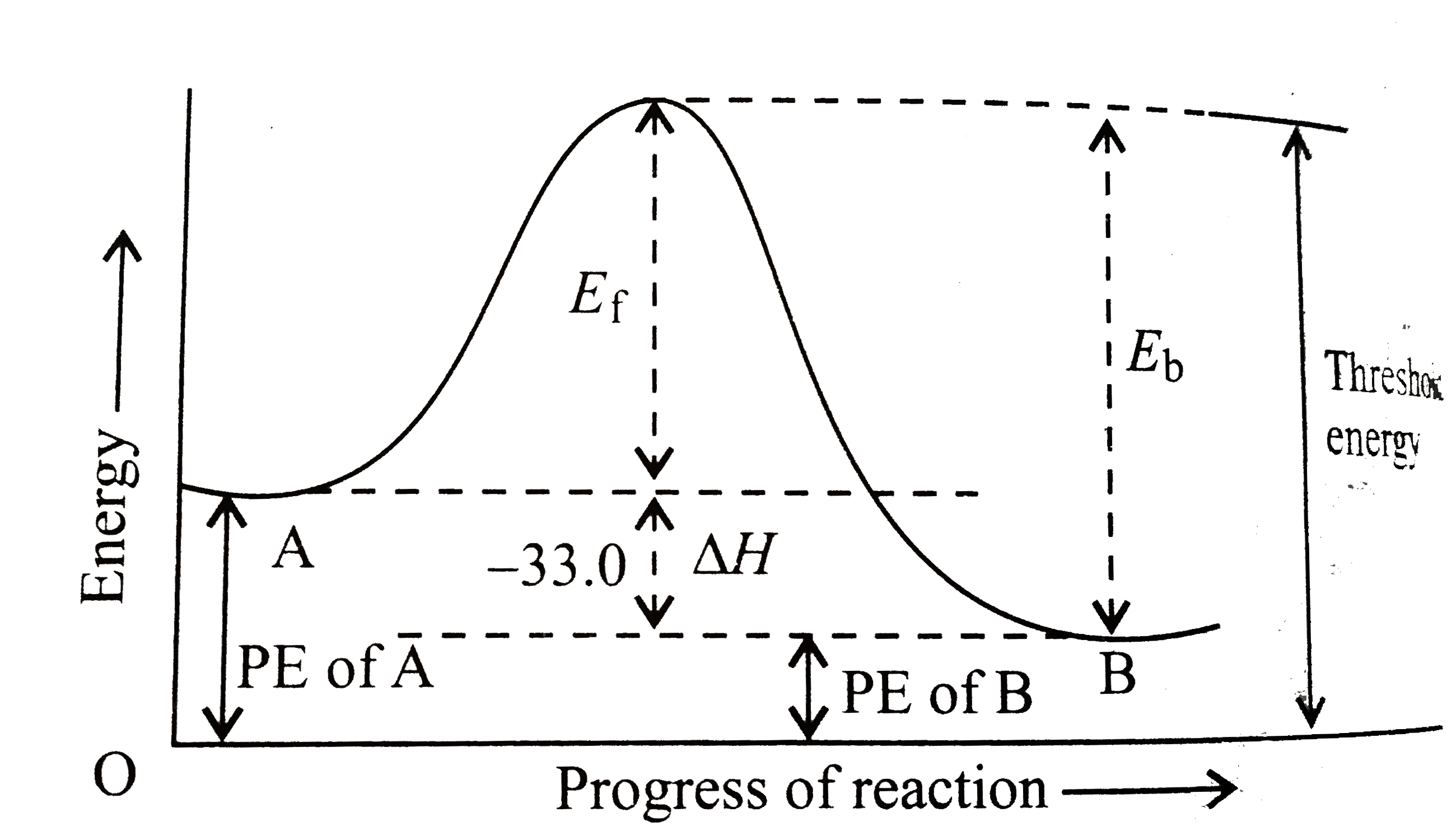
Assuming that pre-exponential factor is same for forward and backward reaction answer the following:
The energy of activation for forward and backward reactions (Ef and Eb) at 300K. Given that Ef and Eb are in the ratio 20:31,
View SolutionFor the reaction,
A⇔B,δH for the reaction is -33.0 kJ/mol.
Calculate
(i) Equilibrium constant Kc for the reaction at 300 K
(ii) If Ea(f)andEa(r) in the ratio 20:31 , calculate Ea(f) at 300 K .
Assuming pre-exponential factor same for forward and reverse reaction.
View SolutionThe energy change accompanying the equilibrium reaction A⇔B is −33.0kJmol−1.
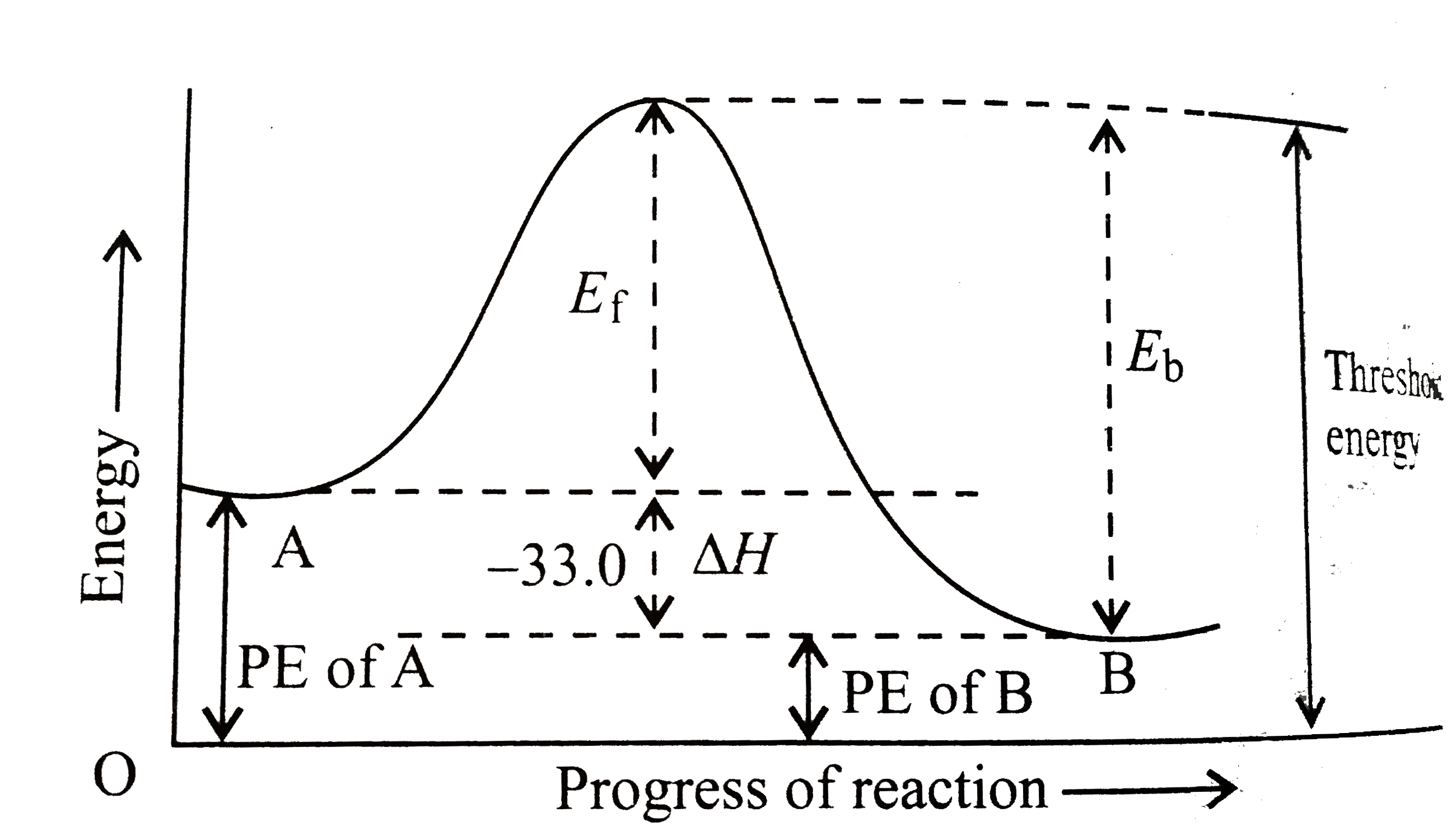
Assuming that pre-exponential factor is same for forward and backward reaction answer the following:
The threshold energy of the reaction is when PE of B is 30kJmol−1.
(a)30
(b)140
(c)123
(d) 125
View SolutionAt equilibrium for a reaction A↔B.
correct representation is {rf= rate of forward reaction, rb = rate of backward reaction}
View SolutionIf the activation energies of the forward and backward reactions of a reversible reaction are Ea(f) and Ea(b), respectively. The ΔE of the reaction is ………..
View SolutionIf Ef and Er are the activation energies of forward and backward reactions and the reaction is known to be exothermic, then
View SolutionThe Ea for forward reaction is 50 kcal. The Ea for its backward reaction is:
View SolutionThe activation energies for forward and backward reactions are EfandEb respectively. If the reaction is exothermic then which is true regarding relation of activation energies?
View Solution