Question

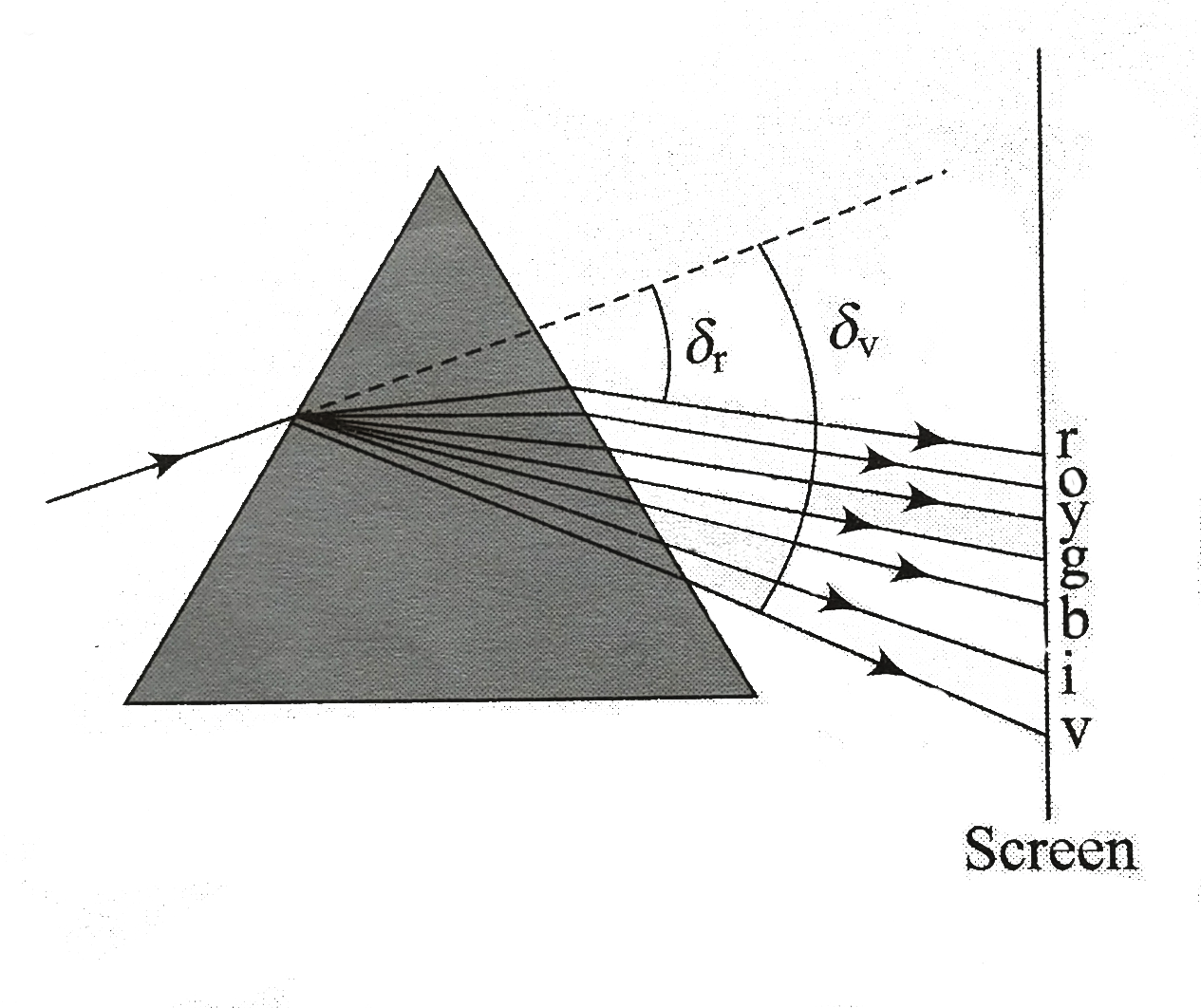
Find the angle of a prism of dispersive power 0.020 and refractive index 1.60 to form an achromatic combination with the prism of angle $4^{\circ }$ and dispersive power 0.040 having refractive index 1.75. Also calculate the resultant deviation.

Explanation

To solve the problem, we need to find the angle of a prism with a dispersive power of 0.020 and a refractive index of 1.60 that will form an achromatic combination with another prism that has an angle of 4 degrees, a dispersive power of 0.040, and a refractive index of 1.75. We will also calculate the resultant deviation.

1. Understanding the Condition for Achromatic Combination:
The condition for two prisms to form an achromatic combination is given by:
\( \omega_1 ( \mu_1 - 1 ) A_1 + \omega_2 ( \mu_2 - 1 ) A_2 = 0 \)
where:
- \( \omega_1 \) and \( \omega_2 \) are the dispersive powers of the prisms,
- \( \mu_1 \) and \( \mu_2 \) are the refractive indices,
- \( A_1 \) and \( A_2 \) are the angles of the prisms.

2. Substituting Known Values:
For the first prism:
- \( \omega_1 = 0.020 \)
- \( \mu_1 = 1.60 \)
- \( A_1 = ? \) (this is what we need to find)

For the second prism:
- \( \omega_2 = 0.040 \)
- \( \mu_2 = 1.75 \)
- \( A_2 = 4^\circ \)

Plugging these values into the equation gives:
\( 0.020 (1.60 - 1) A_1 + 0.040 (1.75 - 1) (4^\circ) = 0 \)

3. Calculating the Terms:
- \( \mu_1 - 1 = 1.60 - 1 = 0.60 \)
- \( \mu_2 - 1 = 1.75 - 1 = 0.75 \)

Now substituting these values:
\( 0.020 \times 0.60 A_1 + 0.040 \times 0.75 \times 4^\circ = 0 \)

4. Simplifying the Equation:
- Calculate \( 0.040 \times 0.75 \times 4 \):
\( 0.040 \times 0.75 = 0.030 \quad \text{and} \quad 0.030 \times 4 = 0.12 \)
So the equation becomes:
\( 0.012 A_1 + 0.12 = 0 \)

5. Solving for \( A_1 \):
Rearranging gives:
\( 0.012 A_1 = -0.12 \)
\( A_1 = \frac{-0.12}{0.012} = -10^\circ \)

6. Calculating the Resultant Deviation:
The resultant deviation \( D \) for the two prisms can be calculated using:
\( D = (\mu_1 - 1) A_1 + (\mu_2 - 1) A_2 \)
Substituting the values:
\( D = (1.60 - 1)(-10^\circ) + (1.75 - 1)(4^\circ) \)
\( D = (0.60)(-10) + (0.75)(4) \)
\( D = -6 + 3 = -3^\circ \)

Final Answers:
- The angle of the first prism \( A_1 = 10^\circ \).
- The resultant deviation \( D = -3^\circ \).