A fighter plane is moving in a vertical circle of radius 'r'. Its minimum velocity at the highest point of the circle will be
√12gr
√2gr
√gr
√3gr
The correct Answer is:3
To find the minimum velocity of a fighter plane moving in a vertical circle at the highest point, we can follow these steps:
Step 1: Understand the forces acting on the plane at the highest point
At the highest point of the vertical circle, the forces acting on the plane are:
- The gravitational force (weight) acting downwards, which is mg.
- The centripetal force required to keep the plane in circular motion, which is provided by the gravitational force at this point.
Step 2: Set up the equation for centripetal force
At the highest point, the centripetal force is given by the equation:
Fc=mv2r
where m is the mass of the plane, v is the velocity of the plane, and r is the radius of the circle.
Step 3: Relate the gravitational force to the centripetal force
At the minimum velocity, the gravitational force provides exactly the centripetal force needed to keep the plane in circular motion. Therefore, we can set the gravitational force equal to the centripetal force:
mg=mv2r
Step 4: Simplify the equation
Since the mass m appears on both sides of the equation, we can cancel it out (assuming m≠0):
g=v2r
Step 5: Solve for the velocity v
Rearranging the equation gives us:
v2=gr
Taking the square root of both sides, we find:
v=√gr
Conclusion
Thus, the minimum velocity of the fighter plane at the highest point of the vertical circle is:
v=√gr
Final Answer
The minimum velocity at the highest point of the circle is √gr.
---
At the highest point of the vertical circle, the forces acting on the plane are:
- The gravitational force (weight) acting downwards, which is mg.
- The centripetal force required to keep the plane in circular motion, which is provided by the gravitational force at this point.
At the highest point, the centripetal force is given by the equation:
Fc=mv2r
where m is the mass of the plane, v is the velocity of the plane, and r is the radius of the circle.
At the minimum velocity, the gravitational force provides exactly the centripetal force needed to keep the plane in circular motion. Therefore, we can set the gravitational force equal to the centripetal force:
mg=mv2r
Since the mass m appears on both sides of the equation, we can cancel it out (assuming m≠0):
g=v2r
Rearranging the equation gives us:
v2=gr
Taking the square root of both sides, we find:
v=√gr
Thus, the minimum velocity of the fighter plane at the highest point of the vertical circle is:
v=√gr
The minimum velocity at the highest point of the circle is √gr.
Topper's Solved these Questions
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - EFFICIENT
50 Videos
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - JEE Advanced (archive)
14 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE ADVANCED (LEVEL -2)
55 Videos
Similar Questions
A particle of mass m attached to an inextensible light string is moving in a vertical circle of radius r. The critical velocity at the highest point is v0 to complete the vertical circle. The tension in the string when it becomes horizontal is
View SolutionA stone is fastened to one end of a string and is whirled in a verticla circle of radius R. Find the minimum speed the stone can have at the highest point of the circle.
View Solution
Knowledge Check
The ride is circus rides along a circular track in a vertical plane. The minimum velocity at the highest point of the track will be
A√(2gR)B√(5gR)C√(3gR)D√(gR)The velocity of a body moving in a vertical circle of radius r is √7gr at the lowest point of the circle. What is the ratio of maximum and minimum tension?
A4:1B√7:1C3:1D2:1
A stone of mass m tied to the end of a string revolves in a vertical circle of radius R. The net forces at the lowest and highest points of the circle directed vertically downwards are: [Choose the correct alternative] Lowest point Highest point
T1 and V1 denote the tension and speed at the lowest point T2 and V2 denote the corresponding values at the highest points.
View SolutionA bead is free to slide down a smooth wire tightly stretched between points A and on a verticle circle of radius R. if the bead starts from rest at 'A', the highest point on the circle, its velocity when it arrives at B is :-
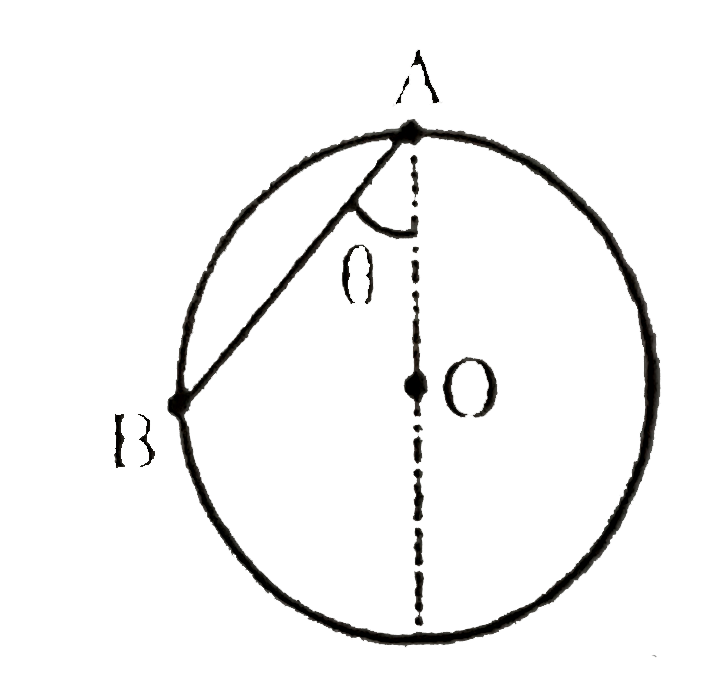
View SolutionA stone of mass 1kg is tied with a string and it is whirled in a vertical circle of radius 1 m. If tension at the highest point is 14 N, then velocity at lowest point will be
View SolutionA body is moving in a vertical circle of radius r such that the string is just taut at its highest point. The speed of the particle when the string is horizontal is
View SolutionA particle of mass m is being circulated on a vertical circle of radius r. If the speed of particle at the highest point be v, then
View SolutionA small particle of mass m attached with a light inextensible thread of length L is moving in a vertical circle. In the given case particle is moving in complete vertical circle and ratio of its maximum to minimum velocity is 2:1 .
Velocity of the particle when it is moving vertically downward is

View Solution
