Two solution of H2SO4 of molarities x and y are mixed in the ratio of V1mL:V2mL to form a solution of molarity M1. If they are mixed in the ratio of V2mL:V1mL, they form a solution of molarity M2. Given V1/V2=xy>1andM1M2=54, then x:y is
2:1
4:1
1:2
3:1
To solve the problem, we need to analyze the mixing of two solutions of sulfuric acid (H₂SO₄) with molarities x and y. The solutions are mixed in two different volume ratios to yield two different molarities, M₁ and M₂.
1. Understanding the Problem:
- We have two solutions with molarities x and y.
- They are mixed in the ratio V₁ : V₂ to form a solution of molarity M₁.
- When mixed in the ratio V₂ : V₁, they form a solution of molarity M₂.
- We are given that V₁/V₂ = x/y > 1 and M₁/M₂ = 5/4.
2. Setting Up the Equations:
- From the first mixing scenario (V₁ : V₂), we can write the equation for molarity:
M1=xV1+yV2V1+V2
- From the second mixing scenario (V₂ : V₁), we have:
M2=xV2+yV1V2+V1
3. Using the Given Ratios:
- We know that M1M2=54. Therefore:
xV1+yV2xV2+yV1=54
4. Cross-Multiplying:
- Cross-multiplying gives us:
4(xV1+yV2)=5(xV2+yV1)
5. Expanding the Equation:
- Expanding both sides:
4xV1+4yV2=5xV2+5yV1
6. Rearranging the Terms:
- Rearranging gives:
4xV1−5yV1=5xV2−4yV2
- Factoring out common terms:
V1(4x−5y)=V2(5x−4y)
7. Substituting the Ratio:
- We know V1V2=xy. Let V1=kx and V2=ky for some constant k:
kx(4x−5y)=ky(5x−4y)
- Dividing both sides by k (assuming k ≠ 0):
x(4x−5y)=y(5x−4y)
8. Expanding and Rearranging:
- Expanding gives:
4x2−5xy=5xy−4y2
- Rearranging results in:
4x2−10xy+4y2=0
9. Factoring the Quadratic:
- This can be factored as:
(2x−2y)(2x−2y)=0
- Thus, we have:
2x=2y⟹x=2y
10. Finding the Ratio:
- Therefore, the ratio x:y=2:1.
Final Answer:
The ratio of x to y is 2:1.
- We have two solutions with molarities x and y.
- They are mixed in the ratio V₁ : V₂ to form a solution of molarity M₁.
- When mixed in the ratio V₂ : V₁, they form a solution of molarity M₂.
- We are given that V₁/V₂ = x/y > 1 and M₁/M₂ = 5/4.
- From the first mixing scenario (V₁ : V₂), we can write the equation for molarity:
M1=xV1+yV2V1+V2
- From the second mixing scenario (V₂ : V₁), we have:
M2=xV2+yV1V2+V1
- We know that M1M2=54. Therefore:
xV1+yV2xV2+yV1=54
- Cross-multiplying gives us:
4(xV1+yV2)=5(xV2+yV1)
- Expanding both sides:
4xV1+4yV2=5xV2+5yV1
- Rearranging gives:
4xV1−5yV1=5xV2−4yV2
- Factoring out common terms:
V1(4x−5y)=V2(5x−4y)
- We know V1V2=xy. Let V1=kx and V2=ky for some constant k:
kx(4x−5y)=ky(5x−4y)
- Dividing both sides by k (assuming k ≠ 0):
x(4x−5y)=y(5x−4y)
- Expanding gives:
4x2−5xy=5xy−4y2
- Rearranging results in:
4x2−10xy+4y2=0
- This can be factored as:
(2x−2y)(2x−2y)=0
- Thus, we have:
2x=2y⟹x=2y
- Therefore, the ratio x:y=2:1.
The ratio of x to y is 2:1.
Topper's Solved these Questions
- THEORY OF SOLUTIONSBOOK - VMC MODULES ENGLISHCHAPTER - THEORY OF SOLUTIONSEXERCISE - LEVEL 2
65 Videos
- THEORY OF SOLUTIONSBOOK - VMC MODULES ENGLISHCHAPTER - THEORY OF SOLUTIONSEXERCISE - JEE Main (Archive)
57 Videos
- THEORY OF SOLUTIONSBOOK - VMC MODULES ENGLISHCHAPTER - THEORY OF SOLUTIONSEXERCISE - JEE Advanced (Archive)
31 Videos
- THE SOLID STATEBOOK - VMC MODULES ENGLISHCHAPTER - THE SOLID STATEEXERCISE - EXERCISE-J
10 Videos
- THERMOCHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - THERMOCHEMISTRYEXERCISE - JEE ADVANCED (ARCHIVE)
31 Videos
Similar Questions
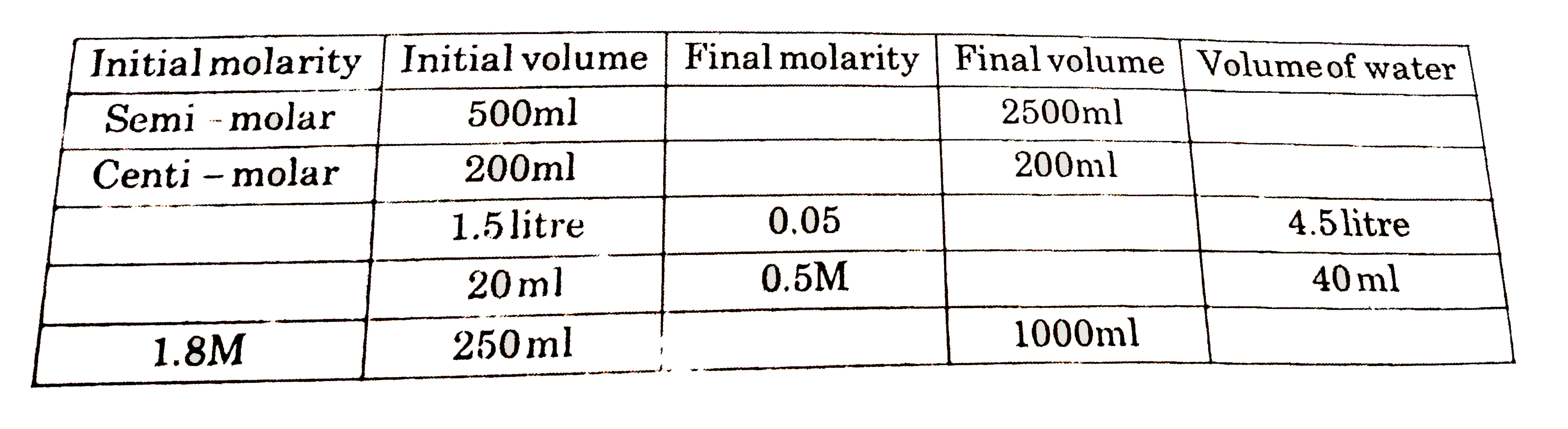
Use of dilution formula (M1V1=M2V2)
View SolutionIn the titration of K2Cr2O7 and ferrous sulphate, following data is obtained:
V1 mL of K2Cr2O7 solution of molarity M1 requires V2mL of FeSO4 solution of molarity M2.
Which of the following relations is /are true for the above titration?
View Solution1MHCland2MHCl are mixed in volume ratio 4:1. What is the final molarity of HCl solution?
View Solution1MHCland2MHCl are mixed in volume ratio 4:1. What is the final molarity of HCl solution?
View Solution500 ml of aM and 500 ml of bM solution of a solute are mixed and diluted to 2 litre to prepare a solution of 1.5 M. If a and b are in the ratio 2 : 1, then the value of a is.
View SolutionV1mL of CH3COONa solution (of molarity M1) and V2mL of a HCl solution (of Molarity M2) are available ,Can the two be mixed to obtain a buffer solution? If Yes, what should be the mathematical condition relating M1,M2,V1&V2 for this?
View Solution100ml of 2.45%(w/v)H2SO4 solution is mixed with 200ml of 7%(w/w)H2SO4 solution (density=1.4gm/ml) and the mixture is diluted to 500ml. What is the molarity of the diluted solution ?
View SolutionTwo solutions of a substance (non-electroyte) are mixed in the following manner 480mL of 1.5M of first solution with 520mLor1.2M of second solution. The molarity of final solution is:
View Solution20 ml of 0.4 MH2SO4 , and 80 ml of 0.2 M NaOH are mixed. Then the pH of the resulting solution is
View SolutionWhen V ml of 2.2MH2SO4 solution is mixed with 10 V ml of water, the volume contraction of 2% take place. Calculate the molarity of diluted solution ?
View Solution
