The relation between ΔU is :
ΔH=ΔU+RT
ΔH=ΔU−ΔgnRT
ΔH=ΔU+ΔgnRT
ΔU=ΔH+ΔgnRT
The correct Answer is:C
To derive the relationship between the change in internal energy (ΔU) and the change in enthalpy (ΔH), we can follow these steps:
Step 1: Start with the definition of enthalpy
The enthalpy (H) is defined as:
H=U+PV
where U is the internal energy, P is the pressure, and V is the volume.
Step 2: Differentiate the enthalpy
Taking the differential of both sides, we have:
dH=dU+PdV+VdP
Step 3: Consider constant pressure conditions
Under constant pressure conditions (which is often the case in chemical reactions), dP=0. Therefore, the equation simplifies to:
dH=dU+PdV
Step 4: Relate changes in volume to the number of moles
For reactions involving gases, we can express the change in volume in terms of the change in the number of moles of gas. If n1 is the number of moles of gaseous reactants and n2 is the number of moles of gaseous products, then:
Δn=n2−n1
Step 5: Use the ideal gas law
According to the ideal gas law, we have:
PV=nRT
Thus, for the reactants and products, we can write:
- For reactants: PV1=n1RT
- For products: PV2=n2RT
Step 6: Substitute into the enthalpy equation
Substituting PdV (where dV=V2−V1) into the equation gives:
dH=dU+P(V2−V1)
Using the ideal gas law, we can express this as:
dH=dU+RT(n2−n1)
Step 7: Final relationship
Thus, we arrive at the relationship:
ΔH=ΔU+ΔnRT
where Δn=n2−n1.
Conclusion
The final relationship between the change in enthalpy and the change in internal energy is:
ΔH=ΔU+ΔnRT
---
The enthalpy (H) is defined as:
H=U+PV
where U is the internal energy, P is the pressure, and V is the volume.
Taking the differential of both sides, we have:
dH=dU+PdV+VdP
Under constant pressure conditions (which is often the case in chemical reactions), dP=0. Therefore, the equation simplifies to:
dH=dU+PdV
For reactions involving gases, we can express the change in volume in terms of the change in the number of moles of gas. If n1 is the number of moles of gaseous reactants and n2 is the number of moles of gaseous products, then:
Δn=n2−n1
According to the ideal gas law, we have:
PV=nRT
Thus, for the reactants and products, we can write:
- For reactants: PV1=n1RT
- For products: PV2=n2RT
Substituting PdV (where dV=V2−V1) into the equation gives:
dH=dU+P(V2−V1)
Using the ideal gas law, we can express this as:
dH=dU+RT(n2−n1)
Thus, we arrive at the relationship:
ΔH=ΔU+ΔnRT
where Δn=n2−n1.
The final relationship between the change in enthalpy and the change in internal energy is:
ΔH=ΔU+ΔnRT
Topper's Solved these Questions
- THERMOCHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - THERMOCHEMISTRYEXERCISE - LEVEL-2
65 Videos
- THERMOCHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - THERMOCHEMISTRYEXERCISE - JEE MAIN (ARCHIVE)
20 Videos
- THERMOCHEMISTRYBOOK - VMC MODULES ENGLISHCHAPTER - THERMOCHEMISTRYEXERCISE - LEVEL - 0 Long Answer Type
13 Videos
- THEORY OF SOLUTIONSBOOK - VMC MODULES ENGLISHCHAPTER - THEORY OF SOLUTIONSEXERCISE - JEE Advanced (Archive)
31 Videos
- THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE)
44 Videos
Similar Questions
The enthalpy change for chemical reaction is denoted as ΔHΘ and ΔHΘ=HΘP−HΘR. The relation between enthalpy and internal energy is expressed by equation:
ΔH=ΔU+ΔnRT
where ΔU= change in internal energy Δn= change in number of moles, R= gas constant.
Enthalpy of the system is given as
View SolutionThe enthalpy change for chemical reaction is denoted aas ΔHΘ and ΔHΘ=HΘP−HΘR. The relation between enthalpy and internal enegry is expressed by equation:
ΔH=ΔU+ΔnRT
where ΔU= change in internal enegry Δn= change in number of moles, R= gas constant.
For a reaction, 2X(s)+2Y(s)→2C(l)+D(g),ΔH at 27∘C is −28kcalmol−1.ΔU is .....kcalmol−1
View Solution
Knowledge Check
Which of the following relationships is not correct for the relation between ΔHandΔU?
AWhen Δng=0 then ΔH=ΔUBWhen Δng>0 then ΔH>ΔUCWhen Δng<0 then ΔH<ΔUDWhen ΔngRT=0 then ΔH<ΔUThe relation between Y. η and B is
A1Y=13η+19BB9Y=1η+3BC1η=1B+1YD9Y=3η+1B
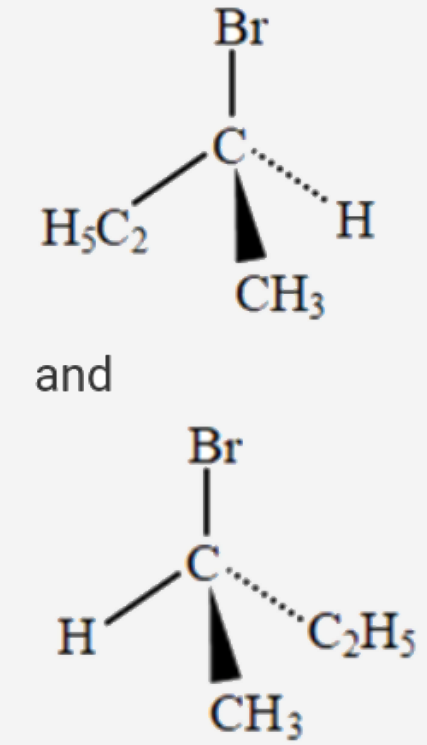
The relation between given pair is
View SolutionThe enthalpy change for chemical reaction is denoted as ΔHΘ and ΔHΘ=HΘP−HΘR. The relation between enthalpy and internal enegry is expressed by equation:
ΔH=ΔU+ΔnRT
where ΔU= change in internal enegry Δn= change in number of moles, R= gas constant.
For the change, Cdiamond→Cgraphite,ΔH=−1.89kJ, if 6g of diamond and 6g of graphite are seperately burnt to yield CO2 the heat liberated in first case is
View SolutionThe enthalpy change for chemical reaction is denoted aas ΔHΘ and ΔHΘ=HΘP−HΘR. The relation between enthalpy and internal enegry is expressed by equation:
ΔH=ΔU+ΔnRT
where ΔU= change in internal enegry Δn= change in number of moles, R= gas constant.
Enthalpy of the system is given as
View SolutionThe enthalpy change for chemical reaction is denoted aas ΔHΘ and ΔHΘ=HΘP−HΘR. The relation between enthalpy and internal energy is expressed by equation:
ΔH=ΔU+ΔnRT
where ΔU= change in internal energy Δn= change in number of moles, R= gas constant.
Which of the following equations corresponds to the definition of enthalpy of formation at 298K?
View SolutionWhat is the relation between Velocity, displacement and time?
View SolutionGive the relation between Kc and Delta G
View Solution
