At a given temperature T, gases Ne, Ar, Xe and Kr are found to deviate from ideal gas behavior.
Their equation of state is given as P=RTV−b at T. Here, b is the van der Waals constant. Which gas will exhibit steepest increase in the plot of Z (compression factor) vs P?
Xe
Ne
Kr
Ar
The correct Answer is:A
To determine which gas among Ne, Ar, Xe, and Kr will exhibit the steepest increase in the plot of the compressibility factor (Z) versus pressure (P), we can follow these steps:
1. Understand the Compressibility Factor (Z):
The compressibility factor Z is defined as:
Z=PVRT
For real gases, Z deviates from 1, especially under high pressures.
2. Use the Given Equation of State:
The equation of state provided is:
P=RTV−b
Rearranging this gives us:
V=RTP+b
3. Substituting into the Compressibility Factor Equation:
Substitute V from the rearranged equation into the Z equation:
Z=P(RTP+b)RT=1+PbRT
4. Analyze the Relationship:
From the equation Z=1+PbRT, we see that Z is directly proportional to the van der Waals constant b:
Z∝b
This indicates that the larger the value of b, the steeper the increase of Z with pressure P.
5. Identify the Van der Waals Constant (b):
The value of b is related to the size of the gas atoms. Larger atoms have larger values of b. The order of atomic size for the given gases is:
- Neon (Ne) < Argon (Ar) < Krypton (Kr) < Xenon (Xe)
6. Conclusion:
Since Xenon (Xe) has the largest atomic size, it will have the largest value of b and therefore will exhibit the steepest increase in the plot of Z versus P.
Final Answer:
The gas that will exhibit the steepest increase in the plot of Z versus P is Xenon (Xe).
The compressibility factor Z is defined as:
Z=PVRT
For real gases, Z deviates from 1, especially under high pressures.
The equation of state provided is:
P=RTV−b
Rearranging this gives us:
V=RTP+b
Substitute V from the rearranged equation into the Z equation:
Z=P(RTP+b)RT=1+PbRT
From the equation Z=1+PbRT, we see that Z is directly proportional to the van der Waals constant b:
Z∝b
This indicates that the larger the value of b, the steeper the increase of Z with pressure P.
The value of b is related to the size of the gas atoms. Larger atoms have larger values of b. The order of atomic size for the given gases is:
- Neon (Ne) < Argon (Ar) < Krypton (Kr) < Xenon (Xe)
Since Xenon (Xe) has the largest atomic size, it will have the largest value of b and therefore will exhibit the steepest increase in the plot of Z versus P.
The gas that will exhibit the steepest increase in the plot of Z versus P is Xenon (Xe).
Topper's Solved these Questions
- STATES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - JEE-Advanced
78 Videos
- STATES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - ILLUSTRATION
30 Videos
- STATES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Level-2 (NUMBERICAL VALUE TYPE FOR JEE MAIN
15 Videos
- STATES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - IMPECCABLE
50 Videos
- STOICHIOMETRY - IBOOK - VMC MODULES ENGLISHCHAPTER - STOICHIOMETRY - IEXERCISE - JEE Advanced (Archive)
31 Videos
Similar Questions
At a constant temperature Ne,Ar,Kr and Xe devite from ideal behaviro according to equation
P=RTVm−b
View SolutionThe equation of state for real gas is given by ((p+aV2(V−b)=RT. The dimension of the constant a is ………………. .
View SolutionIf v is the volume of one molecule of a gas under given conditions, then van der Waals constant b is
View SolutionOne way of writing the equation of state for real gases is
PV=RT[1+BV+….]
where B is a constant. Derive an approximate expression for B in terms of the van der Waals constants a and b.
View SolutionBoyle's temperature (TB) : It is the temperature at which a real gas exhibits ideal behavior in low pressure range. Different gases have different value of TB, which is related to the van der Wall's constant a and ab as follows: TB=aRb
The compressiblity factor for a real gas is given as Z=1+0.35P−168TP. Where P is in bar and T is in Kelvin. What is the Boyle's temperature of the gas?
View SolutionOne way of writing the equation of state for real gases is PV=RT[1+RV+…] ? Where B is constant. Derive an approximate expression for B in terms of the van der Waals constant a and b.
View SolutionAt low pressure the van der Waals' equation is reduced to [P+aV2]V=RT The compressibility factor can be given as .
View SolutionAt a particular temperature and pressure for a real gas Van der Waal's equation can be written as:
(P+aV2m)(Vm−b)=RT
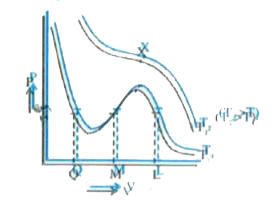
where Vm is molar volume of gas. This is cubic equation in the variable Vm and therefore for any single value of P & T there should be 3 values of Vm. Which are shown in graph as Q, M and L.
As temperature is made to increase at a certain higher temperature the three values of Vm becomes identical. The temperature, pressure & molar volume at point X are called Tc, Pc & Vc for real gas. The compressibility factor in terms of Pc, Vc and T is called Zc.
The expression of Van dcr Waal's constant 'a' can be given as
View SolutionBoyle's temperature or Boyle point is the temperature at which a real gas starts behaving like an ideal gas over a particular range of pressure. A graph is plotted between compressibility factor Z and pressure P.

What is the deviation of real gas from ideal behaviour in terms of compressibility factor , Z ?
View SolutionIf the equation of state of a gas is expressed as (P+aV2)(V−b)=RT where P is the pressure, V is the volume and T the absolute temperature and a, b , R are constants, then find the dimensions of 'a' and 'b' ?
View Solution