A metallic carbonyl is in gaseous state. The rate of diffusion of is 3.31 time faster than this gaseous carbonyl under identical conditions. If atomic mass of metal is 63.29, the closest integer value of X is___________.
The correct Answer is:4
To solve the problem, we will use Graham's law of effusion, which states that the rate of diffusion of a gas is inversely proportional to the square root of its molar mass.
1. Understanding the Problem:
We know that the rate of diffusion of methane (CH₄) is 3.31 times faster than that of the metallic carbonyl M(CO)x. We need to find the value of x given that the atomic mass of the metal M is 63.29 g/mol.
2. Using Graham's Law:
According to Graham's law:
Rate of CH4Rate of M(CO)x=√MM(CO)xMCH4
Given that:
Rate of CH4Rate of M(CO)x=3.31
3. Calculating Molar Mass of CH₄:
The molar mass of methane (CH₄) is calculated as follows:
- Carbon (C) = 12 g/mol
- Hydrogen (H) = 1 g/mol × 4 = 4 g/mol
- Total molar mass of CH₄ = 12 + 4 = 16 g/mol
4. Calculating Molar Mass of M(CO)x:
The molar mass of the metallic carbonyl M(CO)x can be expressed as:
MM(CO)x=M+x×MCO
where MCO (molar mass of CO) = 12 (C) + 16 (O) = 28 g/mol.
Therefore:
MM(CO)x=63.29+28x
5. Setting Up the Equation:
Substitute the values into Graham's law:
3.31=√63.29+28x16
6. Squaring Both Sides:
To eliminate the square root, square both sides:
(3.31)2=63.29+28x16
Calculate (3.31)2:
3.312=10.9561
Therefore:
10.9561=63.29+28x16
7. Multiplying Both Sides by 16:
Multiply both sides by 16 to isolate the numerator:
10.9561×16=63.29+28x
Calculate:
175.2976=63.29+28x
8. Solving for x:
Rearranging the equation gives:
28x=175.2976−63.29
Calculate:
28x=112.0076
Dividing by 28:
x=112.007628≈4
9. Conclusion:
The closest integer value of x is 4.
Final Answer:
The closest integer value of x is 4.
We know that the rate of diffusion of methane (CH₄) is 3.31 times faster than that of the metallic carbonyl M(CO)x. We need to find the value of x given that the atomic mass of the metal M is 63.29 g/mol.
According to Graham's law:
Rate of CH4Rate of M(CO)x=√MM(CO)xMCH4
Given that:
Rate of CH4Rate of M(CO)x=3.31
The molar mass of methane (CH₄) is calculated as follows:
- Carbon (C) = 12 g/mol
- Hydrogen (H) = 1 g/mol × 4 = 4 g/mol
- Total molar mass of CH₄ = 12 + 4 = 16 g/mol
The molar mass of the metallic carbonyl M(CO)x can be expressed as:
MM(CO)x=M+x×MCO
where MCO (molar mass of CO) = 12 (C) + 16 (O) = 28 g/mol.
Therefore:
MM(CO)x=63.29+28x
Substitute the values into Graham's law:
3.31=√63.29+28x16
To eliminate the square root, square both sides:
(3.31)2=63.29+28x16
Calculate (3.31)2:
3.312=10.9561
Therefore:
10.9561=63.29+28x16
Multiply both sides by 16 to isolate the numerator:
10.9561×16=63.29+28x
Calculate:
175.2976=63.29+28x
Rearranging the equation gives:
28x=175.2976−63.29
Calculate:
28x=112.0076
Dividing by 28:
x=112.007628≈4
The closest integer value of x is 4.
The closest integer value of x is 4.
Topper's Solved these Questions
- STATES OF MATTERBook:VMC MODULES ENGLISHChapter:STATES OF MATTERExercise:JEE-Main ( ARCHIVE )
Explore 17 Videos
- STATES OF MATTERBook:VMC MODULES ENGLISHChapter:STATES OF MATTERExercise:JEE-Advanced
Explore 78 Videos
- STATES OF MATTERBook:VMC MODULES ENGLISHChapter:STATES OF MATTERExercise:Level-2
Explore 50 Videos
- STATES OF MATTERBook:VMC MODULES ENGLISHChapter:STATES OF MATTERExercise:IMPECCABLE
Explore 50 Videos
- STOICHIOMETRY - IBook:VMC MODULES ENGLISHChapter:STOICHIOMETRY - IExercise:JEE Advanced (Archive)
Explore 31 Videos
Similar Questions
EAN of a metal carbonyl M(CO)x is 36. If atomic number of metal M is 26, what is the value of x?
View SolutionThe density of vapours of a substance at 1 atm and 500 K is 0.3kgm−3. The vapours effuse 0.4216 times faster than O2 through a pin hole under identical conditions. If R= 0.08 litre atm K−1mol−1. The molar volume of gas is a×102 litre. The value of a is _____________.
View SolutionA fluoride of phosphorous in gaseous state was found to diffuse 2.12 times more slowly than nitrogen under similar conditions. Calculate the molecular mass and molecular formula of this fluoride which contains one atom of phosphorous per molecule.
View SolutionIn metal carbonyl having and genral formula M(CO)x, where, M = metal, x = 4 and the metal is bonded to
View SolutionA current of 5.0 A flows for 4.0 h through an electrolytic cell containing a molten slat of metal M. This result in deposition of 0.25 mol of the metal M at the cathode. The oxidation state of M in the molten salt is +x. The value of 'x' is (1 Faraday = 96000 C mol−1) 3
View SolutionThe density of vapour of a substance (X) at 1 atm pressure and 500 K is 0.8kg/m3. The vapour effuse through a small hole at a rate of 4/5 times slower than oxygen under the same condition. What is the compressibility factor (z) of the vapour ?
View SolutionA thermocol vessel contains 0.5kg of distilled water at 30∘C. A metal coil of area 5×10−3m2, number of turns 100, mass 0.06kg and resistance 1.6Ω is lying horizontally at the bottom of the vessel. A uniform time-varying magnetic field is set up to pass vertically through the coil at time t=0. The field is first increased from zero to 0.8T at a constant rate between 0 and 0.2s and then decreased to zero at the same rate between 0.2and0.4s. the cycle is repeated 12000 times. Make sketches of the current through the coil and the power dissipated in the coil as function of time for the first two cycles. Clearly indicate the magnitude of the quantities on the axes. Assumes that no heat is lost to the vessel or the surroundings. Determine the final tempreture of water under thermal equilibrium. Specific heat of metal =500jkg−1K−1 and the specific heat of water =4200jkg−1K−1. Neglect the inductance of coil.
View SolutionThe existence of negatively charged particle in an atom was shown by J.J. Thomson as a result of the studies of the passage of electricity through gases at extremely low pressure known as discharge tube experiments. When a high voltage of the order of 10,000 volts or more was impressed across the electrodes, some sort of invisible rays moved from the negative electrode to the positive electrode these rays are called as cathode rays.
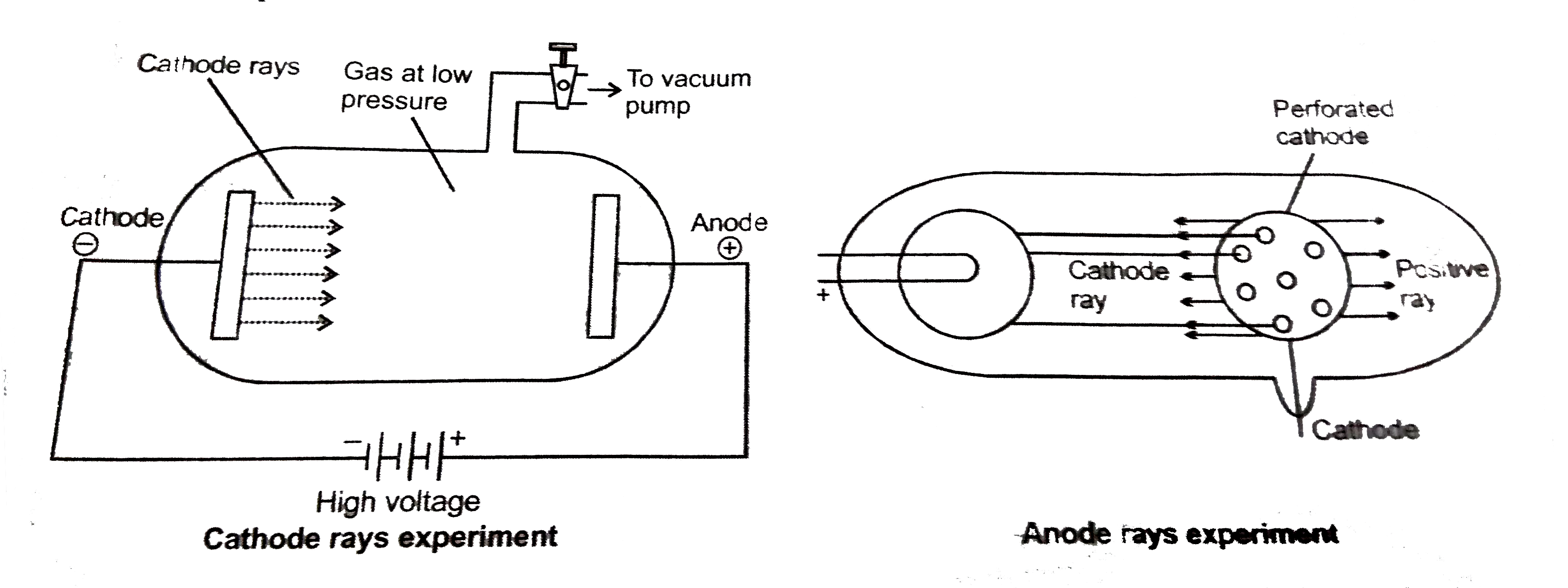
Cathode rays travel in straight path in absence of electrical and magnetic field . Cathode rays consist of material part and charged particles? Cathode rays produce X-rays and light is emitted when they strike on ZnS screen. Cathode rays penetrate through thin sheets of aluminium and other metals . They affect the photogenic plate and passes heating effect when they strike on metal foil. The raito of charge to mass i.e charge/mass is same for all the cathode rays irrespective of the gas used in the tube.
The existence of positively charged particle in an atom was shown be E. Goldstein. He repeated the same discharge tube experiments by using a perforated cathode. It was observed that when a high potential difference was applied between the electrodes, not only cathode rays were produced but also a new type of rays were produced simultaneoulsy from anode moving towards cathode and passes through the holes or canal of the cathode. These termed as canal rays or anode rays.
These rays travel in straight lines and consists of positively charged particles. These rays have kinetic energy and produces heating effect also. The e/m ratio of these rays is smaller than that of electrons. Unlike cathode rays, their e/m value is dependent upon the nature of the gas taken in the tube. These rays produced flashes of light on ZnS screen and can pass throughs thin metal foils. They can produce physical and chemical changes and are capable to produce ionisation in gases.
For cathode rays the value of e/m:
View SolutionUnder identical conditions of pressure and temperature, 4 L of gaseous moxture (H2 and CH4) effuses through a hole in 5 min whereas 4 L of a gas X of molecular mass 36 takes to 10 min to effuse through the same hole. The mole ratio of H2:CH4 in the mixture is:
View SolutionA mixture of C3H8 and CH4 exerts a pressure of 320 mm Hg at temperature T K in a V litre flask. On complete combustion, gaseous mixture contains CO2 only and exerts a pressure of 448 mm Hg under identical conditions. Hence mole fraction of C3H8 in the mixture is
View Solution