The ratio of Van Der Waal's constants a and b, (ab) has the dimension of :
atm L−1
L atm mo1−1
L mo1−2
atm L mo1−2
The correct Answer is:B
To solve the problem of finding the dimension of the ratio of Van der Waals constants a and b, we will follow these steps:
Step 1: Understand the Van der Waals equation
The Van der Waals equation is given by:
P+n2aV2=nRTV−nB
Where:
- P = pressure
- V = volume
- n = number of moles
- R = universal gas constant
- T = absolute temperature
- a and b = Van der Waals constants
Step 2: Find the dimension of constant a
From the equation, we can isolate a:
P=n2aV2⟹a=PV2n2
Now, we need to determine the dimensions of a:
- Dimension of pressure P is (P)=ML−1T−2
- Dimension of volume V is (V)=L3
- Dimension of number of moles n is (n)=mol
Substituting these into the equation for a:
(a)=(ML−1T−2)(L3)2(mol)2=ML−1T−2⋅L6mol2=ML5mol2T2
Step 3: Find the dimension of constant b
From the equation, we can isolate b:
V−nB=V⟹nB=V⟹B=Vn
Now, we need to determine the dimensions of b:
(b)=(L3)(mol)=L3⋅mol−1
Step 4: Find the ratio ab
Now we can find the ratio of a and b:
ab=ML5mol2T2L3⋅mol−1=ML5mol2T2⋅molL3=ML5L3molT2=ML2molT2
Step 5: Final dimension of ab
The final dimension simplifies to:
ab=L2⋅atm⋅mol−1
This corresponds to the dimension of L⋅atm⋅mol−1.
Conclusion
The dimension of the ratio ab is:
L⋅atm⋅mol−1
The Van der Waals equation is given by:
P+n2aV2=nRTV−nB
Where:
- P = pressure
- V = volume
- n = number of moles
- R = universal gas constant
- T = absolute temperature
- a and b = Van der Waals constants
From the equation, we can isolate a:
P=n2aV2⟹a=PV2n2
Now, we need to determine the dimensions of a:
- Dimension of pressure P is (P)=ML−1T−2
- Dimension of volume V is (V)=L3
- Dimension of number of moles n is (n)=mol
(a)=(ML−1T−2)(L3)2(mol)2=ML−1T−2⋅L6mol2=ML5mol2T2
From the equation, we can isolate b:
V−nB=V⟹nB=V⟹B=Vn
Now, we need to determine the dimensions of b:
(b)=(L3)(mol)=L3⋅mol−1
Now we can find the ratio of a and b:
ab=ML5mol2T2L3⋅mol−1=ML5mol2T2⋅molL3=ML5L3molT2=ML2molT2
The final dimension simplifies to:
ab=L2⋅atm⋅mol−1
This corresponds to the dimension of L⋅atm⋅mol−1.
The dimension of the ratio ab is:
L⋅atm⋅mol−1
Topper's Solved these Questions
- STATES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Level-2
50 Videos
- STATES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - Level-2 (NUMBERICAL VALUE TYPE FOR JEE MAIN
15 Videos
- STATES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - LEVEL -0 (Short Answer Type-II)
27 Videos
- STATES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - STATES OF MATTEREXERCISE - IMPECCABLE
50 Videos
- STOICHIOMETRY - IBOOK - VMC MODULES ENGLISHCHAPTER - STOICHIOMETRY - IEXERCISE - JEE Advanced (Archive)
31 Videos
Similar Questions
The ratio of units of van der Waals constants a and b is
View SolutionThe van der Waal's gas constant, a is given by
View SolutionUnits of van der Waal's constants 'a' and 'b' are respectively
View SolutionWrite the units of van der Waals' constant 'a' and 'b'.
View SolutionThe value of van der Waals constant a is the maximum for
View SolutionVan der Waal's constant 'a' has the fimensions of
View SolutionIn the van der Waals equation
View SolutionFor three different gases, value of van der waals' constant 'a' and 'b' are given. What is the correct orde of liquefaction of gases?
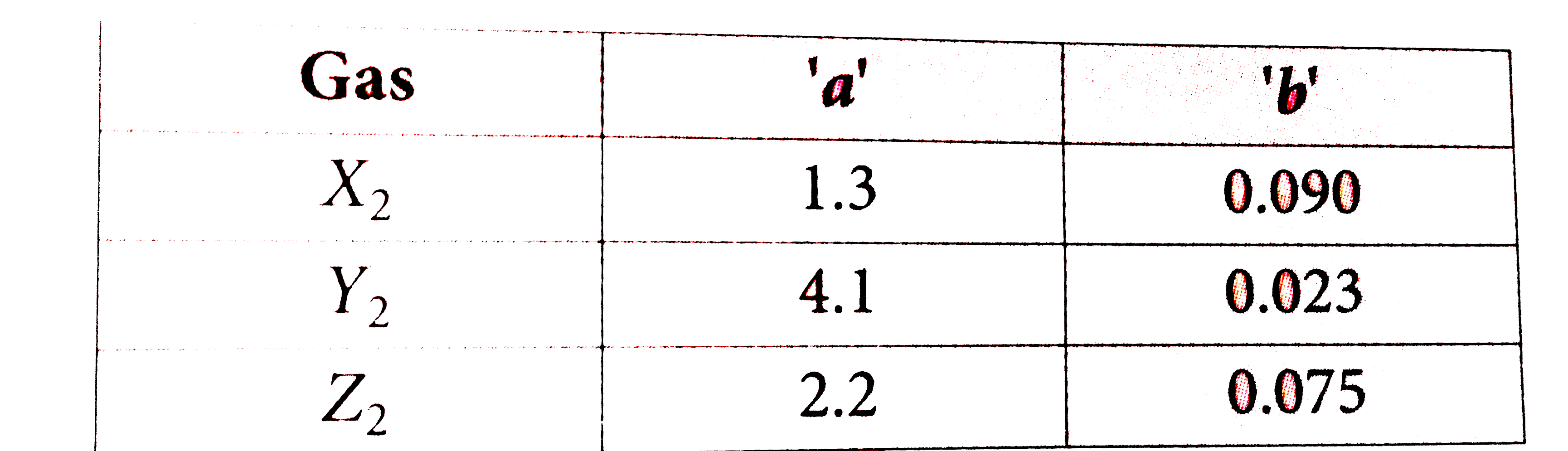
View SolutionThe values of van der Waals constant 'a' for a gas is zero. What does it signify ?
View SolutionThe values of van der Waals constant a for a gas is zero. What does it signify ?
View Solution

