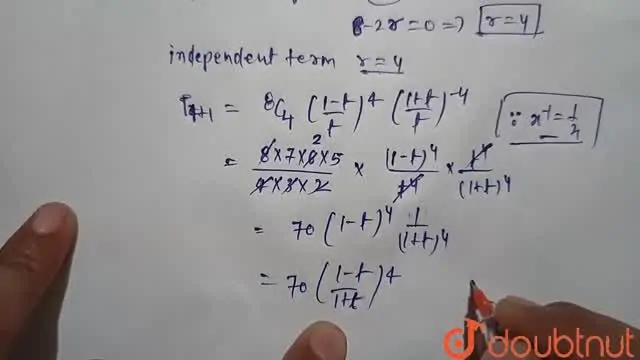
The the term independent in the expansion of [(t−1−1)x+(t−1+1)−1x−1]8 is
56(1−t1+t)3
56(1+t1−t)3
70(1−t1+t)4
70(1+t1−t)4
The correct Answer is:C
Step by step video & image solution for The the term independent in the expansion of [(t^(-1)-1)x+(t^(-1)+1)^(-1)x^(-1)]^8 is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - VMC MODULES ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - LEVEL 250videos
BINOMIAL THEOREM
- BOOK - VMC MODULES ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - NUMERICAL VALUE TYPE FOR JEE MAIN14videos
BINOMIAL THEOREM
- BOOK - VMC MODULES ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - JEE Archive56videos
BINOMIAL THEOREM
- BOOK - VMC MODULES ENGLISHCHAPTER - CIRCLESEXERCISE - JEE ADVANCED ( ARCHIVE )68videos
CIRCLES
Similar Questions
The term independent of x in the expansion of (2x−1x)10 is
View SolutionFind the term independent of x in the expansion of: (x−1x)12 .
View SolutionThe term independent ofx in the expansion of (1+x)10⋅(1+1x)10 is
View SolutionThe term independent of x in the expansion of (x2−1x)6 is
View SolutionThe term independent of x in the expansion of (2x+13x)6 is
View SolutionTerm independent of x in the expansion of (3x2−13x)9.
View SolutionFind the term independent of x in the expansion of (3x2−12x3)10 .
View SolutionThe term independent of x in the expansion of (1+x)n(1+1x)n is
View SolutionFind the term independent of x in the expansion of [12x1/3+x−1/5]8
View SolutionFind the terms independent of x in the expansion of (√x+13x2)10
View Solution