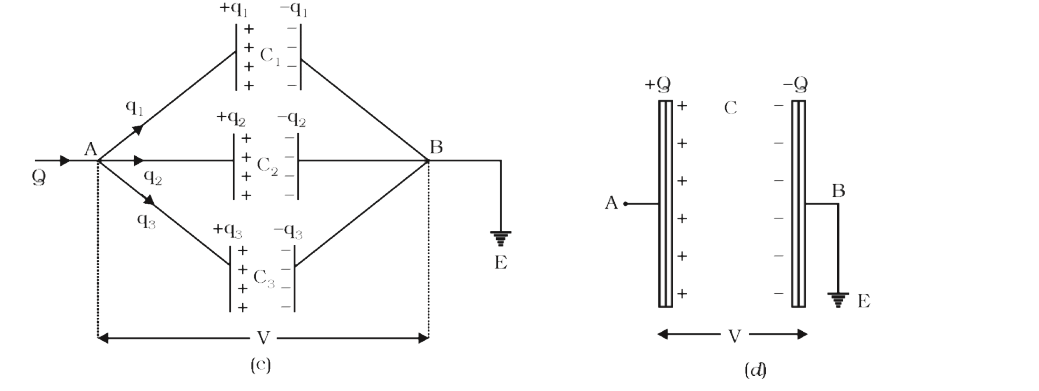
Deduce an expression for equivalent capacitance C when three capacitors C1,C2andC3 are connected in parallel.
In figure (a) three capacitors of capacitances C1,C2,C3 are connected in series between point A and D.
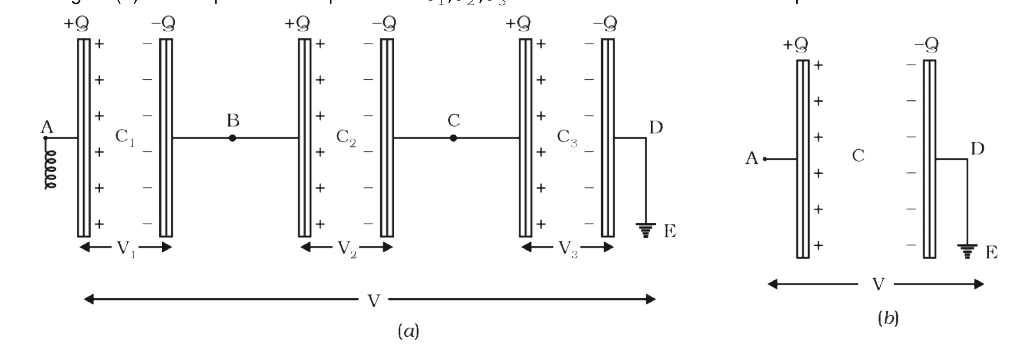
In series first plate of each capacitor has charge + Q and second plate of each capacitor has change –Q i.e,, charge on each capacitor is Q .
Let the potential differences across the capacitors C1,C2,C3 be V1,V2,V3 respectively. As the second plate of first capacitors C1 and first plate of second capacitor C2 are connected together, their potentials are equal. Let this common potential be VB. Similarly the common potential of second plate of C2 and first plate of C3 is VC. the second plate of capacitor C3 is connected to each , therefore its potential As charge flows from higher potential to lower potential, therefore, VA>VB>CV>VD.
For first capacitor, V1=VA−VB=QC1 . .. (i)
For second capacitor, V2=VB−VC=QC20 . . (ii)
For this capacitor, V3=VC−VD=QC3 . . (iii)
Addition equations (i), (ii), (iii) we get V1+V2+V3=VA−VD=[1C1+1C2+1C3]Q . . (iv)
If V be the potential difference betweenn A and D, then VA−VD=V
∴From (iv), we get V=(V1+V2+V3)=Q[1C1+1C2+1C3] . . (v)
If in place of all the three capacitors, only one capacitor is placed between A and D such that on giving it charge Q, the potential difference between its plates become V, then it will be called equivalent capacitor. If its capacitance is C, then
V=QC . . (vi)
Comparing (v) and (vi) we get QC=Q[1C1+1C2+1C3]
or 1C=1C1+1C2+1C3 . . (vii)
Thus in series arrangement, “The reciprocal of equivalent capacitance is equal to the sum of the reciprocals of the individual capacitors.”
Parallel Arrament: in fig (c) three
Topper's Solved these Questions
- CAPACITORSBook:VMC MODULES ENGLISHChapter:CAPACITORSExercise:LEVEL 1 (MCQs)
Explore 60 Videos
- CAPACITORSBook:VMC MODULES ENGLISHChapter:CAPACITORSExercise:LEVEL 2
Explore 40 Videos
- CAPACITORSBook:VMC MODULES ENGLISHChapter:CAPACITORSExercise:LEVEL 0 (Short Answer Type)
Explore 19 Videos
- BASIC MATHEMATICS & VECTORSBook:VMC MODULES ENGLISHChapter:BASIC MATHEMATICS & VECTORSExercise:Impeccable
Explore 50 Videos
- CURRENT ELECTRICITYBook:VMC MODULES ENGLISHChapter:CURRENT ELECTRICITYExercise:IN-CHAPTER EXERCISE-F
Explore 10 Videos
Similar Questions
Obtain an expression for equivalent capacitance when three capacitors C1,C2andC3 are connected in series.
View Solution(a) State the underlying principle of a potentiometer. Why is it necessary to
(i) use a long wire,
(ii) have uniform area of cross-section of the wire ?
(b) Obtain an expression for equivalent capacitance when three capacitors C1,C2andC3 are connected in series.
View SolutionThe equivalent capacitance of three capacitors of capacitance C1:C2 and C3 are connected in parallel is 12 units and product C1.C2.C3=48. When the capacitors C1 and C2 are connected in parallel, the equivalent capacitance is 6 units. then the capacitance are
View SolutionThree capacitors each of capacitance C are connected in series. Their equivalent capacitance is Cs. The same three capacitors are now connected in parallel. Their equivalent capacitance becomes Cp. Find the ratio (CpCs)
(Working must be shown).
View SolutionFour capacitors of equal capacitance have an equivalent capacitance C1 when connected in series and an equivalent capacitance C2 when connected in parallel. The ratio C1C2 is
View SolutionTwo capacitors with capacitances C1andC2 are connected in parallel. Find the resultant capacitance.
View SolutionThe effective capacitance of two capacitors of capacitances C1 and C2(C2>C1) connected in parallel is 256 times the effective capacitance when they are connected in series. The ratio C2C1 is
View SolutionFind the equivalent capacitance between A and B. All the capacitors have capacitance C.

View SolutionFind the equivalent capacitance between A and B. All the capacitors have capacitance C.

View SolutionThere are 7 identical capacitors. The equivalent capacitance when they are connected in series is C. The equivalent capacitance when they are connected in parallel is
View Solution