A police car with a siren of frequency 8KHz is moving with uniform velocity 36Km/hr towards a ball building which reflects the sound waves. The speed of sound in air is 320m/s. The frequency of the siren heard by the car driver is
8.50kHz
8.25kHz
7.75kHz
7.50kHz
The correct Answer is:A
To solve the problem, we will use the Doppler effect formula to find the frequency of the siren heard by the car driver. Here’s a step-by-step breakdown of the solution:
Step 1: Identify the Given Values
- Frequency of the siren (source frequency), f0=8kHz=8000Hz
- Speed of the police car (source), vs=36km/hr
- Speed of sound in air, c=320m/s
Step 2: Convert the Speed of the Police Car
Convert the speed from km/hr to m/s:
vs=36km/hr×1000m1km×1hr3600s=10m/s
Step 3: Calculate the Apparent Frequency at the Building
When the police car approaches the building, the frequency heard by the building (which is stationary) can be calculated using the formula:
f′=f0cc−vs
Substituting the values:
f′=8000Hz⋅320m/s320m/s−10m/s=8000⋅320310
Step 4: Calculate the Frequency
Now, calculate f′:
f′=8000⋅320310≈8000⋅1.03226≈8260.65Hz
Step 5: Calculate the Frequency Heard by the Driver
Now, the reflected sound wave will be heard by the driver. The driver is now the observer moving towards the building. The formula for the frequency heard by the driver is:
f′′=f′c+voc
Where vo is the speed of the observer (the driver), which is also 10m/s:
f′′=8260.65⋅320+10320=8260.65⋅330320
Step 6: Calculate the Final Frequency
Now, calculate f′′:
f′′=8260.65⋅1.03125≈8530.65Hz≈8.53kHz
Conclusion
The frequency of the siren heard by the car driver is approximately 8.53 kHz.
---
- Frequency of the siren (source frequency), f0=8kHz=8000Hz
- Speed of the police car (source), vs=36km/hr
- Speed of sound in air, c=320m/s
Convert the speed from km/hr to m/s:
vs=36km/hr×1000m1km×1hr3600s=10m/s
When the police car approaches the building, the frequency heard by the building (which is stationary) can be calculated using the formula:
f′=f0cc−vs
Substituting the values:
f′=8000Hz⋅320m/s320m/s−10m/s=8000⋅320310
Now, calculate f′:
f′=8000⋅320310≈8000⋅1.03226≈8260.65Hz
Now, the reflected sound wave will be heard by the driver. The driver is now the observer moving towards the building. The formula for the frequency heard by the driver is:
f′′=f′c+voc
Where vo is the speed of the observer (the driver), which is also 10m/s:
f′′=8260.65⋅320+10320=8260.65⋅330320
Now, calculate f′′:
f′′=8260.65⋅1.03125≈8530.65Hz≈8.53kHz
The frequency of the siren heard by the car driver is approximately 8.53 kHz.
Topper's Solved these Questions
- WAVE MOTIONBook:VMC MODULES ENGLISHChapter:WAVE MOTIONExercise:JEE ADVANCED ARCHIVE LEVEL 2 (MUTIPLE CORRECT TYPE)
Explore 20 Videos
- WAVE MOTIONBook:VMC MODULES ENGLISHChapter:WAVE MOTIONExercise:JEE ADVANCED ARCHIVE LEVEL 2 (PARAGRAPH TYPE)
Explore 6 Videos
- WAVE MOTIONBook:VMC MODULES ENGLISHChapter:WAVE MOTIONExercise:JEE MAIN ARCHIVE LEVEL 1
Explore 56 Videos
- UNITS, MEASUREMENTS & ERRORSBook:VMC MODULES ENGLISHChapter:UNITS, MEASUREMENTS & ERRORSExercise:IN - CHAPTER EXERCISE - B
Explore 10 Videos
- WORK ENERGY AND POWER Book:VMC MODULES ENGLISHChapter:WORK ENERGY AND POWER Exercise:IMPECCABLE
Explore 54 Videos
Similar Questions
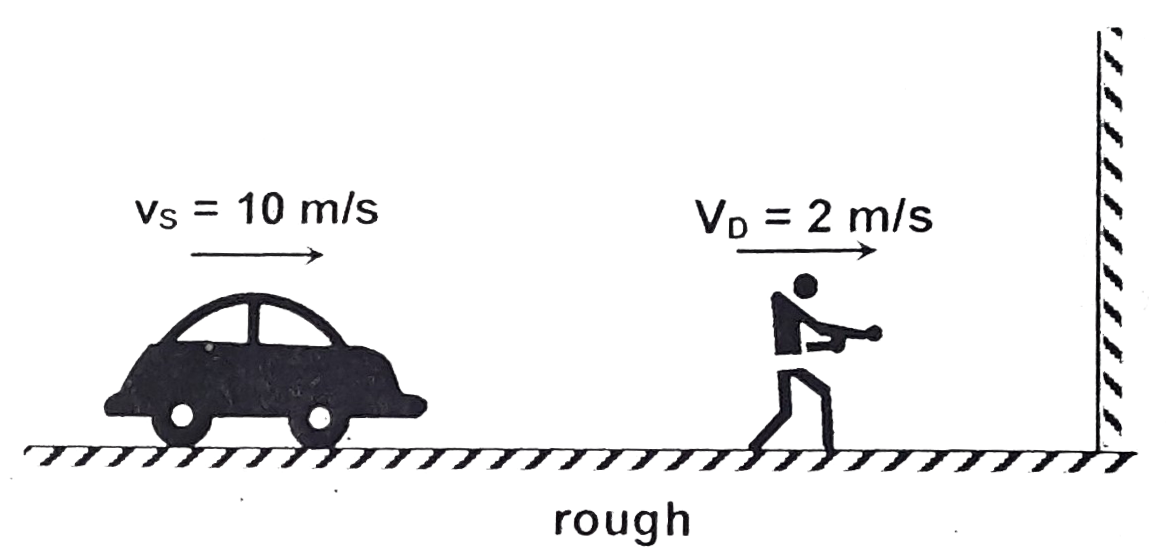
A car is moving with a constant velocity 10m/s towards the stationary wall. The car blows a horn of frequency 320Hz. The velocity of sound in air is 330m/s. The beats frequency received by the observer is
View Solutiona bus is moving with a velocity 4 m/s towards a wall, the driver sounds a horn of frequency 173 Hz . if the speed of sound in air is 350 m/s. the number of beat heard per second by a passanger on the bus will be
View SolutionThe driver of a car travelling with speed 30ms−1 towards a hill sounds a horn of frequency 600 Hz. If the velocity of sound in air is 330ms−1, the frequency of reflected sound as heard by driver is
View SolutionA car blowing a horn of frequency 350 Hz is moving normally towards a wall a speed of 5 m/s The beat frequency heard by a person standing between the car and wall is (speed of sound in air =350 m/s )
View SolutionA source of sound of frequency 500 Hz is moving towards an observer with velocity 30 m/s . The speed of sound is 330 m/s . the frequency heard by the observer will be
View SolutionAn engine blowing a whistle of frequency 133 Hz moves with a velocity of 60ms towards a wall from which an echo is heard. Calculate the frequency of the echo heard by the driver. (velocity of sound in air in 340ms.)
View SolutionA car travels at a speed of 'a' towards a high wall. The driver sounds a horn of frequency 'n'. If V is the velocity of sound in air, frequency of reflected sound heard by the driver is
View SolutionAn observer is standing 500 m away from a vertical hill. Starting between the observer and the hill, a police van sounding a siren of frequency 1000 Hz moves towards the hill with a uniform speed. If the frequency of the sound heard directly from the siren is 970 Hz, the frequency of the sound heard after reflection from the hill (in Hz) is about,(velocity of sound = 330ms−1
View SolutionAn engine blowing a whistle of frequency 133 Hz moves with a velocity of 60ms towards a hill from which an echo is heard. Calculate the frequency of the echo heard by the driver. (velocity of sound in air in 340ms.)
View SolutionA car B, with a hom of frequency 700 Hz moves with a velocity 90kmh−1. It approaches another car A, coming towards it with a velocity 72kmh−1. Calculate the apparent frequency of the sound heard by the driver in the car A (a) before crossing (b) after crossing ? Velocity of sound =350ms−1.
View Solution