A motorcycle starts from rest and accelerates along a straight line at 2.2ms2. The speed of sound is 330ms. A siren at the starting point remains stationary. When the driver hears the frequency of the siren at 90% of when motorcycle is stationary, the distance travelled by the motorcyclist is
123.75 m
247.5 m
495m
990m
The correct Answer is:B
To solve the problem step by step, we will use the Doppler effect and the equations of motion.
Step 1: Understand the Doppler Effect
The frequency heard by the observer (motorcyclist) is affected by their motion relative to the source (siren). The formula for the observed frequency n′ is given by:
n′=nv+u0v−us
Where:
- n′ = observed frequency
- n = emitted frequency
- v = speed of sound
- u0 = speed of the observer (motorcyclist)
- us = speed of the source (siren)
In this case, the siren is stationary, so us=0.
Step 2: Set Up the Equation
We know that the motorcyclist hears the frequency at 90% of the stationary frequency:
n′=0.9n
Substituting this into the Doppler effect equation gives:
0.9n=nv+u0v
Step 3: Simplify the Equation
Dividing both sides by n (assuming n≠0):
0.9=v+u0v
Rearranging gives:
0.9v=v+u0
Thus,
u0=0.9v−v=−0.1v
Since the motorcycle is moving away from the siren, we take the positive value:
u0=0.1v
Step 4: Substitute the Speed of Sound
Given that the speed of sound v=330m/s:
u0=0.1×330=33m/s
Step 5: Use the Equation of Motion
Now we can use the equation of motion to find the distance s traveled by the motorcycle. The equation is:
v2=u2+2as
Where:
- v = final velocity
- u = initial velocity (0, since it starts from rest)
- a = acceleration (given as 2.2m/s2)
Substituting the known values:
(33)2=0+2×2.2×s
Step 6: Solve for s
Calculating 332:
1089=4.4s
Now, solving for s:
s=10894.4≈247.5m
Final Answer
The distance traveled by the motorcyclist is approximately 247.5 meters.
---
The frequency heard by the observer (motorcyclist) is affected by their motion relative to the source (siren). The formula for the observed frequency n′ is given by:
- n′ = observed frequency
- n = emitted frequency
- v = speed of sound
- u0 = speed of the observer (motorcyclist)
- us = speed of the source (siren)
We know that the motorcyclist hears the frequency at 90% of the stationary frequency:
Dividing both sides by n (assuming n≠0):
Given that the speed of sound v=330m/s:
Now we can use the equation of motion to find the distance s traveled by the motorcycle. The equation is:
- v = final velocity
- u = initial velocity (0, since it starts from rest)
- a = acceleration (given as 2.2m/s2)
Calculating 332:
The distance traveled by the motorcyclist is approximately 247.5 meters.
Topper's Solved these Questions
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - LEVEL-2
41 Videos
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE MAIN ARCHIVE LEVEL 1
56 Videos
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE ADVANCED ARCHIVE LEVEL 2 (TRUE FALSE TYPE)
4 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - IN - CHAPTER EXERCISE - B
10 Videos
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - IMPECCABLE
54 Videos
Similar Questions
A motor cycle starts from rest and accelerates along a straight path at 2m/s2. At the starting point of the motor cycle there is a stationary electric siren. How far has the motor cycle gone when the driver hears the frequency of the siren at 94% of its value when the motor cycle was at rest ? (Speed of sound = 330ms−2)
View SolutionA motor cycle starts from rest and accelerates along a straight path at 2m/s2. At the starting point of the motor cycle there is a stationary electric siren. How far has the motor cycle gone when the driver hears the frequency of the siren at 94% of its value when the motor cycle was at rest ? (Speed of sound = 330ms−2)
View Solution
Knowledge Check
How long would it take a car, starting from rest and accelerating uniformly in a straight line at 5m/s2, to cover a distance of 200 m ?
A9.0 sB10.5 sC12.0 sD15.5 s
A motor cycle starts from rest and accelerates along a straight path at 2m/s2. At the straight point of the motor cycle there is a stationary electric siren. How far has the motor cycle gone when the driver hears the frequency of the siren at 94% of its value when the motor cycle was al rest? (Speed of sound = 330ms−1)
View SolutionA motorboat starting from rest on a lake accelerates in a straight line at a constant rate of 3.0m/s2 for 8.0s. How far does the boat travel during this time ?
View SolutionA body starts from rest and accelerates with 4m/s2 for 5 seconds. Find the distance travelled with 5 seconds.
View SolutionThe acceleration of a particle starting from rest and travelling along a straight line is shown in figure. The maximum speed of the particle is
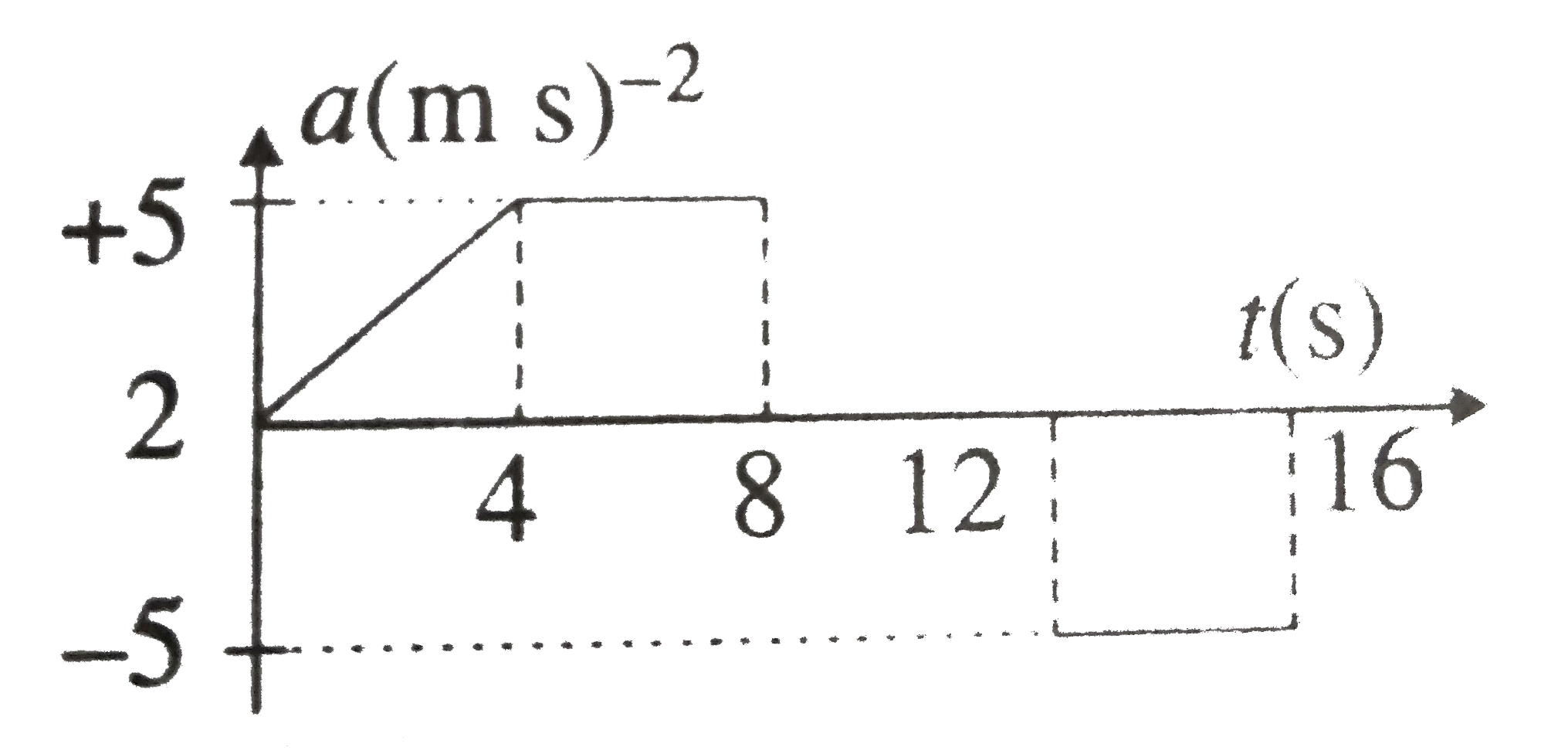
View SolutionA particle starts motion from rest and moves along a straight line. Its acceleration-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View SolutionA bus starts from rest and moves whith acceleration a=2ms2. The ratio of the distance covered in 6th second to that covered in 6 seconds is
View SolutionA body starting from rest moves along a straight line with a constant acceleration. The variation of speed (v) with distance (s) is represented by the graph:
View Solution
