One mole of and ideal monoatomic gas is compressed isothermally in a rigid vessel to double its pressure at room temperature, 27∘C . The work done on the gas will be :
300R
300RIn6
300RIn2
300RIn7
The correct Answer is:C
W=nRTln(Vf)Vi=300ln2
Topper's Solved these Questions
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )
111 Videos
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - Level - 2
40 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
1 Videos
- GRAVITATIONBOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE
1 Videos
Similar Questions
When an ideal gas is compressed isothermally then its pressure increase because:
View SolutionOne mole of a gas is heated at constant pressure to raise its temperature by 1∘C. The work done in joules is
View SolutionIf one mole of gas doubles its volume at temperature T isothermally then work done by the gas is
View SolutionAt 27∘C, one mole of an ideal gas is compressed isothermally and reversibly from a pressure of 2 atm to 10 atm. Calculate ΔU and q.
View SolutionOne mole of an ideal and monoatomic gas is expanded reversibly and isothermally at 298K temperature according to the given diagram, calculate work done.
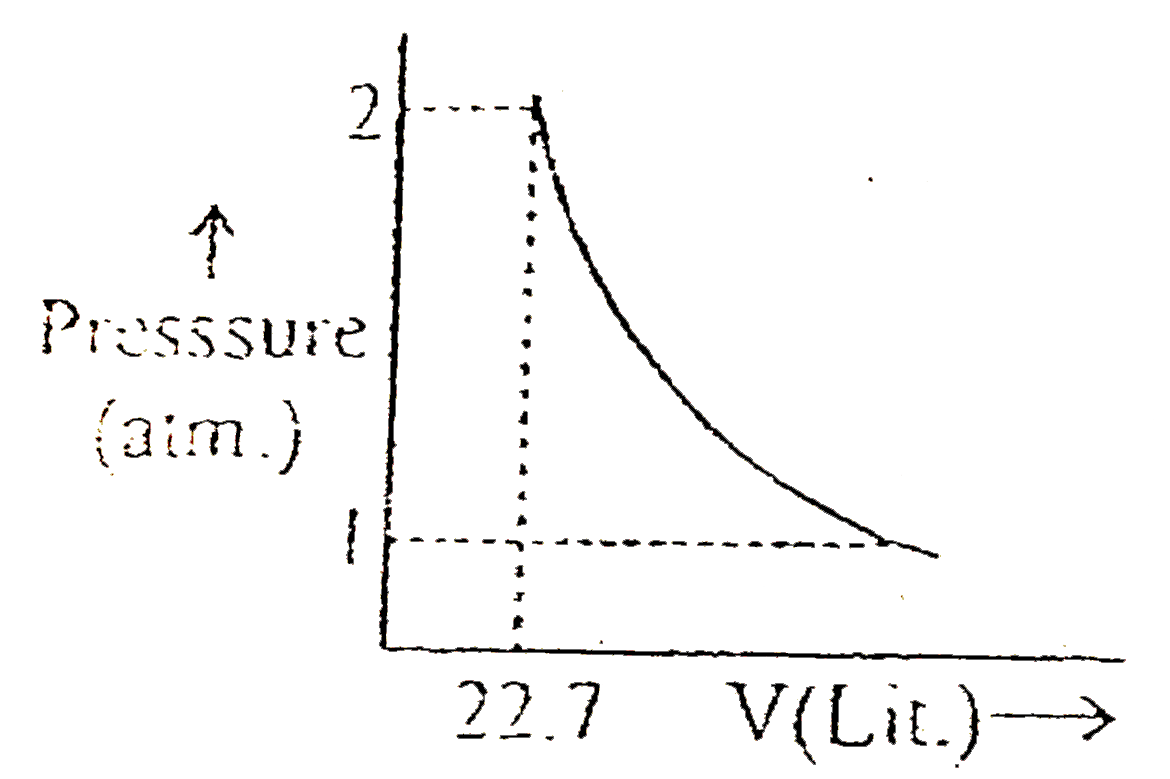
View SolutionAn ideal gas at 300K is expanded isothermally to double its volume,then pressure of the gas will be
View SolutionOne gm mol of a diatomic gas (γ=1.4) is compressed adiabatically so that its temperature rises from 27∘C to 127∘C . The work done will be
View SolutionOne gm mol of a diatomic gas (γ=1.4) is compressed adiabatically so that its temperature rises from 27∘C to 127∘C . The work done will be
View SolutionTwo moles of an ideal monatomic gas is heated at constant pressure so that its temperature increases from 127∘C to 227∘C , then work done by gas
View SolutionA monoatomic gas is compressed adiabatically to 8/27 of its initial volume, if initial temperature is 27∘C, then increase in temperature
View Solution
