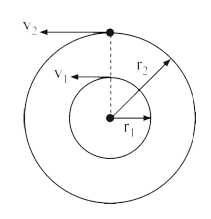
Two satellite revolve round a planet in coplanar circular orbits in the same sense. Their periods of revolutions are 1 h nd 8 h respectively. The radius of the orbit of is . When is closet to ., find (a). The speed of relative to and (b). the angular speed of as observed by an astronaut in .
The correct Answer is:(i)4 (ii
(i) According to Kepler’s third law
km
The speed of satellite km/hr
The speed of satellite
The speed of satellites relative to .
The speed of satellite
The speed of satellites relative to .
km/hr
(ii) The angular speed of satellite relative to
rad/hr
Related Playlists
- BOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) FILL IN THE BLANKS TYPE6videos
GRAVITATION
- BOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE1videos
GRAVITATION
- BOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive)MATCH MATRIX1videos
GRAVITATION
- BOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )111videos
GASEOUS STATE & THERMODYNAMICS
- BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Advanced ( ARCHIVE LEVEL-2)12videos
INTRODUCTION TO VECTORS & FORCES
Knowledge Check
- Question 1 - Select One
Two satellites S1 and S2 revolve around a planet in coplanar circular orbits in the same sense their periods of revolution are 1 hour and 8hours respectively the radius of the orbit of S1 is 104 km when S1 is closest to S2 the angular speed of S2 as observed by an astronaut in S1 is :
Aπ×104B−π×104Cπ×105Dπ×105 - Question 1 - Select One
In the above example the angular velocity of S2 as actually observed by an astronaut in S1 is -
A3×10−4B3×1023C3×10−6D3×10−7
Similar Questions
Two satellites S1 and S2 revolve round a planet in coplaner circular orbit in the same sense. Their period of revolution are 1 hour and 8 hour respectively. The radius of the orbit of S1 is 104km. When S2 is closest to S1, find
(a) The speed of S2 relative to S1,
(b) The angular speed of S2 actually observed by an astronaut is S1View SolutionTwo satellites S1 and S2 are revolving round a planet in coplanar and concentric circular orbit of radii R1 and R2 in te same direction respectively. Their respective periods of revolution are 1 hr and 8 hr. the radius of the orbit of satellite S1 is equal to 104km. Find the relative speed in kmph when they are closest.
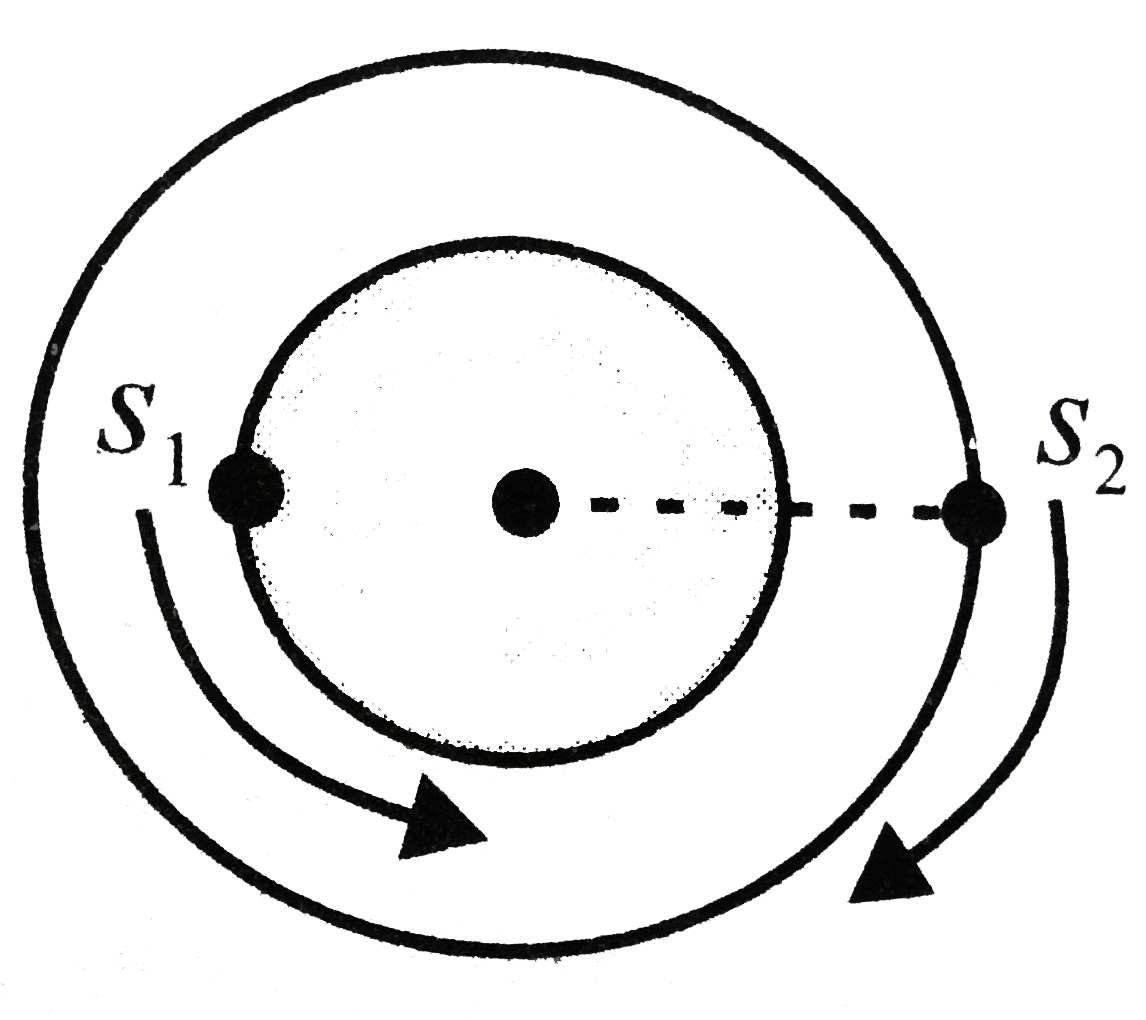
View SolutionTwo satellites S1 and S2 are revolving around the earth in coplanar concentric orbits in the opposite sense. At t=0, the position of satellites are shown in the diagram. The periods of S1 and S2 are 4h and 24h, respectively. The radius of orbit of S1 is 1.28×104km. For this situation, mark the correct statement (s).
 View Solution
View SolutionTwo satellities s1 a & s2 of equal masses revolves in the same sense around a heavy planet in coplaner circular orbit of radii R & 4R
View SolutionTwo satellites S1 and S2 revolve around the earth at distances 3R and 6R from the centre of the earth. Find the ratio of their (a) linear speeds and (b) angular speeds.
View SolutionA satellite is revolving round the earth in a circular orbit with a velocity of 8km/s. at a height where acceleration due to gravity is 8m/s2. How high is the satellite from the earth ? (Take R = 6000 km)
View SolutionThe Velocity of four gas molecules are given by 1 km/s, 2 km/s, 6 km/s and 8 km/s respectively. The rms velocity is
View SolutionOrbital radius of a satellite S of earth is four times that s communication satellite C. Period of revolution of S is :-
View Solution

