A railway carriage moves over a straight track with acceleration a. Passenger in the carriage drops a stone. What is the acceleration of the stone w.r.t. the carriage and the earth?
To solve the problem, we need to analyze the motion of the stone dropped from the railway carriage in two different frames of reference: the Earth and the carriage itself.
1. Identify the Acceleration of the Carriage:
The railway carriage is moving with a constant acceleration a in a horizontal direction.
2. Identify the Acceleration of the Stone:
When the stone is dropped from the carriage, it will start to fall freely under the influence of gravity. The acceleration of the stone due to gravity is g (approximately 9.81m/s2) acting downward.
3. Acceleration of the Stone with Respect to the Earth:
Since the stone is in free fall, its acceleration with respect to the Earth is simply g downward. Therefore, we can write:
astone, Earth=g(downward)
4. Acceleration of the Stone with Respect to the Carriage:
To find the acceleration of the stone with respect to the carriage, we need to consider the acceleration of both the stone and the carriage. The stone has an acceleration of g downward, while the carriage has an acceleration of a horizontally.
The acceleration of the stone with respect to the carriage can be calculated using the formula:
astone, carriage=astone−acarriage
Here, astone is g downward and acarriage is a in the horizontal direction.
5. Vector Representation:
Since the two accelerations are perpendicular (one is vertical and the other is horizontal), we can use the Pythagorean theorem to find the resultant acceleration of the stone with respect to the carriage:
astone, carriage=√g2+a2
6. Conclusion:
- The acceleration of the stone with respect to the Earth is g(downward).
- The acceleration of the stone with respect to the carriage is √g2+a2.
Summary:
- Acceleration of the stone with respect to the Earth: g(downward)
- Acceleration of the stone with respect to the carriage: √g2+a2
The railway carriage is moving with a constant acceleration a in a horizontal direction.
When the stone is dropped from the carriage, it will start to fall freely under the influence of gravity. The acceleration of the stone due to gravity is g (approximately 9.81m/s2) acting downward.
Since the stone is in free fall, its acceleration with respect to the Earth is simply g downward. Therefore, we can write:
astone, Earth=g(downward)
To find the acceleration of the stone with respect to the carriage, we need to consider the acceleration of both the stone and the carriage. The stone has an acceleration of g downward, while the carriage has an acceleration of a horizontally.
astone, carriage=astone−acarriage
Here, astone is g downward and acarriage is a in the horizontal direction.
Since the two accelerations are perpendicular (one is vertical and the other is horizontal), we can use the Pythagorean theorem to find the resultant acceleration of the stone with respect to the carriage:
astone, carriage=√g2+a2
- The acceleration of the stone with respect to the Earth is g(downward).
- The acceleration of the stone with respect to the carriage is √g2+a2.
- Acceleration of the stone with respect to the Earth: g(downward)
- Acceleration of the stone with respect to the carriage: √g2+a2
Topper's Solved these Questions
- Motion in Two DimensionsBook:VMC MODULES ENGLISHChapter:Motion in Two DimensionsExercise:Long Answer Type
Explore 5 Videos
- Motion in Two DimensionsBook:VMC MODULES ENGLISHChapter:Motion in Two DimensionsExercise:Level -1
Explore 140 Videos
- Motion in Straight Line Book:VMC MODULES ENGLISHChapter:Motion in Straight Line Exercise:IN-CHAPTER EXERCISE-J
Explore 10 Videos
- MOVING CHARGES & MAGNETISM Book:VMC MODULES ENGLISHChapter:MOVING CHARGES & MAGNETISM Exercise:IN-CHAPTER EXERCISE-K
Explore 10 Videos
Similar Questions
A bus moves over a straight level road with a constant acceleration a. A boy in the bus drops a ball out side. The acceleration of the ball w.r.t the bus and the earth are respectively
View SolutionA train is moving forward with a horizontal acceleration a. A man sitting in the train drops a coin on the floor of the train . The acceleration of the coin w.r.t man is
View SolutionA stone is released from an elevator going up with an acceleration of g2. What is the acceleration of the stone just after release ?
View SolutionIf block A is moving with an acceleration of 5ms−2, the acceleration of B w.r.t. ground is
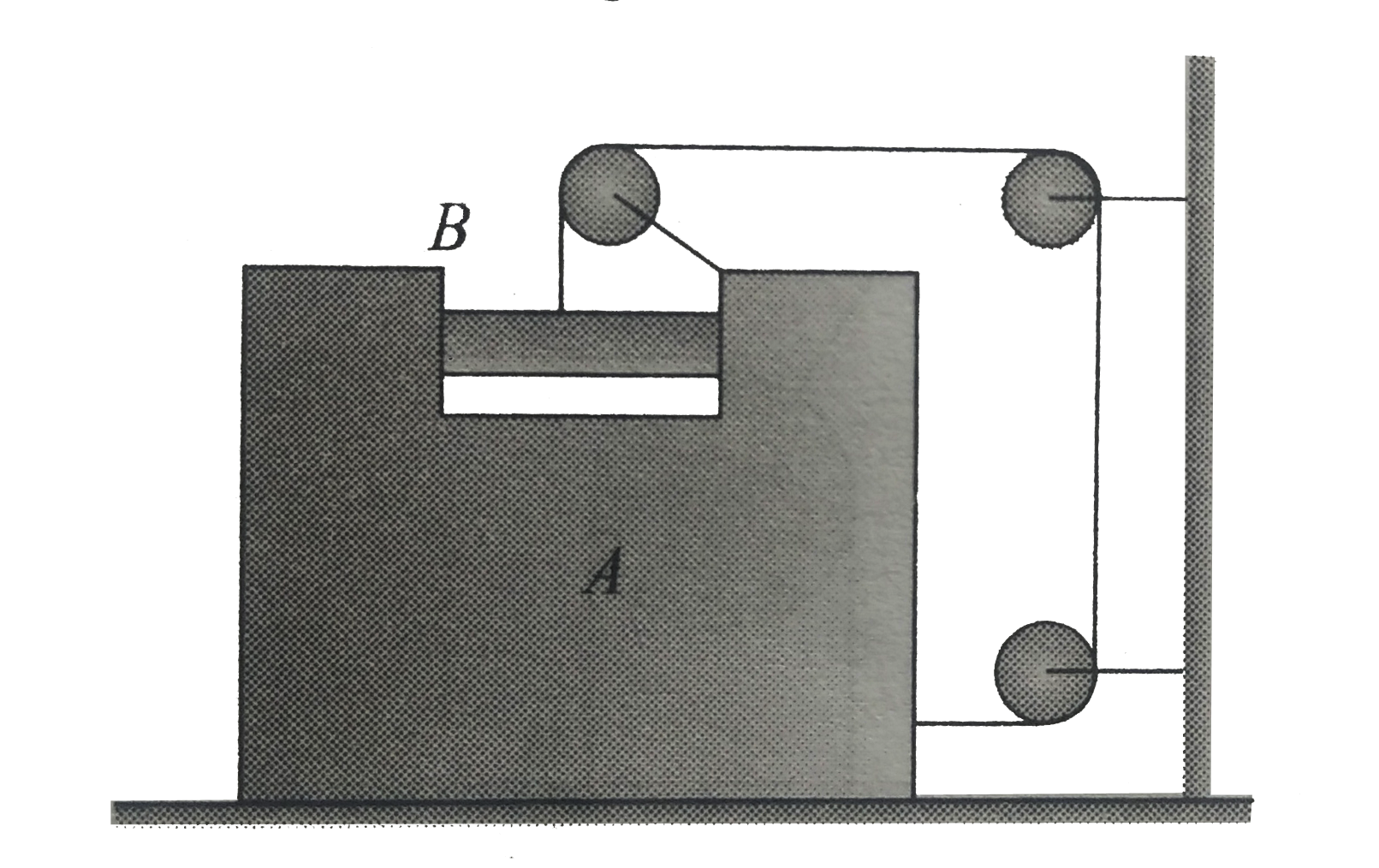
View SolutionA body moving in a straight line with constant acceleration covers distances a and b in successive equal time interval of t. The acceleration of the body is
View SolutionA particle moves in a straight line so that s=√t, then its acceleration is proportional to
View SolutionA particle moves in a straight line so that s=√t, then its acceleration is proportional to
View Solution_S01_017_Q01.png)
An open water tanker moving on a horizontal straight road has a cubical block of cork floating over its surface if the tanker has an acceleration of a as shown, the acceleration of the cork w.r.t container is ignore viscosity.
View Solution_S01_017_Q01.png)
An open water tanker moving on a horizontal straight road has a cubical block of cork floating over its surface if the tanker has an acceleration of a as shown, the acceleration of the cork w.r.t container is ignore viscosity.
View SolutionA piece of stone tied at the end of a thread is whirled in a horizontal circle with uniform speed by hand, Answer the following questions
(a) Is the velocity of stone uniform or variable?
(b ) Is the acceleration of stone uniform or variable?
( c) What is the direction of acceleration of stone at any instant?
(d) which force provides the centripetal force required for circular motion?
(e) Name the force and its direction which acts on the hand.
View Solution