A metal (M∘=100 gm/mole) having density 10 gm/cm3 forms a cubic lattice. If radius of an atom is 1.4 Å find packing fraction. [NA=6×1023 perm ole].
0.74
0.69
0.52
0.90
To find the packing fraction of the metal, we will follow these steps:
Step 1: Determine the number of atoms per unit cell (Z)
For a simple cubic lattice, there is 1 atom per unit cell. This is because there are 8 corners in a cube, and each corner atom is shared by 8 unit cells. Therefore, the contribution from the corners is:
Z=8×18=1
Hint: Remember that for a simple cubic lattice, the number of atoms per unit cell is calculated based on the contribution of atoms at the corners.
Step 2: Calculate the volume of one atom
The volume of one atom can be calculated using the formula for the volume of a sphere:
Vatom=43πr3
Given the radius r=1.4Å=1.4×10−8cm:
Vatom=43π(1.4×10−8)3
Hint: Use the formula for the volume of a sphere and ensure to convert the radius to the correct units.
Step 3: Calculate the volume of the unit cell
For a simple cubic lattice, the edge length a is related to the radius r by:
a=2r
Thus, the volume of the unit cell is:
Vunit cell=a3=(2r)3=8r3
Hint: Remember that the edge length of a simple cubic unit cell is twice the radius of the atom.
Step 4: Substitute values to find the packing fraction
The packing fraction PF is given by:
PF=Z×VatomVunit cell
Substituting the values we have:
PF=1×43πr38r3
This simplifies to:
PF=43π8=π6
Step 5: Calculate the numerical value of the packing fraction
Using π≈3.14:
PF≈3.146≈0.523
Thus, the packing fraction is approximately 0.52.
Final Answer:
The packing fraction of the metal is approximately 0.52.
---
For a simple cubic lattice, there is 1 atom per unit cell. This is because there are 8 corners in a cube, and each corner atom is shared by 8 unit cells. Therefore, the contribution from the corners is:
Z=8×18=1
The volume of one atom can be calculated using the formula for the volume of a sphere:
Vatom=43πr3
Given the radius r=1.4Å=1.4×10−8cm:
Vatom=43π(1.4×10−8)3
For a simple cubic lattice, the edge length a is related to the radius r by:
a=2r
Thus, the volume of the unit cell is:
Vunit cell=a3=(2r)3=8r3
The packing fraction PF is given by:
PF=Z×VatomVunit cell
Substituting the values we have:
PF=1×43πr38r3
This simplifies to:
PF=43π8=π6
Using π≈3.14:
PF≈3.146≈0.523
The packing fraction of the metal is approximately 0.52.
Topper's Solved these Questions
- THE SOLID STATEBook:VMC MODULES ENGLISHChapter:THE SOLID STATEExercise:LEVEL-2 (Numerical Value Type for JEE Main )
Explore 15 Videos
- THE SOLID STATEBook:VMC MODULES ENGLISHChapter:THE SOLID STATEExercise:JEE Main (Archive )
Explore 39 Videos
- THE SOLID STATEBook:VMC MODULES ENGLISHChapter:THE SOLID STATEExercise:LEVEL-1
Explore 75 Videos
- SURFACE CHEMISTRYBook:VMC MODULES ENGLISHChapter:SURFACE CHEMISTRYExercise:PRACTICE EXERCISE
Explore 9 Videos
- THEORY OF SOLUTIONSBook:VMC MODULES ENGLISHChapter:THEORY OF SOLUTIONSExercise:JEE Advanced (Archive)
Explore 31 Videos
Similar Questions
Knowledge Check
The number of atoms in 0.1 mole of a triatomic gas is (NA=6.02×1023mol−1)
A6.026×1022B1.806×1023C3.600×1023D1.800×1022
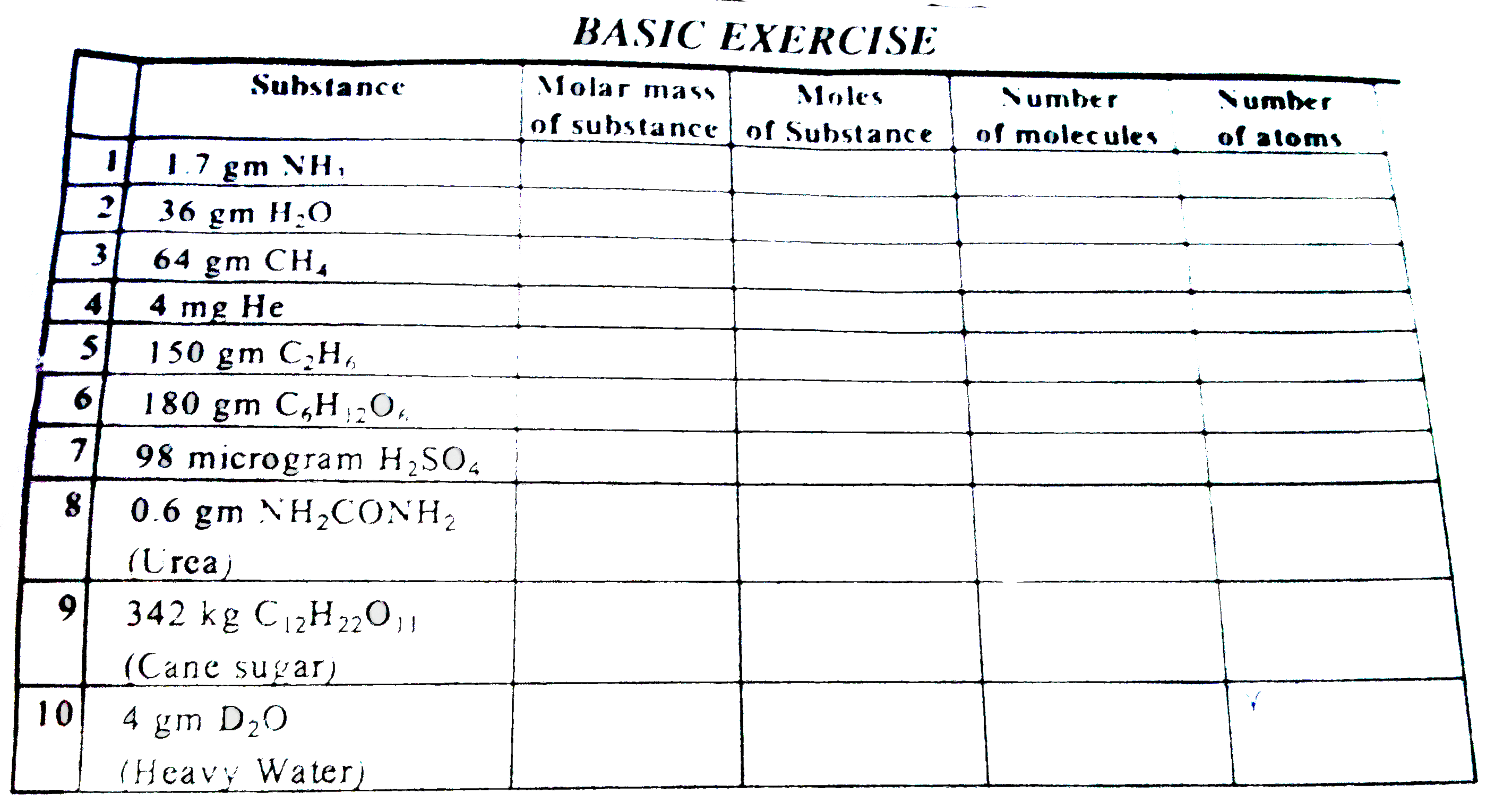
A box contains 12×1022 number of oxygen atoms. Find moles of O-atoms? (NA=6×1023)
View SolutionThe edge length of unit cell of a metal having molecular weight 75gmol−1 is 5Å which crystallizes in cubic lattice. If the density is 2gcm−3, then find the radius of metal atom (NA=6×1023). Give the answer in pm.
View SolutionAn element with density 11.2gcm−3 forms a f. c. c. lattice with edge length of 4×10−8 cm. Calculate the atomic mass of the element. (Given : NA=6.022×1023mol−1
View SolutionOne atom of an element X weighs 6×10−23gm. Number of moles of atoms in 3.6 kg of sample of X will be : (NA=6×1023)
View SolutionSilver crystallises in a fcc lattice. The edge length of its unit is 4.077×10−8cm and its density is 10.5gcm−3. Claculate on this basis of the atomic mass of silver (NA=6.02×1023mol−1)
View SolutionNiobium crystallizes in body centred cubic structure. If its density is 8.55 g cm−3, calculate the atomic radius of niobium. (Atomic mass of Nb = 93u , NA=6.02×1023mol−1)
View SolutionTotal number of ions in 17.1 g Al2(SO4)3 is [NA=6×1023]
View Solution