P is a point lying on line y = x then maximum value of , (where ) is
(a)
(b)
(c)
(d)
The correct Answer is:A
To find the maximum value of |PA−PB| where P is a point on the line y=x, and A=(1,3) and B=(5,2), we can follow these steps:
Step 1: Understand the problem
We need to find the maximum value of the expression |PA−PB|. Here, PA is the distance from point P to point A, and PB is the distance from point P to point B.
Step 2: Set the coordinates of point P
Since P lies on the line y=x, we can represent P as P(x,x).
Step 3: Calculate distances PA and PB
Using the distance formula, we can calculate the distances PA and PB:
- The distance PA from P(x,x) to A(1,3):
PA=√(x−1)2+(x−3)2
- The distance PB from P(x,x) to B(5,2):
PB=√(x−5)2+(x−2)2
Step 4: Simplify the expressions for PA and PB
Calculating PA:
PA=√(x−1)2+(x−3)2=√(x−1)2+(x2−6x+9)=√2x2−8x+10
Calculating PB:
PB=√(x−5)2+(x−2)2=√(x2−10x+25)+(x2−4x+4)=√2x2−14x+29
Step 5: Set up the expression for |PA−PB|
Now we want to maximize |PA−PB|:
|PA−PB|=∣∣√2x2−8x+10−√2x2−14x+29∣∣
Step 6: Use the triangle inequality
From the triangle inequality, we know that:
|PA−PB|≤AB
where AB is the distance between points A and B.
Step 7: Calculate the distance AB
Using the distance formula:
AB=√(5−1)2+(2−3)2=√42+(−1)2=√16+1=√17
Step 8: Conclusion
Thus, the maximum value of |PA−PB| is equal to the distance AB:
Maximum value of |PA−PB|=√17
Final Answer
The maximum value of |PA−PB| is √17.
---
We need to find the maximum value of the expression |PA−PB|. Here, PA is the distance from point P to point A, and PB is the distance from point P to point B.
Since P lies on the line y=x, we can represent P as P(x,x).
Using the distance formula, we can calculate the distances PA and PB:
PA=√(x−1)2+(x−3)2
- The distance PB from P(x,x) to B(5,2):
PB=√(x−5)2+(x−2)2
Calculating PA:
PA=√(x−1)2+(x−3)2=√(x−1)2+(x2−6x+9)=√2x2−8x+10
PB=√(x−5)2+(x−2)2=√(x2−10x+25)+(x2−4x+4)=√2x2−14x+29
Now we want to maximize |PA−PB|:
|PA−PB|=∣∣√2x2−8x+10−√2x2−14x+29∣∣
From the triangle inequality, we know that:
|PA−PB|≤AB
where AB is the distance between points A and B.
Using the distance formula:
AB=√(5−1)2+(2−3)2=√42+(−1)2=√16+1=√17
Thus, the maximum value of |PA−PB| is equal to the distance AB:
Maximum value of |PA−PB|=√17
The maximum value of |PA−PB| is √17.
Topper's Solved these Questions
- JEE MAIN REVISION TEST - 28Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISION TEST - 28Exercise:MATHEMATICS (SECTION-2)
Explore 5 Videos
- JEE MAIN REVISION TEST - 27 - JEE -2020Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISION TEST - 27 - JEE -2020Exercise:MATHEMATICS (SECTION 2)
Explore 5 Videos
- JEE MAIN REVISION TEST - 29 (2020)Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISION TEST - 29 (2020)Exercise:MATHEMATICS
Explore 25 Videos
Similar Questions
The co-ordinates of a point P on the line 2x−y+5=0 such that |PA−PB| is maximum where A is (4,−2)andB is (2,−4) will be
View SolutionFind a point P on the line 3x+2y+10=0 such that |PA−PB|is minimum where A is (4,2) and B is (2,4)
View SolutionFrom point P(4,0) tangents PA and PB are drawn to the circle S:x2+y2=4. If point Q lies on the circle, then maximum area of △QAB is- (1) 2√3 (2) 3√3 (3) 4√3 A) 9
View SolutionThe coordinates of the point P on the line 2x+3y+1=0 such that |PA−PB| is maximum where A is (2,0) and B is (0,2) is
View SolutionConsider the point A=(3,4),B(7,13). If 'P' be a point on the line y=x such that PA+PB is minimum then coordinates of P is (A) (137,13,7) (B) (237,237) (C) (317,317) (D) (337,337)
View SolutionIf the point M(h,k) lie on the line 2x+3y=5 such that |MA−MB| is maximum where (1, 2) and B(2, 3), then the value of (h+k) is
View SolutionConsider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
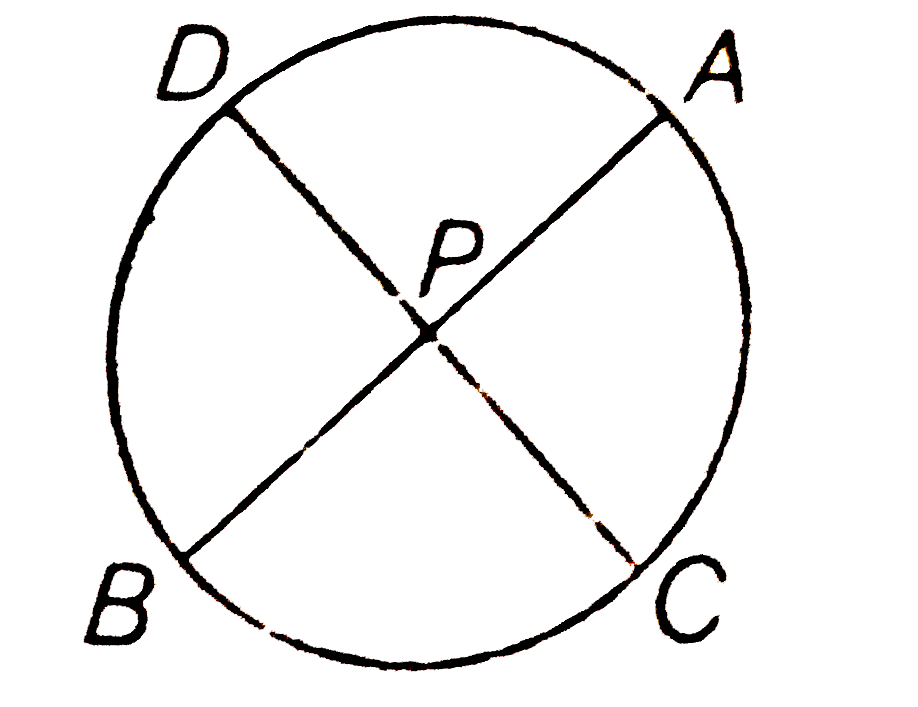
On the basis of above information , answer the questions
If PA=|cosθ+sinθ|andPB=|cosθ−sinθ|, then maximum value of (PC)(PD) , is equal to
View SolutionConsider the points A(0,1)andB(2,0),andP be a point on the line 4x+3y+9=0 . The coordinates of P such that |PA−PB| is maximum are (−245,175) (b) (−845,135) (317,317) (d) (0,0)
View SolutionStatement I Let A≡(0,1)andB≡(2,0) and P be a point on the line 4x+3y+9=0 then the co - ordinates of P such that |PA−PB|is maximum is (−125,175)
Statement II |PA−PB|≤|AB|
View SolutionA chord is drawn passing through P(2,2) on the ellipse x225+y216=1 such that it intersects the ellipse at A and B. Then maximum value of PA.PB is
View Solution