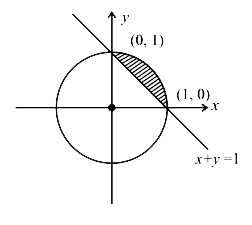
Find the area of the region {(x,y):x2+y2≤1≤x+y}
A
π24
B
π22
C
π4+12
D
π4−12
Video Solution
Text Solution
The correct Answer is:D
Area of shaded region = π(1)24−12(1)(1)=π4−12.
|
Topper's Solved these Questions
- JEE MAIN REVISION TEST - 26 (2020)BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 26 (2020)EXERCISE - MATHEMATICS ( SECTION 2)
5 Videos
- JEE MAIN REVISION TEST - 25 | JEE - 2020BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 25 | JEE - 2020EXERCISE - MATHEMATICS
25 Videos
- JEE MAIN REVISION TEST - 27 - JEE -2020BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST - 27 - JEE -2020EXERCISE - MATHEMATICS (SECTION 2)
5 Videos
Similar Questions
Find the area of the region {(x,y):x2+y2≤4,x+y≥2}, using the method of integration.
View SolutionUsing integration, find the area of the region
{(x,y):x2+y2≤1,x+y≥1,x≥0,y≥0}
View SolutionFind the area of the region {(x,y)/x2−x−1≤y≤−1}
View SolutionThe area of the region R={(x,y)/x2≤y≤x} is
View SolutionFind the area of the region {(x,y):x2+y2≤1≤x+y}.
View SolutionIf the line x=α divides the area of region R={(x,y)∈R2:x3≤y≤x,0≤x≤1} into two equal parts, then
View SolutionThe area of the region {(x,y):xy≤8,1≤y≤x2} is :
View SolutionUsing integration ,find the area of the region {(x,y):y2≤4x,4x2+4y2≤9}
View SolutionThe area of the region {(x,y):x2+y2≤5,||x|−|y∣∣≥1 is
View SolutionUsing integration, find the area of the following region : {(x,y):x2+y2<1≤x+y}
View Solution
