Consider a short bar magnet forming a magnetic dipole enclosed by an imaginary co-axial cylindrical surface with circular base area. Magnet is at the middle of cylinder. If magnetic flux through one of the circular base is phi _(0) then the magnetic flux through the other circular base will be:
ϕ0
>ϕ0
−ϕ0
<ϕ0
The correct Answer is:C
To solve the problem, we need to analyze the situation involving a short bar magnet placed inside a cylindrical surface. The magnet creates a magnetic field, and we need to determine the magnetic flux through the two circular bases of the cylinder.
1. Understanding the Setup:
- We have a short bar magnet placed in the center of a cylindrical surface with two circular bases (let's call them Base A and Base B).
- The bar magnet has a North Pole and a South Pole. Magnetic field lines emerge from the North Pole and enter the South Pole.
2. Magnetic Flux Definition:
- Magnetic flux (Φ) through a surface is defined as the product of the magnetic field (B) and the area (A) of the surface, considering the angle (θ) between the magnetic field and the normal to the surface:
Φ=B⋅A⋅cos(θ)
3. Flux through Base A:
- Let’s denote the magnetic flux through Base A as Φ_A. According to the problem, this is given as Φ_0.
4. Flux through Base B:
- The magnetic field lines that enter Base A (the base facing the North Pole) will exit through Base B (the base facing the South Pole).
- Due to the conservation of magnetic field lines, the number of lines entering Base A is equal to the number of lines exiting Base B.
5. Applying Gauss's Law for Magnetism:
- According to Gauss's law for magnetism, the total magnetic flux through a closed surface is zero:
ΦA+ΦB+ΦC=0
- Here, Φ_C is the flux through the cylindrical side surface, which is zero because no magnetic field lines pass through the curved surface of the cylinder.
6. Setting Up the Equation:
- Since Φ_C = 0, we can simplify the equation to:
ΦA+ΦB=0
- This implies:
ΦB=−ΦA
7. Substituting the Known Value:
- We know that Φ_A = Φ_0, therefore:
ΦB=−Φ0
8. Conclusion:
- The magnetic flux through the other circular base (Base B) is:
ΦB=−Φ0
Final Answer:
The magnetic flux through the other circular base will be −Φ0.
---
- We have a short bar magnet placed in the center of a cylindrical surface with two circular bases (let's call them Base A and Base B).
- The bar magnet has a North Pole and a South Pole. Magnetic field lines emerge from the North Pole and enter the South Pole.
- Magnetic flux (Φ) through a surface is defined as the product of the magnetic field (B) and the area (A) of the surface, considering the angle (θ) between the magnetic field and the normal to the surface:
Φ=B⋅A⋅cos(θ)
- Let’s denote the magnetic flux through Base A as Φ_A. According to the problem, this is given as Φ_0.
- The magnetic field lines that enter Base A (the base facing the North Pole) will exit through Base B (the base facing the South Pole).
- Due to the conservation of magnetic field lines, the number of lines entering Base A is equal to the number of lines exiting Base B.
- According to Gauss's law for magnetism, the total magnetic flux through a closed surface is zero:
ΦA+ΦB+ΦC=0
- Here, Φ_C is the flux through the cylindrical side surface, which is zero because no magnetic field lines pass through the curved surface of the cylinder.
- Since Φ_C = 0, we can simplify the equation to:
ΦA+ΦB=0
- This implies:
ΦB=−ΦA
- We know that Φ_A = Φ_0, therefore:
ΦB=−Φ0
- The magnetic flux through the other circular base (Base B) is:
ΦB=−Φ0
The magnetic flux through the other circular base will be −Φ0.
Topper's Solved these Questions
- JEE MAIN REVISON TEST-23BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISON TEST-23EXERCISE - PHYSICS (SECTION 2)
1 Videos
- JEE Main Revision Test-9 | JEE-2020 BOOK - VMC MODULES ENGLISHCHAPTER - JEE Main Revision Test-9 | JEE-2020 EXERCISE - PHYSICS SECTION 2
5 Videos
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - JEE Advanced (archive)
14 Videos
Similar Questions
If the magnetic field is parallel to a surface then, the magnetic flux through the surface is
View SolutionA current carrying circular loop is placed in an infinite plane if ϕi is the magnetic flux through the inner region and ϕo is magnitude of magnetic flux through the outer region, then
View Solution
Knowledge Check
The net magnetic flux through any closed surface, kept in a magnetic field is
AzeroBμ04πC4πμ0D4μ0π
A current carrying circular loop is placed in an infinite plane if ϕi is the magnetic flux through the inner region and ϕo is magnitude of magnetic flux through the outer region, then
View SolutionA circular coil, carrying a constant current i s kept in the x - y plane. The magnetic flux through the entire x - y plane exluding the area of the circular coil is given by ϕ and the magetic flux through the area of the circular coil area is given by ϕ0, then
View SolutionConsider a rectangular coil of wire carrying constant current I, forming a magnetic dipole. The magnetic flux through an infinite plane that contains the circular coil and excluding the rectangular area is given by ϕi. The magnetic flux through the area of the rectangular area is given by ϕ0. Which of the following option is correct?
View SolutionThe net magnetic flux through any closed surface, kept in a magnetic field is
View SolutionConsider a rectangular coil of wire carrying constant current I, forming a magnetic dipole. The magnetic flux through an infinite plane that contains the rectangular coil and excluding the rectangular area is given byϕ. magnetic flux through the area of the rectangular area is given by ϕ. Which of the following option is correct?
View SolutionThe magnetic field perpendicular to the plane of a loop of area 0.1m2 is 0.2 T. Calculate the magnetic flux through the loop (in weber)
View Solution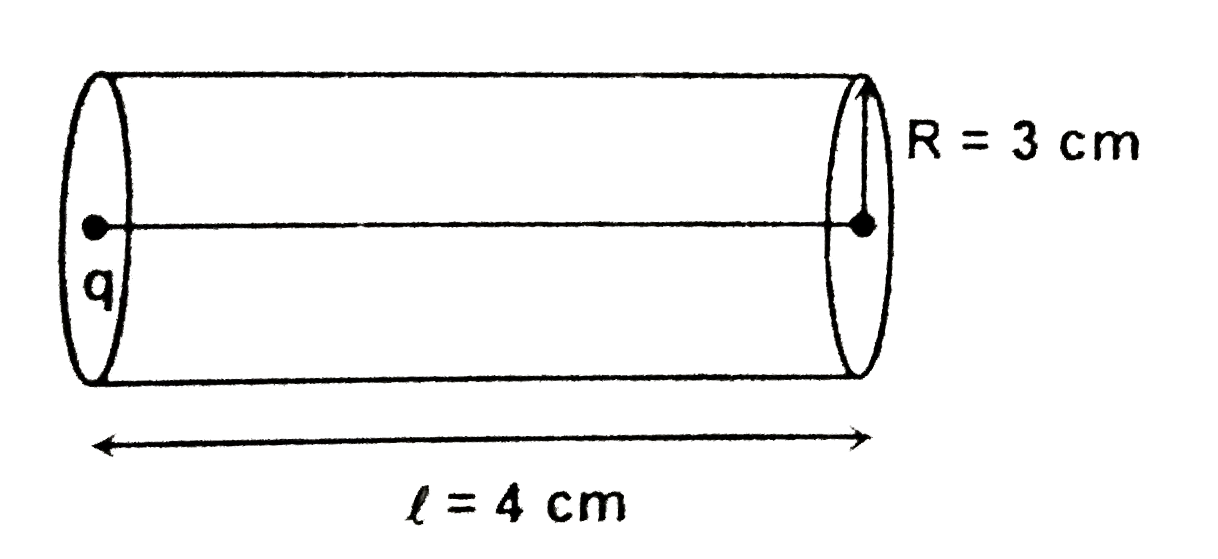
A point charge 'q' is placed at the centre of left circular end of a cylinder of length l=4cm and radius R=3 cm shown. Then the electric flux through the curved surface of the cylinder is
View Solution
