n moles of an ideal gas with constant volume heat capacity CV undergo an isobaric expansion by certain volumes. The ratio of the work done in the process, to the heat supplied is:
4nRCV−nR
nRCV−nR
nRCV+nR
4nRCV+nR
The correct Answer is:C
To solve the problem, we need to find the ratio of the work done (W) to the heat supplied (Q) during an isobaric expansion of an ideal gas. Let's break it down step by step.
Step 1: Understand the Process
In an isobaric process, the pressure (P) remains constant. For an ideal gas, we can use the ideal gas law:
PV=nRT
Where:
- P = Pressure
- V = Volume
- n = Number of moles
- R = Universal gas constant
- T = Temperature
Step 2: Calculate the Work Done (W)
The work done by the gas during an isobaric process can be calculated using the formula:
W=PΔV
Since P is constant, we can express this in terms of temperature change. The change in volume can be related to the change in temperature using the ideal gas law:
W=nR(Tf−Ti)
Where Tf is the final temperature and Ti is the initial temperature.
Step 3: Calculate the Heat Supplied (Q)
The heat supplied to the gas during an isobaric process can be calculated using:
Q=ΔU+W
Where ΔU is the change in internal energy. For an ideal gas, the change in internal energy is given by:
ΔU=nCV(Tf−Ti)
So, substituting for Q:
Q=nCV(Tf−Ti)+nR(Tf−Ti)
Factoring out (Tf−Ti):
Q=n(Tf−Ti)(CV+R)
Step 4: Find the Ratio WQ
Now we can find the ratio of work done to heat supplied:
WQ=nR(Tf−Ti)n(Tf−Ti)(CV+R)
The n and (Tf−Ti) terms cancel out:
WQ=RCV+R
Final Answer
Thus, the ratio of the work done in the process to the heat supplied is:
WQ=RCV+R
---
In an isobaric process, the pressure (P) remains constant. For an ideal gas, we can use the ideal gas law:
PV=nRT
Where:
- P = Pressure
- V = Volume
- n = Number of moles
- R = Universal gas constant
- T = Temperature
The work done by the gas during an isobaric process can be calculated using the formula:
W=PΔV
Since P is constant, we can express this in terms of temperature change. The change in volume can be related to the change in temperature using the ideal gas law:
W=nR(Tf−Ti)
Where Tf is the final temperature and Ti is the initial temperature.
The heat supplied to the gas during an isobaric process can be calculated using:
Q=ΔU+W
Where ΔU is the change in internal energy. For an ideal gas, the change in internal energy is given by:
ΔU=nCV(Tf−Ti)
So, substituting for Q:
Q=nCV(Tf−Ti)+nR(Tf−Ti)
Factoring out (Tf−Ti):
Q=n(Tf−Ti)(CV+R)
Now we can find the ratio of work done to heat supplied:
WQ=nR(Tf−Ti)n(Tf−Ti)(CV+R)
The n and (Tf−Ti) terms cancel out:
WQ=RCV+R
Thus, the ratio of the work done in the process to the heat supplied is:
WQ=RCV+R
Topper's Solved these Questions
- JEE MAIN REVISION TEST -14 Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISION TEST -14 Exercise:PHYSICS (SECTON 2)
Explore 5 Videos
- JEE MAIN REVISION TEST - 7|JEE - 2020Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISION TEST - 7|JEE - 2020Exercise:Physics (Section -2) (Numerical Value Type)
Explore 5 Videos
- JEE MAIN REVISION TEST -17 (2020)Book:VMC MODULES ENGLISHChapter:JEE MAIN REVISION TEST -17 (2020)Exercise:PHYSICS
Explore 25 Videos
Similar Questions
n moles of an ideal gas with constant volume heat capacity CV undergo an isobaric expansion by certain volume.The ratio of the work done in the process, to the heat supplied is :
View SolutionIn an isobaric process of an ideal gas. The ratio of heat supplied annd work done by the system [i.e.,(QW)] is
View SolutionFor an ideal gas, the heat capacity at constant pressure is larger than than that at constant volume because
View SolutionFor polytropic process PVn = constant, molar heat capacity (Cm) of an ideal gas is given by:
View SolutionThe pressure versus volume graph of one mole of an ideal monatomic gas undergoing a cyclic process is shown in figure. The molecular mass of the gas is M.
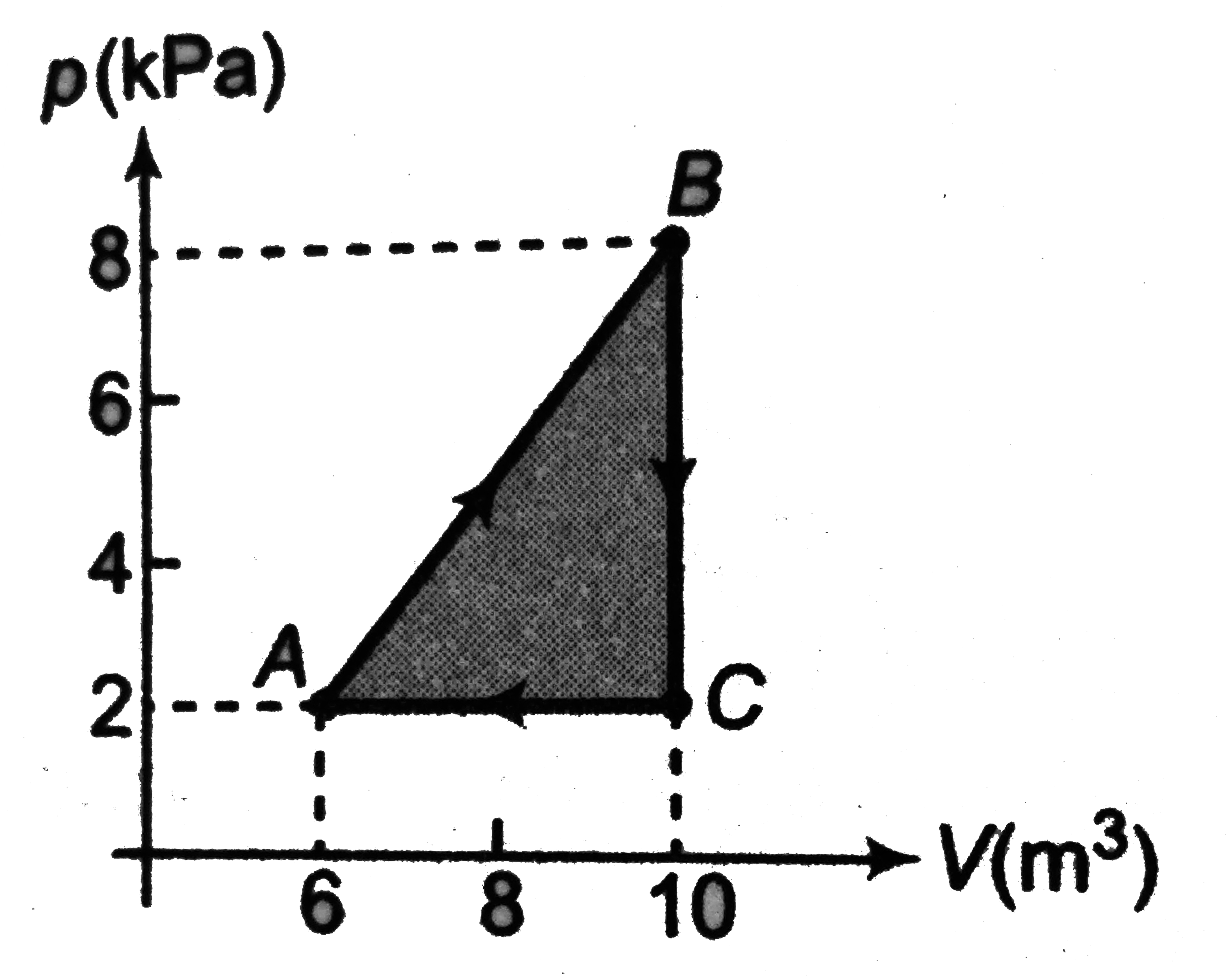
(a) Find the work done in each process.
(b) Find heat rejected by gas in one complete cycle.
(c) Find the effiency of the cycle.
View SolutionOne mole of an ideal monatomic gas undergoes a process described by the equation PV3= constant. The heat capacity of the gas during this process is
View SolutionOne mole of a gas is enclosed in a cylinde in a cyclinder and occupies a volume of 1.5L at a pressure 1.5 atm. It is subjected to strong heating due to which temperature of the gas increase according to the relation T=αV2, where α is a positive constant and V is volume of the gas.
a. Find the work done by air in increasing the volume of gas to 9L.
b. Draw the P−V diagram of the process.
c. Determine the heat supplied to the gas (assuming γ=1.5).
View SolutionTwo moles of an ideal monoatomic gas, initially at pressure p1 and volume V1, undergo an adiabatic compression until its volume is V2. Then the gas is given heat Q at constant volume V2.
(i) Sketch the complete process on a p-V diagram.
(ii) Find the total work done by the gas, the total change in its internal energy and the final temperature of the gas. [Give your answer in terms of p1,V1,V2,QandR]
View Solutionan ideal diatomic gas undergoes a polytropic process described by the equation P√V=constant . The molar heat capacity of the gas during this process is
View SolutionAn ideal gas with adiabatic exponent γ=43 undergoes a process in which internal energy is related to volume as U=V2 . Then molar heat capacity of the gas for the process is :
View Solution