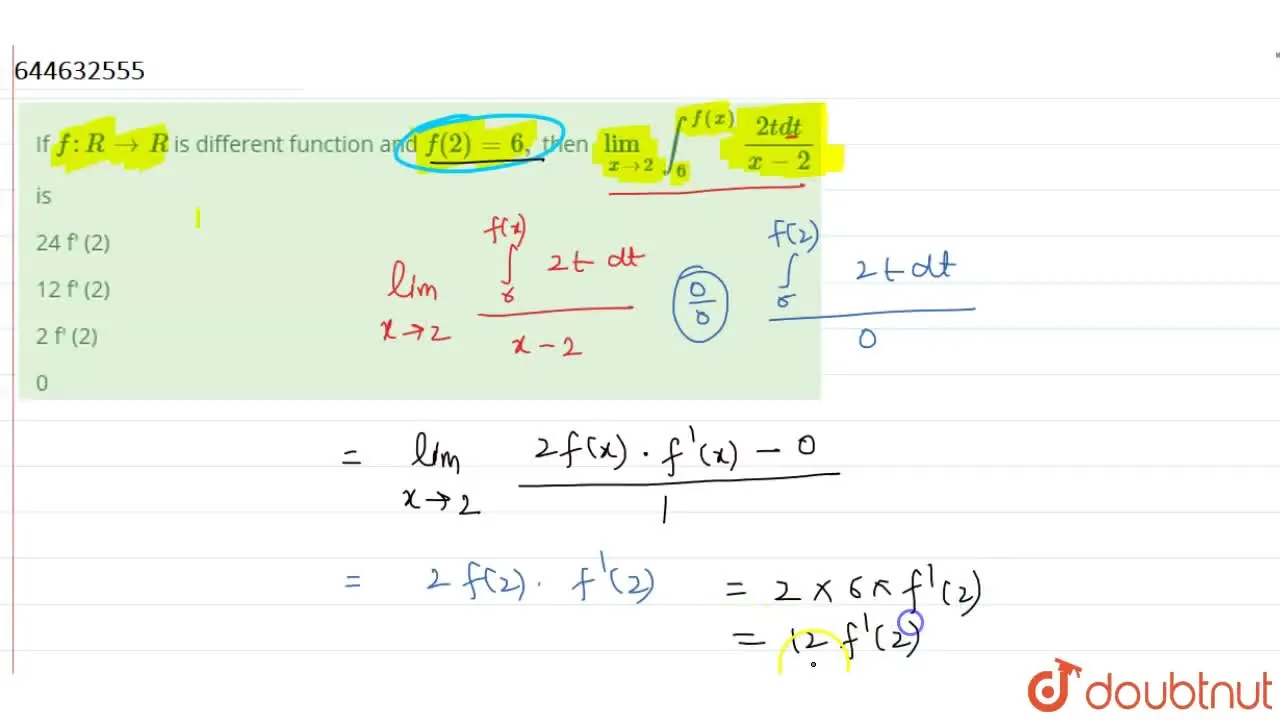
If f:R→R is different function and f(2)=6, then limx→2∫f(x)62tdtx−2 is
24 f' (2)
12 f' (2)
2 f' (2)
0
The correct Answer is:B
limx→2∫f(X)62tx−2dt
Given that f (2) = 6
=limx→2∫f(x)62tdt(x−2)(00form)
limx→2(2f(x)f'x−01−0 Using L.H Rule
=2×6f'(2)
= 12 f' (2)
Related Playlists
- BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST 11 (2020) EXERCISE - MATHEMATICS (SECTION - 2)4videos
JEE MAIN REVISION TEST 11 (2020)
- BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST -17 (2020)EXERCISE - MATHEMATICS25videos
JEE MAIN REVISION TEST -17 (2020)
- BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST 5 (2020)EXERCISE - MATHEMATICS (SECTION 2)5videos
JEE MAIN REVISION TEST 5 (2020)
Similar Questions
Let f:R→R be a differentiable function and f(1)=4. Then, the value of limx→1∫f(x)42tx−1dt is :
View SolutionLet f:R→R be a differentiable function such that f(2)=2. Then, the value of limx→2∫f(x)24t3x−2dt, is
View SolutionLet f:R∈R be a continuous function such that f(1)=2. If limx→1∫f(x)22tx−1dt=4, then the value of f'(1) is
View SolutionLet f:R→R be a continuously differentiable function such that f(2)=6 and f'(2)=148. If ∫f(x)64t3dt=(x−2)g(x) than limx→2g(x) is equal to
View SolutionLet f:R→R be a differentiable function such that f(x)=x2+∫x0e−tf(x−t)dt. f(x) increases for
View SolutionLet f:R+→R be a differentiable function with f(1)=3 and satisfying :
∫xy1f(t)dt=y∫x1f(t)dt+x∫y1f(t)dt∀x,y∈R+, then f(e)=View SolutionLet f:R→R be a differentiable function having f(2)=6,f′(2)=148. Then evaluate limx→2∫f(x)64t3x−2dt
View SolutionLet f:R→R be a differentiable function such that f(x)=x2+∫x0e−tf(x−t)dt.
y=f(x) isView SolutionIf f:R∈R is continuous and differentiable function such that
∫x−1f(t)dt+f'''(3)∫0xdt=∫x1t3dt−f'(1)∫x0t2dt+f'(2)∫3xrdt, then the value of f'(4), isView SolutionLet f be a differentiable function on R and satisfying the integral equation
x∫x0f(t)dt−∫x0tf(x−t)dt=ex−1∀x∈R. Then f(1) equals to ___View Solution