A helicopter flying along the path y=7+x32, A soldier standint at point (12,7) wants to hit the helicopter when it is closest from him, then minimum distance is equal to
√56
13√73
12
16√73
The correct Answer is:D
y=7+x3/2
Let the point on curve be P(x1,7+x3/21) and given point be A(12,7) for the nearest point normal at P passes through A, so slope of line AP = slope of normal at P.
x3/21x1−12=−dxdy=−23√x1
3x21=1−2x1
3x21+2x1−1=0
(x1+1)(3x1−1)=0
x1=13,[x1=−1(not possible as x1>0)]
Point P(13,7+13√3)
So,AP=√136+127=16√73
Topper's Solved these Questions
- JEE MAIN REVISION TEST-3 (2020)BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISION TEST-3 (2020)EXERCISE - MATHEMATICS (SECTION 2)
5 Videos
- JEE Main Revision Test-20 | JEE-2020 BOOK - VMC MODULES ENGLISHCHAPTER - JEE Main Revision Test-20 | JEE-2020 EXERCISE - MATHEMATICS
25 Videos
- JEE Main Revision Test-6 | JEE-2020BOOK - VMC MODULES ENGLISHCHAPTER - JEE Main Revision Test-6 | JEE-2020EXERCISE - MATHEMATICS
25 Videos
Similar Questions
An Apache helicopter of enemy is flying along the curve given by y=x2+7. A soldier, placed at (3, 7), wants to shoot down the helicopter when it is nearest to him. Find the nearest distance.
View SolutionFind the point on the curve y2=2x which is at a minimum distance from the point (1, 4) .
View SolutionThe distance between points (2,−7,3) and (−2,7,3) is:
View SolutionQ is the image of point P(1, -2, 3) with respect to the plane x−y+z=7. The distance of Q from the origin is.
View SolutionA helicopter is flying at 200m and flying at 25ms−1 at an angle 37∘ above the horizontal when a package is dropped from it.
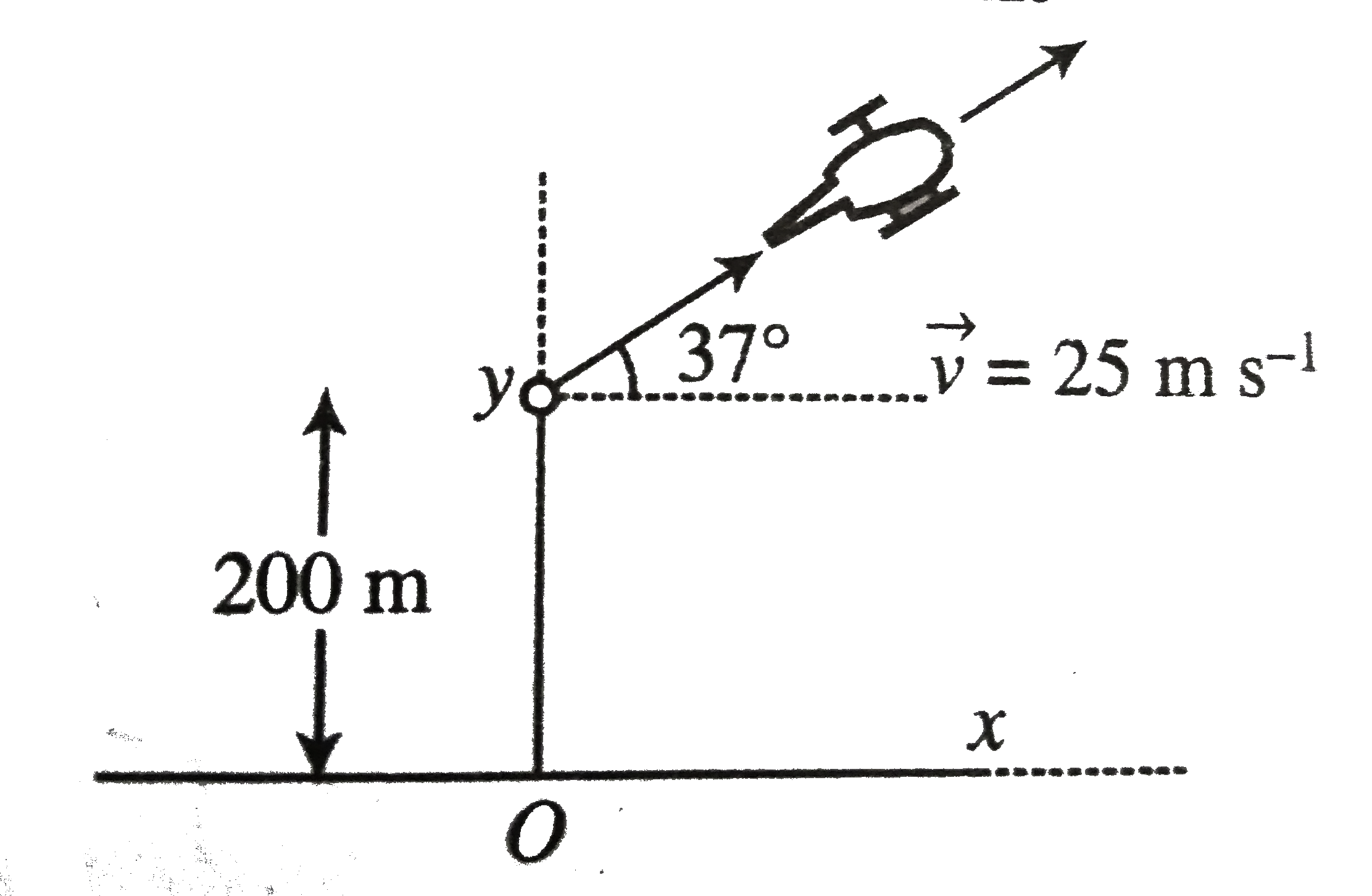
If the helicopter flies at constant velocity, find the x and y coordinates of the location of the helicopter when the package lands.
View SolutionA helicopter is flying at 200m and flying at 25ms−1 at an angle 37∘ above the horizontal when a package is dropped from it.
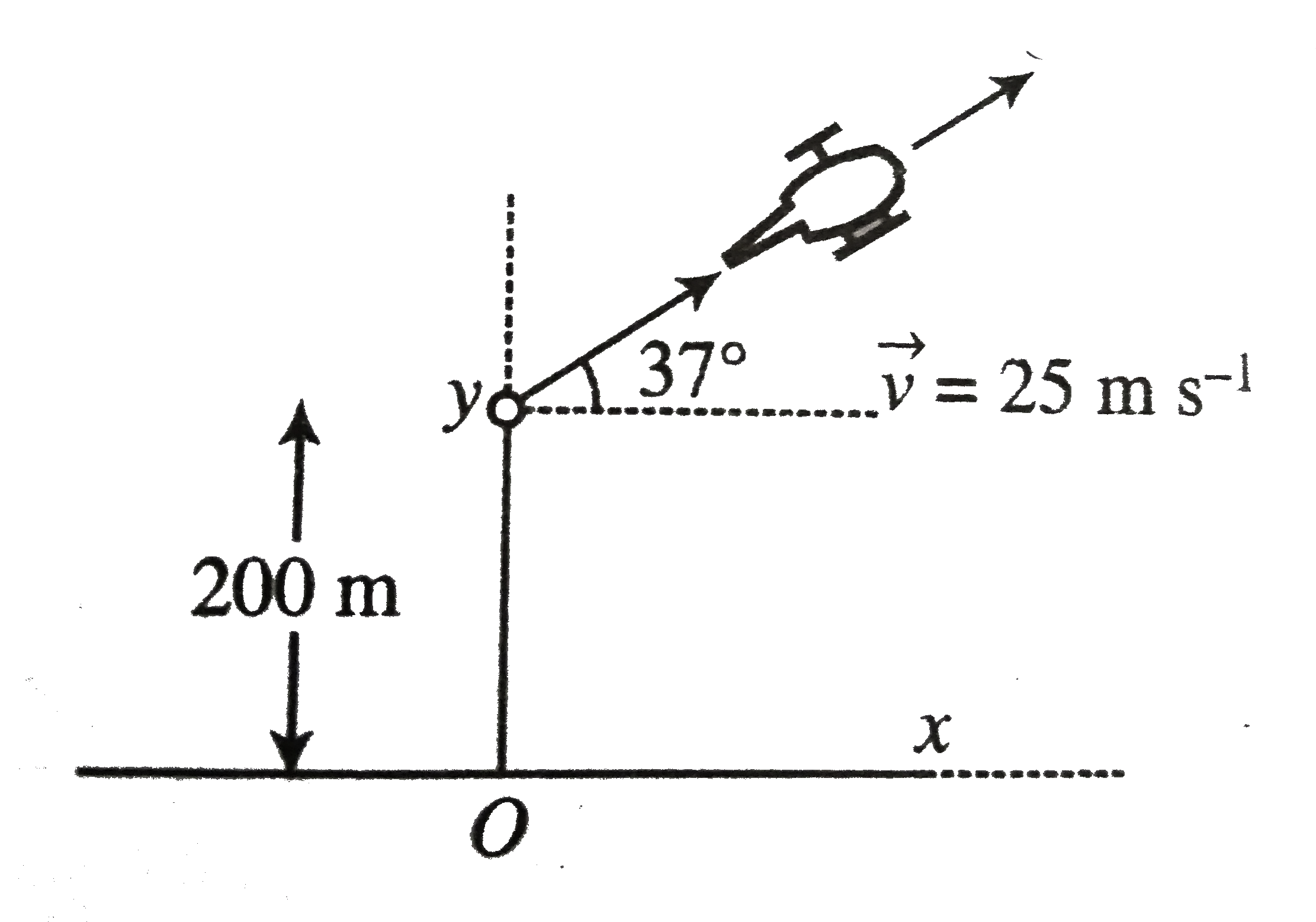
The distance of the point O where the package lands is
View SolutionA plane 2x+3y+5z=1 has a point P which is at minimum distance from line joining A(1,0,−3),B(1,−5,7), then distance AP is equal to
View SolutionA helicopter is moving verticallly upwards with a velocity 5ms−1. When the helicopter is at a height 10m from ground, a stone is thrown with a velocity (3ˆi+4ˆj)ms−1 from the helicopter w.r.t. the man in it. Considering the point on ground vertically below the helicopter as the origin of coordinates, and the ground below as xy plane, find the coordinates of the point where the stone will fall, its distance from origin at the instant the stone strikes the ground, assuming helicopter moves upwards with constant velocity.
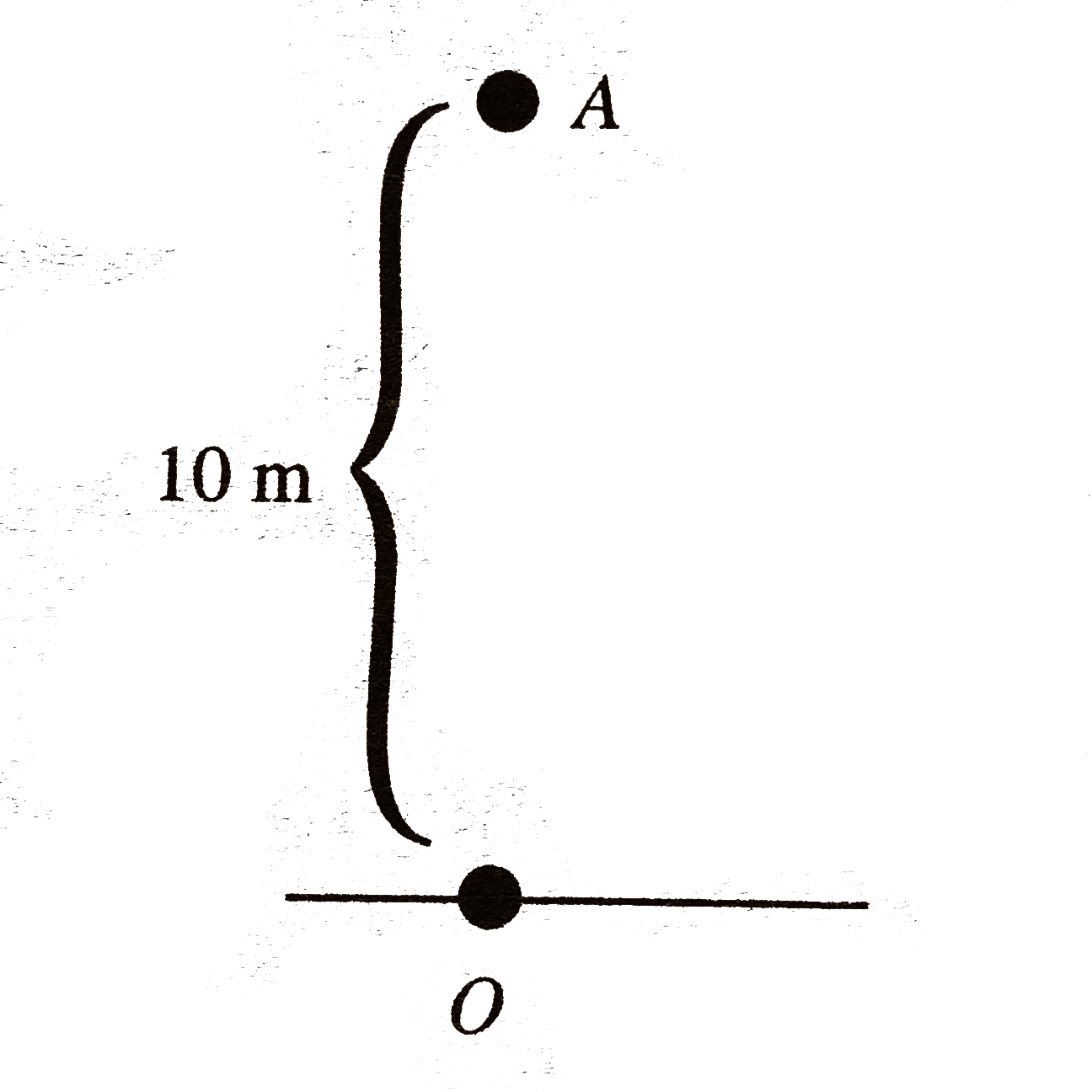 .
.
View SolutionThe distance between the points (1,3) and (x,7) is 5, find x.
View SolutionA jet of enemy is along the curve y=x2+2 and a soldier is placed at (3,2).Find the minimum distance between the jet and soldier.
View Solution

