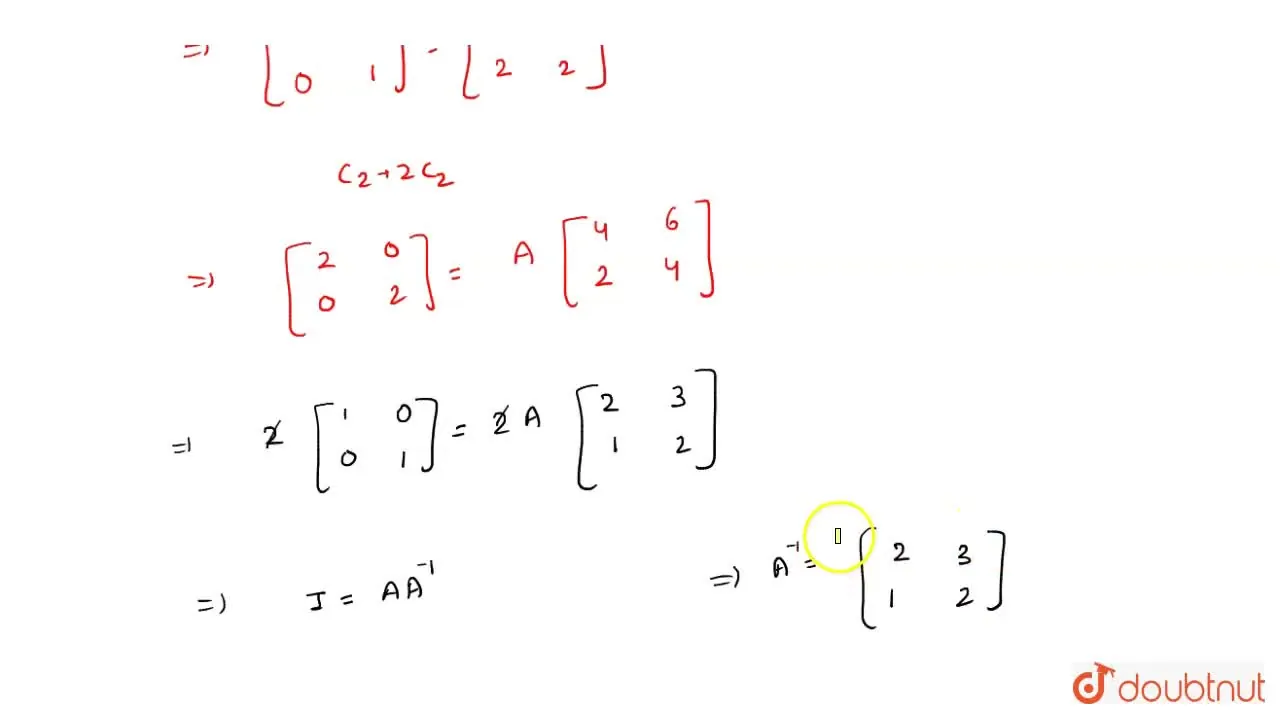Find using column transformations :
The correct Answer is:
Step by step video, text & image solution for Find A^(-1) using column transformations : A = [(2,-3),(-1,2)] by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - TRIGONOMETRIC FUNCTIONS44videos
QUESTION BANK 2021
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - PAIRS OF LINES21videos
QUESTION BANK 2021
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - MATHEMATICAL LOGIC32videos
QUESTION BANK 2021
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - MULTIPLE CHOICE QUESTIONS9videos
PROBABILITY DISTRIBUTION
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - MULTIPLE CHOICE QUESTIONS8videos
THREE DIMENSIONAL GEOMETRY
Similar Questions
Find the inverse of the following , if it exists, by using elementary row (column) transformations :
[2−3−12]View SolutionFind the inverse of the following , if it exists, by using elementary row (column) transformations :
[2−61−2]View SolutionFind the inverse of the following , if it exists, by using elementary row (column) transformations :
[3512]View SolutionFind the inverse of the following , if it exists, by using elementary row (column) transformations :
[3−1−42]View SolutionFind the inverse of the following , if it exists, by using elementary row (column) transformations :
[6−3−21]View SolutionFind the inverse of the following , if it exists, by using elementary row (column) transformations :
[2357]View SolutionFind the inverse of the following , if it exists, by using elementary row (column) transformations :
[−43−54]View SolutionFind the inverse of the following , if it exists, by using elementary row (column) transformations :
[1−123]View SolutionFind the inverse of the following , if it exists, by using elementary row (column) transformations :
[122−1]View SolutionFind the inverse of the following , if it exists, by using elementary row (column) transformations :
[1−221]View Solution