Currents of equal magnitude pass through two long parallel wires having separation of 1.35 cm. If the force per unit length on each wire is 4.76×10−2 N/m, what is I ?
The correct Answer is:56.68 A
To solve the problem, we will use the formula for the force per unit length between two parallel wires carrying currents. The force per unit length F/L between two long parallel wires is given by the formula:
FL=μ04π⋅2I1I2D
Where:
- F is the force between the wires,
- L is the length of the wires,
- μ0 is the permeability of free space (4π×10−7T m/A),
- I1 and I2 are the currents through the wires,
- D is the separation between the wires.
Given:
- D=1.35cm=1.35×10−2m
- FL=4.76×10−2N/m
- Since the currents are equal, we can set I1=I2=I.
Step 1: Substitute the known values into the formula
We can rewrite the formula as:
FL=μ04π⋅2I2D
Substituting the known values:
4.76×10−2=(4π×10−7)4π⋅2I21.35×10−2
Step 2: Simplify the equation
The 4π cancels out:
4.76×10−2=10−72⋅2I21.35×10−2
This simplifies to:
4.76×10−2=10−7I21.35×10−2
Step 3: Rearranging to solve for I2
Multiply both sides by 1.35×10−2:
(4.76×10−2)⋅(1.35×10−2)=10−7I2
Calculating the left side:
(4.76×1.35)×10−4=10−7I2
6.426×10−4=10−7I2
Step 4: Solve for I2
Now, divide both sides by 10−7:
I2=6.426×10−410−7=6.426×103
Step 5: Take the square root to find I
I=√6.426×103≈80.1A
Final Answer
The current I is approximately 80.1A.
---
- F is the force between the wires,
- L is the length of the wires,
- μ0 is the permeability of free space (4π×10−7T m/A),
- I1 and I2 are the currents through the wires,
- D is the separation between the wires.
- D=1.35cm=1.35×10−2m
- FL=4.76×10−2N/m
- Since the currents are equal, we can set I1=I2=I.
Topper's Solved these Questions
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Magnetic Effect of Electric Current ( Short Answer II (SA2) ( 3 MARKS Each ) )
7 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Magnetic Effect of Electric Current (Long Answer ( LA) ( 4 marks Each) )
3 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Magnetic Effect of Electric Current (Very Short Answer (VSA) ( 1 MARK Each ) )
7 Videos
- MULTIPLE CHOICE QUESTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MULTIPLE CHOICE QUESTIONSEXERCISE - Communication Systems
6 Videos
- SHORT ANSWER QUESTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SHORT ANSWER QUESTIONSEXERCISE - Assignments
3 Videos
Similar Questions
Two parallel wires have separation 0.1m and each carries 5A current.The force per metre on either wire is: ?
View SolutionTwo long parallel wires are separated by a distance of 2.50cm. The force per unit length that each wire exerts on the other is 4.00×10−5Nm, and the wires repel each other. The current in one wire is 0.600A.
a. What is the current in the second wire ?
b. Are the two currents in the same direction or in opposite direction?
View Solution
Knowledge Check
If same current I passing through two parallel wires separated by a distance b, then force per unit length will he
Aμ04π2I2bBμ0I4πbCμ0I24πb2Dμ0I24πbTwo long parallel conducting wires carry current I in same direction, placed at distance b. Force per unit length of wire is
Aμ0I2πbBμ0I22πb2Cμ0I22πbDμ0I2πb2Currents of 10A,2A are passed through two parallel wires A and B respectively in opposite directions. If the wire A is infinitely long and the length of the wire B is 2 metre, the force on the conductor B, which is situated at 10cm distance from A will be
A8×10−5newtonB5×10−5 netwonC8π×10−7 newtonD4π×10−7 newton
A straight , long wire carries a current a current of 20A. Another wire carrying equal current is placed parallel to it. If the force acting on a length of 10cm of the second wire is (2.0×(10−5))N, what is the separation between them?
View SolutionThree long, straight and parallel wires are arranged as shown in Fig. The forces experienced by 10 cm length of wire Q is
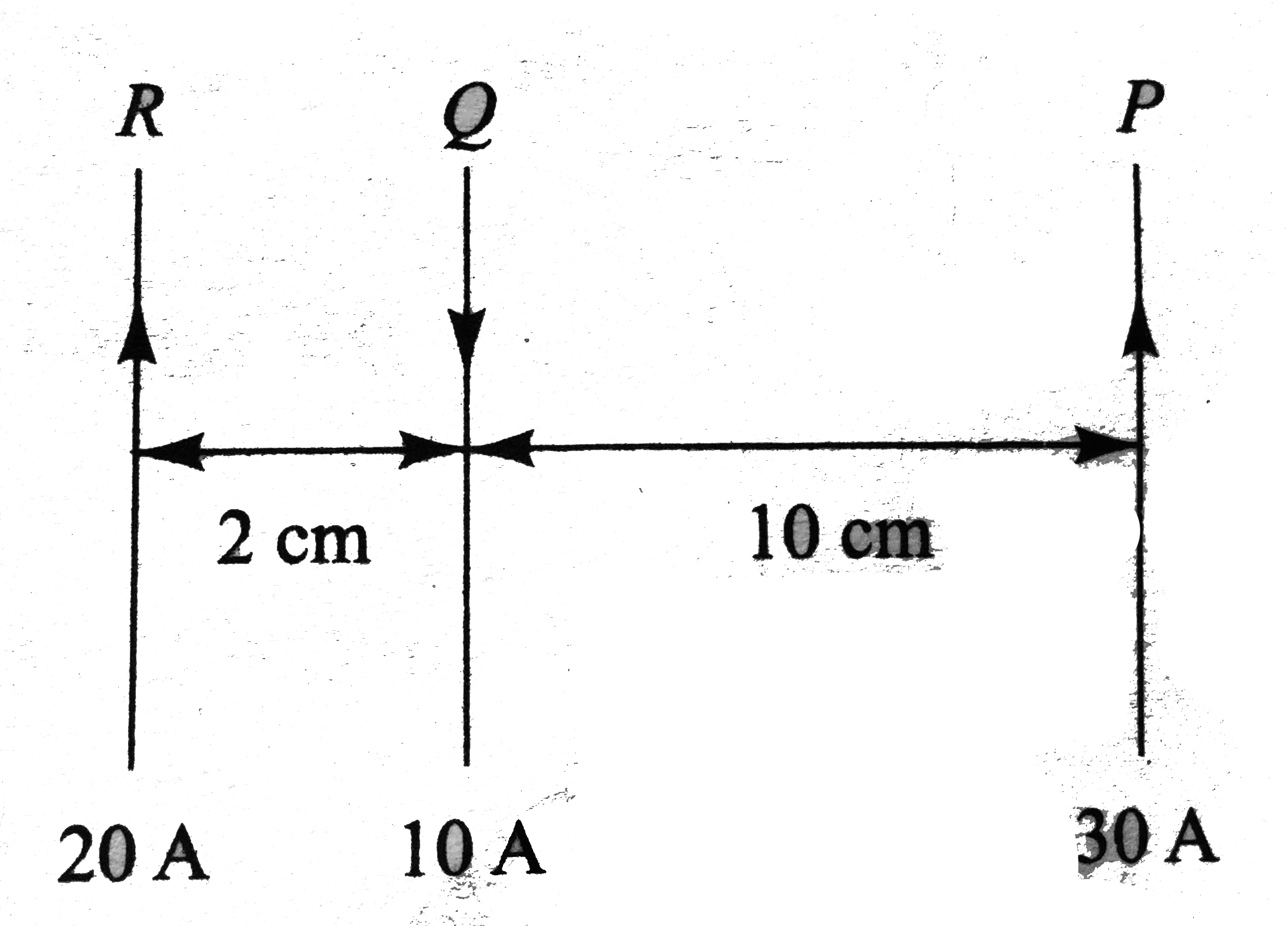
View SolutionIf same current 'I' is flowing through two parallel wires, separted by the distance 'b' in air, then force acting per unit length of wires will be (μ0=permeability of free space)
View SolutionEqual currents are passing through two very long and straight parallel wires in the same direction. They will _________
View SolutionTwo long parallel copper wires carry current of 5A each in opposite directions. If the wires are separated by a distance distance of 0.5m, then the force per unit length between the two wires is
View Solution
