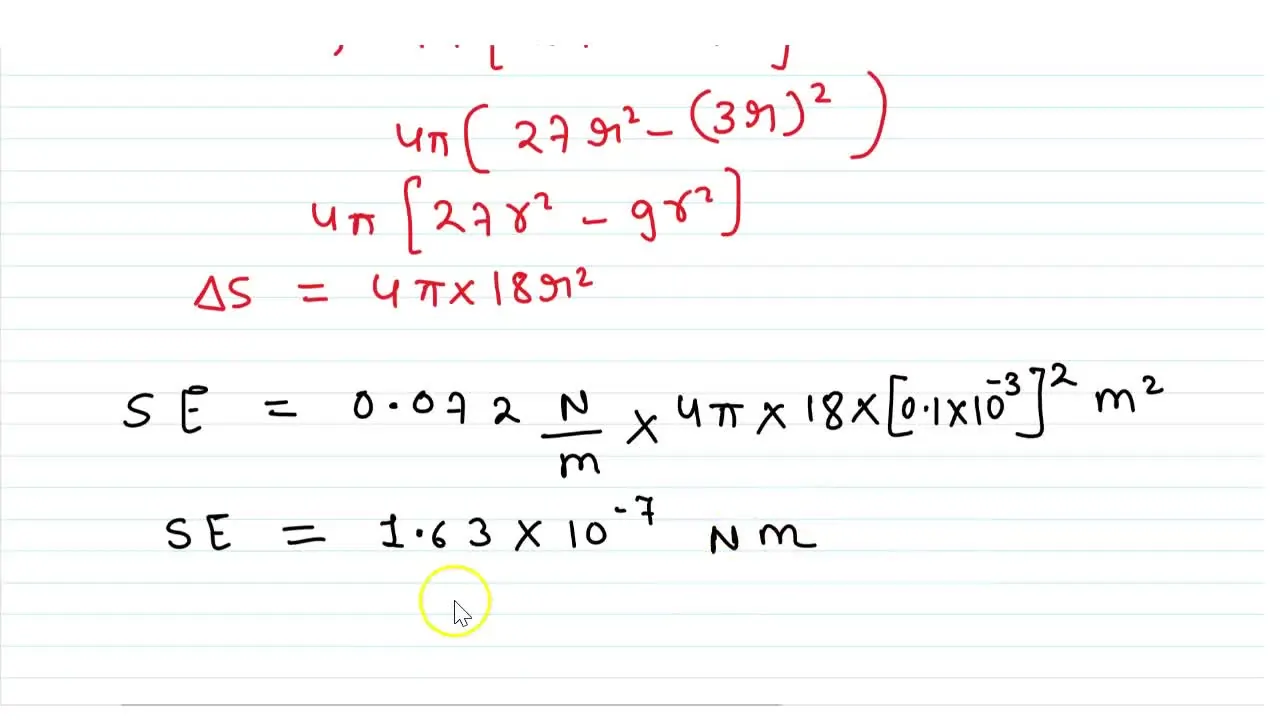Twenty seven droplets of water, each of radius 0.1 mm coalesce into a single drop. Find the change in surface energy. Surface tension of water is 0.072 N/m.
The correct Answer is:W=1.628×10−7J
Step by step video, text & image solution for Twenty seven droplets of water, each of radius 0.1 mm coalesce into a single drop. Find the change in surface energy. Surface tension of water is 0.072 N/m. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Mechanical Properties of fluids (Long Answer ( LA) ( 4 marks Each) )3videos
QUESTION BANK 2021
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Kinetic Theory of gases and Radiation (MCQ’S (1 Mark Each) )7videos
QUESTION BANK 2021
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Mechanical Properties of fluids (Short Answer I (SA1) ( 2 MARKS Each ) )6videos
QUESTION BANK 2021
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MULTIPLE CHOICE QUESTIONSEXERCISE - Communication Systems6videos
MULTIPLE CHOICE QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SHORT ANSWER QUESTIONSEXERCISE - Assignments3videos
SHORT ANSWER QUESTIONS
Similar Questions
Eight droplets of water each of radius 0.5 mm when coalesce into single drop , then the change in surface energy will be ,
View SolutionA water drop of radius 10−2 m is brokenn into 1000 equal droplets. Calculate the gain in surface energy. Surface tension of water ils 0.075Nm−1
View SolutionA drop of mercury of radius 2 mm is split into 8 identical droplets. Find the increase in surface energy. Surface tension of mercury =0.465Jm−2
View SolutionWhat amount of energy will be liberated if 1000 droplets of water, each of diameter 10−8cm, coalesce to form a bigger drop. Surface tension of water is 0.072Nm−1.
View Solutionn' droplets of equal of radius r coalesce to form a bigger drop of radius R. The energy liberated is equal to ( T = Surface tension of water )
View Solution1000 droplets of water having 2 mm diameter each coalesce to form a single drop . Given the surface tension of water is 0.072Nm−1 .the energy loss in the process is
View SolutionA drop of water of radius 4 mm splits into droplets such that the mcrease in surface area is 300π times the surface area of the original drop. The increase in surface energy is
[Surface tension of water = 0.07 N/m]View SolutionA liquid drop of diameter 6 mm breaks into 27 droplets of same size. What will be the change in surface energy if surface tension of liquid drop is 0.06Nm−1?
View SolutionCalculate the energy released when 1000 small water drops each of same radius 10−7m coalesce to form one large drop. The surface tension of water is 7.0×10−2N/m.
View SolutionTwo small drops of mercury, each of radius R, coalesce to form a single large drop. The ratio of the total surface energies before and after the change is
View Solution