Two parallel conducting plates of a capacitor of capacitance C containing charges Q and -2Q at a distance d apart. Find out potential difference between the plates of capacitors.
To find the potential difference between two parallel conducting plates of a capacitor with capacitance C, containing charges Q and −2Q, and separated by a distance d, we can follow these steps:
Step 1: Understand the Charges on the Plates
Given that one plate has a charge Q and the other has a charge −2Q, we can denote the charges as follows:
- Plate 1: Charge Q
- Plate 2: Charge −2Q
Step 2: Calculate the Electric Field Between the Plates
The electric field E between two parallel plates can be calculated using the formula:
E=σϵ0
where σ is the surface charge density and ϵ0 is the permittivity of free space. The surface charge density σ can be calculated as:
σ=QA(for Plate 1)
σ′=−2QA(for Plate 2)
The net electric field E between the plates is the vector sum of the electric fields due to each plate. The electric field due to Plate 1 (positive charge) points away from the plate, while the electric field due to Plate 2 (negative charge) points towards it. Thus, the total electric field E is given by:
E=E1+E2=Q2Aϵ0+2Q2Aϵ0=3Q2Aϵ0
Step 3: Calculate the Potential Difference
The potential difference V between the plates can be calculated using the relationship:
V=E⋅d
Substituting the expression for the electric field we found:
V=(3Q2Aϵ0)⋅d
Final Expression
Thus, the potential difference V between the plates is:
V=3Qd2Aϵ0
Given that one plate has a charge Q and the other has a charge −2Q, we can denote the charges as follows:
- Plate 1: Charge Q
- Plate 2: Charge −2Q
The electric field E between two parallel plates can be calculated using the formula:
E=σϵ0
where σ is the surface charge density and ϵ0 is the permittivity of free space. The surface charge density σ can be calculated as:
σ=QA(for Plate 1)
σ′=−2QA(for Plate 2)
E=E1+E2=Q2Aϵ0+2Q2Aϵ0=3Q2Aϵ0
The potential difference V between the plates can be calculated using the relationship:
V=E⋅d
Substituting the expression for the electric field we found:
V=(3Q2Aϵ0)⋅d
Thus, the potential difference V between the plates is:
V=3Qd2Aϵ0
Topper's Solved these Questions
- CAPACITANCEBook:RESONANCE ENGLISHChapter:CAPACITANCEExercise:Miscellaneous Solved Example
Explore 3 Videos
- CAPACITANCEBook:RESONANCE ENGLISHChapter:CAPACITANCEExercise:Exercise - 1
Explore 79 Videos
- ATOMIC PHYSICSBook:RESONANCE ENGLISHChapter:ATOMIC PHYSICSExercise:Advanved level problems
Explore 17 Videos
- COMMUNICATION SYSTEMSBook:RESONANCE ENGLISHChapter:COMMUNICATION SYSTEMSExercise:Exercise 3
Explore 13 Videos
Similar Questions
In a parallel plate capacitor of capacitor 1μF two plates are given charges 2μC and 4μC the potential difference between the plates of the capacitor is
_2019_CBT2_E01_002_Q01.png)
View SolutionA charge of 1μC is given to one plate of a parallel - plate capacitor of capacitance 0.1μF and a charge of 2μC is given to the other plate . Find the potential difference developed between the plate .
View Solution
Knowledge Check
As the distance between the plates of a parallel plate capacitor decreased
AIf both assertion and reason are ture and reason is the correct explanation of assertion.BIf both assertin and reason are ture but reason is not the correct explanation of assertion .CIf assertion is true but reason is false.DIf both assertion and reason are false.
The force between the plates of a parallel plate capacitor of capacitance C and distance of separation of the plates d with a potential difference V between the plates, is.
View SolutionIn an isolated parallel plate capacitor of capacitance C the four surfaces have charges Q1,Q2,Q3 and Q4 as shown in the figure. The potential difference between the plates is :
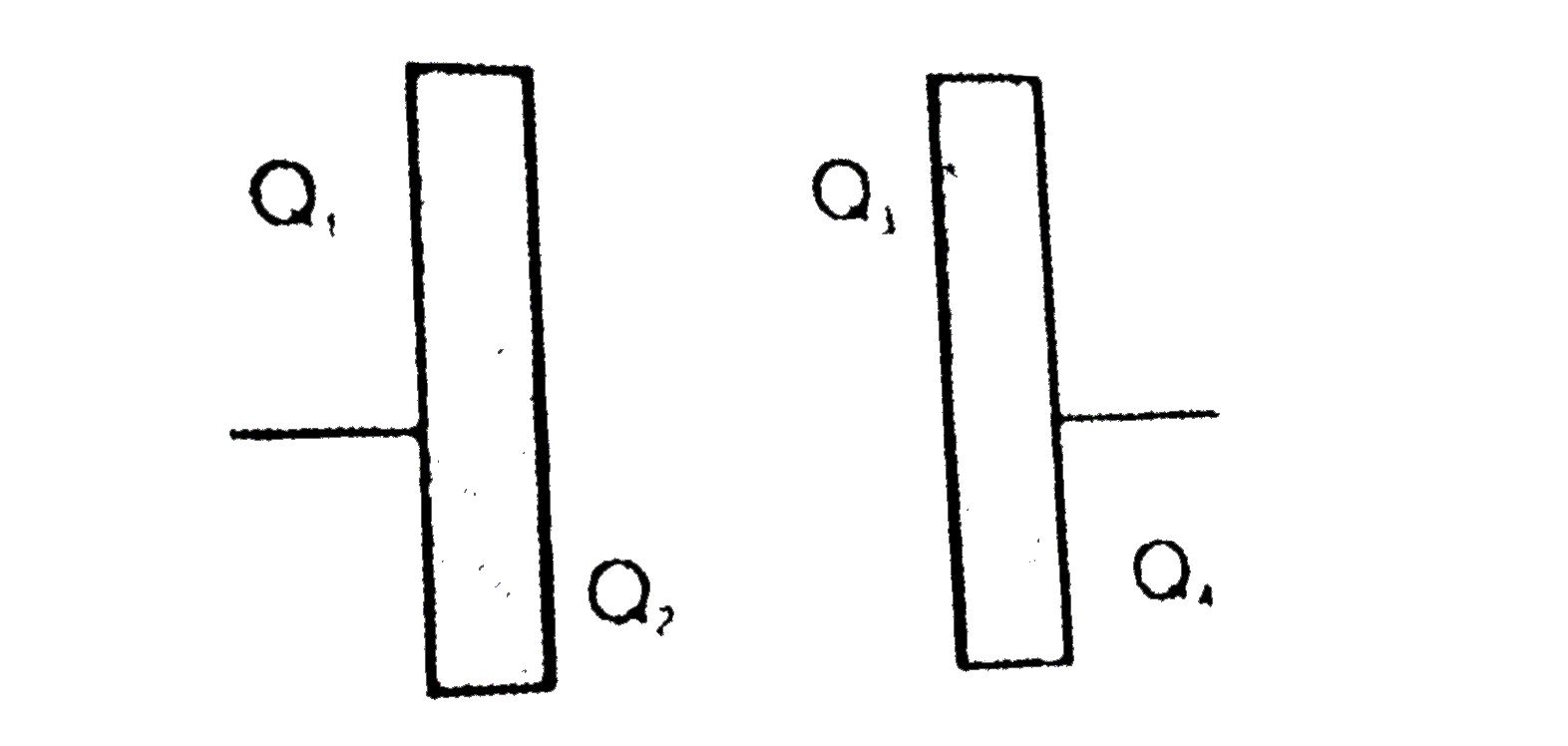
View SolutionA parallel plate capacitor of capacitance C is charged to a potential V by a battery. Without disconnecting the battery, distance between the plates of capacitor is tripled and a dielectric medium of K = 10 is introduced between the plates of capacitor. Explain giving reasons how will the following be affected ?
(a) Capacitance of capacitor
(b) Charge on capacitor
(c ) Energy density of capacitor.
View SolutionA parallel plate capacitor of capacitance C has been charged, so that the potential difference between its plates is V. Now, the plates of this capacitor are connected to another uncharged capacitor of capacitance 2C. Find the common potential acquired by the system and loss of energy.
View SolutionA parallel plate air capacitor has capcity C distance of separtion between plates is d and potential difference V is applied between the plates force of attraction between the plates of the parallel plate air capacitor is
View SolutionTwo large conducting plates of a parallel plate capacitor are given charges Q and 3Q respectively. If the electric field in the region between the plates is E0 , then the force of interaction between the plates is

View SolutionTwo parallel plate capacitors of capacitances C and 2C are connected in parallel and charged to a potential difference V. The battery is then disconnected and the region between the plates of the capacitor C is completely filled with a material of dielectric constant K. The potential differences across the capacitors now becomes...........
View Solution