An altenating voltage of 220volts r.m.s at a frequency of 40 cycles/sec is supplied to a circuit containing a pure inductance of 0.01H and a pure resitance of 6ohm in series. Calculate (i) the current, (ii) potential difference across the resistance, (iii) potential difference across the inductance, (iv) the time lag, (v) power factor.
To solve the problem step by step, we will calculate the required quantities one by one.
Given Data:
- RMS Voltage, Vrms=220V
- Frequency, f=40Hz
- Inductance, L=0.01H
- Resistance, R=6Ω
Step 1: Calculate Angular Frequency (ω)
The angular frequency ω is calculated using the formula:
ω=2πf
Substituting the given frequency:
ω=2π×40=80πrad/s
Step 2: Calculate Inductive Reactance (XL)
Inductive reactance XL is given by:
XL=ωL
Substituting the values:
XL=80π×0.01=0.8π≈2.51Ω
Step 3: Calculate Impedance (Z)
The total impedance Z in the circuit is given by:
Z=√R2+X2L
Substituting the known values:
Z=√62+(2.51)2=√36+6.3001≈√42.3001≈6.51Ω
Step 4: Calculate RMS Current (Irms)
The RMS current is calculated using Ohm's law:
Irms=VrmsZ
Substituting the values:
Irms=2206.51≈33.8A
Step 5: Calculate Potential Difference Across the Resistance (VR)
The potential difference across the resistance is given by:
VR=Irms×R
Substituting the values:
VR=33.8×6≈202.8V
Step 6: Calculate Potential Difference Across the Inductance (VL)
The potential difference across the inductance is given by:
VL=Irms×XL
Substituting the values:
VL=33.8×2.51≈84.8V
Step 7: Calculate Time Lag (ϕ)
The time lag ϕ can be calculated using the formula:
tanϕ=VLVR
Substituting the values:
tanϕ=84.8202.8≈0.418
Now, to find ϕ:
ϕ=tan−1(0.418)≈22.5∘
Step 8: Calculate Power Factor
The power factor cosϕ is given by:
cosϕ=RZ
Substituting the values:
cosϕ=66.51≈0.921
Summary of Results:
1. Current (Irms): ≈33.8A
2. Potential Difference Across Resistance (VR): ≈202.8V
3. Potential Difference Across Inductance (VL): ≈84.8V
4. Time Lag (ϕ): ≈22.5∘
5. Power Factor: ≈0.921
- RMS Voltage, Vrms=220V
- Frequency, f=40Hz
- Inductance, L=0.01H
- Resistance, R=6Ω
The angular frequency ω is calculated using the formula:
ω=2πf
Substituting the given frequency:
ω=2π×40=80πrad/s
Inductive reactance XL is given by:
XL=ωL
Substituting the values:
XL=80π×0.01=0.8π≈2.51Ω
The total impedance Z in the circuit is given by:
Z=√R2+X2L
Substituting the known values:
Z=√62+(2.51)2=√36+6.3001≈√42.3001≈6.51Ω
The RMS current is calculated using Ohm's law:
Irms=VrmsZ
Substituting the values:
Irms=2206.51≈33.8A
The potential difference across the resistance is given by:
VR=Irms×R
Substituting the values:
VR=33.8×6≈202.8V
The potential difference across the inductance is given by:
VL=Irms×XL
Substituting the values:
VL=33.8×2.51≈84.8V
The time lag ϕ can be calculated using the formula:
tanϕ=VLVR
Substituting the values:
tanϕ=84.8202.8≈0.418
Now, to find ϕ:
ϕ=tan−1(0.418)≈22.5∘
The power factor cosϕ is given by:
cosϕ=RZ
Substituting the values:
cosϕ=66.51≈0.921
1. Current (Irms): ≈33.8A
2. Potential Difference Across Resistance (VR): ≈202.8V
3. Potential Difference Across Inductance (VL): ≈84.8V
4. Time Lag (ϕ): ≈22.5∘
5. Power Factor: ≈0.921
Topper's Solved these Questions
- ALTERNATING CURRENTBook:RESONANCE ENGLISHChapter:ALTERNATING CURRENTExercise:EXERCISE SECTION A
Explore 4 Videos
- ALTERNATING CURRENTBook:RESONANCE ENGLISHChapter:ALTERNATING CURRENTExercise:SECTION B
Explore 15 Videos
- ALTERNATING CURRENTBook:RESONANCE ENGLISHChapter:ALTERNATING CURRENTExercise:EXAMPLE
Explore 40 Videos
- ATOMIC PHYSICSBook:RESONANCE ENGLISHChapter:ATOMIC PHYSICSExercise:Advanved level problems
Explore 17 Videos
Similar Questions
An alternating voltage of 220 volts r.m.s. at a freqency of 40 cycles/sec is supplied to a circuit containing a pure inductance of 0.01H and a pure resistance of 6ohms in series.Calculate (i)the current , (ii)potential diffence across the resistance, (iii)potential difference across the inductance, (iv)the time lag, (v)power factor.
View SolutionAn alternating voltage of 220 volts r.m.s. at a freqency of 40 cycles/sec is supplied to a circuit containing a pure inductance of 0.01H and a pure resistance of 6ohms in series.Calculate (i)the current , (ii)potential diffence across the resistance, (iii)potential difference across the inductance, (iv)the time lag, (v)power factor.
View SolutionIn an ac circuit the potential differences across an inductance and resistance joined in series are, respectively, 16 V and 20 V. The total potential difference across the circuit is
View SolutionIn an ac circuit the potential differences across an inductance and resistance joined in series are, respectively, 16 V and 20 V. The total potential difference across the circuit is
View SolutionA 4μF capacitor, a resistance of 2.5MΩ is in series with 12V battery. Find the time after which the potential difference across the capacitor is 3 times the potential difference across the resistor. [ Given In (2) = 0.693]
View SolutionA potential difference of 6V is applied to a coil of inductance 0.5H and a resistance of 4 ohm connected in series. The time taken for the current to reach half the maximum value is (in seconds)
View SolutionAn alternating e.m.f. of 200 V and 50 cycles is connected to a circuit of resistance 3.142Ω) and inductance 0.01 H. The lag in time between the e.m.f. and the current is
View SolutionIn the circuit shown in figure potential differences across 6Ω resistance is 4 volt. Find V and i values across each resistance. Also find emf E of the applied battery.
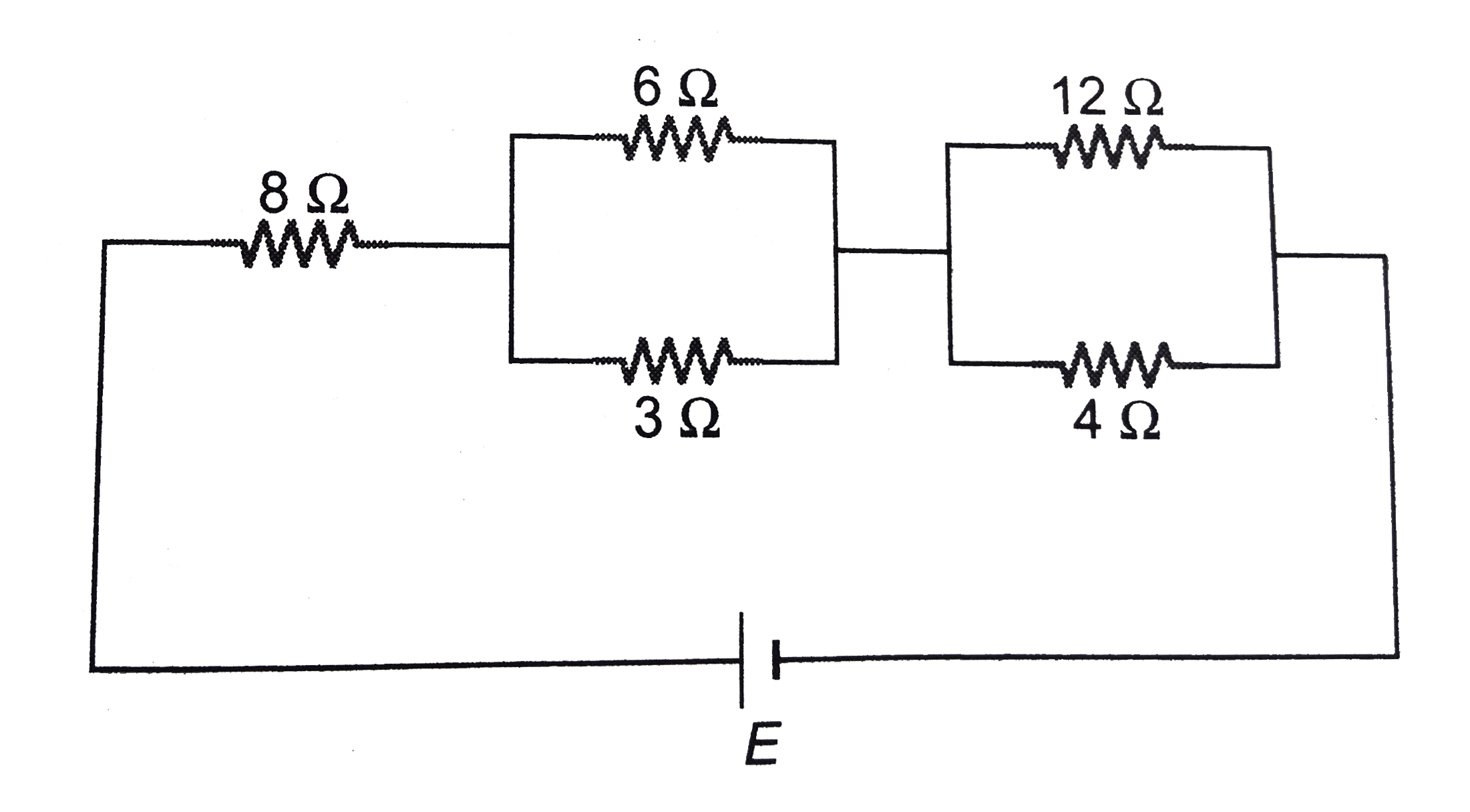
View SolutionA circuit contains resistance R and an inductance L in series. An alternating voltage V=V0sinωt is applied across it. The currents in R and L respectively will be
 .
.
View SolutionA circuit contains resistance R and an inductance L in series. An alternating voltage V=V0sinωt is applied across it. The currents in R and L respectively will be
 .
.
View Solution