The equation of a plane wave travelling along positive direction of x−axis is y=asin2πλ(vt−x) When the wave is reflected at a rigid surface and its amplitude becomes 80%, then find the equation of the reflected wave.
The correct Answer is:y'=0.8asin(2π)λ(vt+x+λ2).
To find the equation of the reflected wave when a plane wave traveling along the positive x-axis reflects off a rigid surface and its amplitude becomes 80%, we can follow these steps:
Step 1: Write the equation of the original wave
The equation of the wave traveling along the positive x-axis is given as:
y=asin(2πλ(vt−x))
Step 2: Determine the amplitude of the reflected wave
When the wave is reflected at a rigid surface, the amplitude of the reflected wave becomes 80% of the original amplitude. Therefore, the amplitude of the reflected wave is:
Areflected=0.8a
Step 3: Determine the direction of the reflected wave
The reflected wave travels in the opposite direction to the original wave. Therefore, we need to change the sign of the x term in the wave equation.
Step 4: Write the equation of the reflected wave
The general form of the wave traveling in the negative x-direction can be written as:
y=Asin(2πλ(vt+x+ϕ))
where ϕ is the phase difference due to reflection. Since the wave reflects off a rigid boundary, the phase difference is λ2.
Thus, substituting the amplitude and the phase difference, the equation of the reflected wave becomes:
y=0.8asin(2πλ(vt+x+λ2))
Final Equation
Therefore, the final equation of the reflected wave is:
y=0.8asin(2πλ(vt+x+λ2))
---
The equation of the wave traveling along the positive x-axis is given as:
y=asin(2πλ(vt−x))
When the wave is reflected at a rigid surface, the amplitude of the reflected wave becomes 80% of the original amplitude. Therefore, the amplitude of the reflected wave is:
Areflected=0.8a
The reflected wave travels in the opposite direction to the original wave. Therefore, we need to change the sign of the x term in the wave equation.
The general form of the wave traveling in the negative x-direction can be written as:
y=Asin(2πλ(vt+x+ϕ))
where ϕ is the phase difference due to reflection. Since the wave reflects off a rigid boundary, the phase difference is λ2.
y=0.8asin(2πλ(vt+x+λ2))
Therefore, the final equation of the reflected wave is:
y=0.8asin(2πλ(vt+x+λ2))
Topper's Solved these Questions
- TRAVELLING WAVESBook:RESONANCE ENGLISHChapter:TRAVELLING WAVESExercise:Exercise- 1 PART II
Explore 30 Videos
- TRAVELLING WAVESBook:RESONANCE ENGLISHChapter:TRAVELLING WAVESExercise:Exercise- 2 PART I
Explore 21 Videos
- TRAVELLING WAVESBook:RESONANCE ENGLISHChapter:TRAVELLING WAVESExercise:Board Level Exercise
Explore 27 Videos
- TEST SERIESBook:RESONANCE ENGLISHChapter:TEST SERIESExercise:PHYSICS
Explore 130 Videos
- WAVE ON STRING Book:RESONANCE ENGLISHChapter:WAVE ON STRING Exercise:Exercise- 3 PART II
Explore 7 Videos
Similar Questions
Knowledge Check
Equation of a plane progressive wave is given by y=0.6sin2π(t−x2). On reflection from a denser medium, its amplitude becomes 2/3 of the amplitude of the incident wave. The equation of the reflected wave is
Ay=0.6sin2π(t+x2)By=−0.4sin2π(t+x2)Cy=0.4sin2π(t+x2)Dy=−0.4sin2π(t−x2)
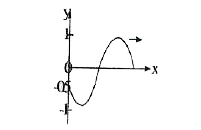
the equation of a wave travelling along the positive x - axis ,as shown in figure at t = 0 is given by
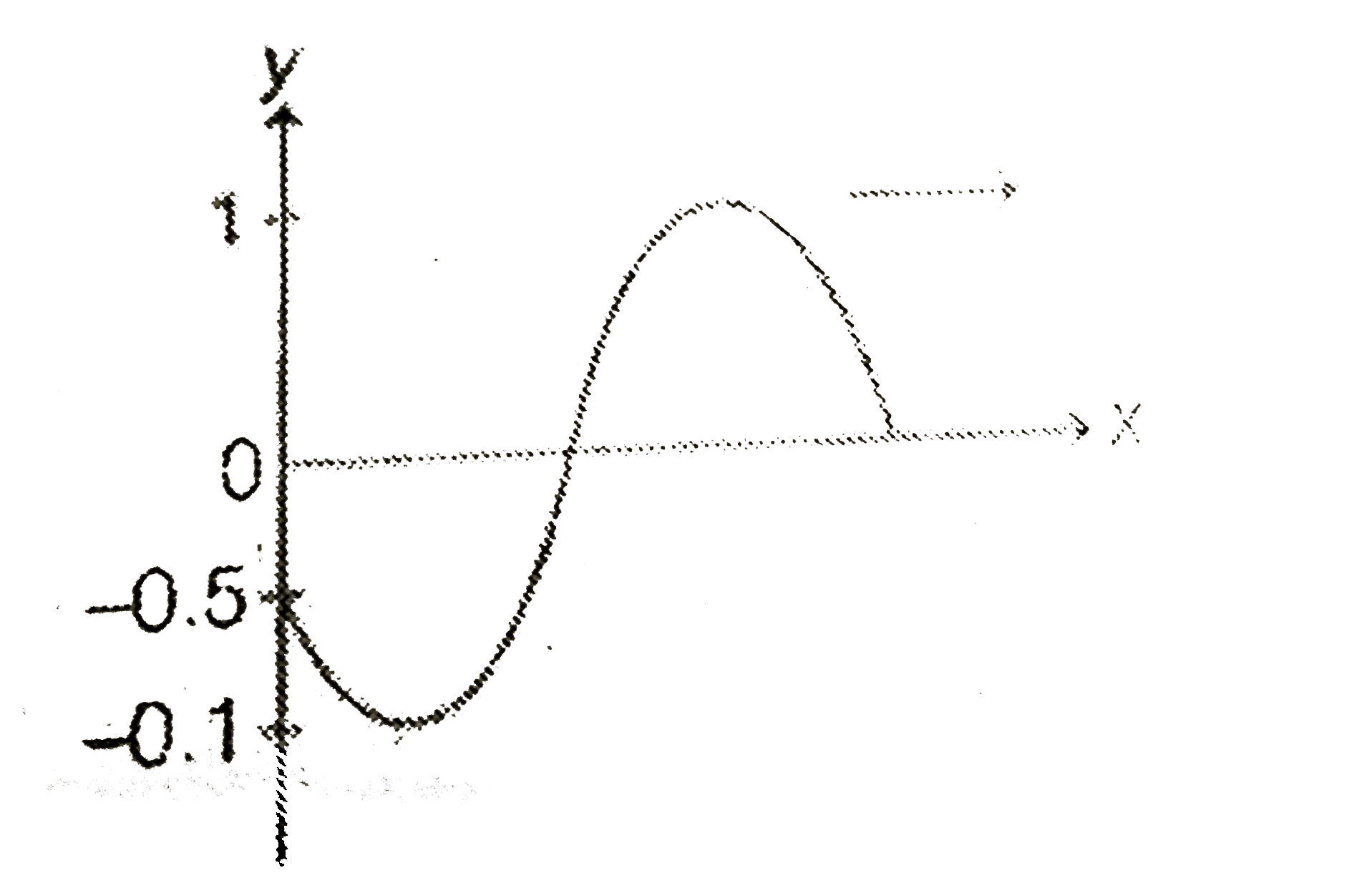
View SolutionA wave travelling along positive x-axis is given by y=Asin(ωt−kx). If it is reflected from rigid boundary such that 80% amplitude is reflected, then equation of reflected wave is
View SolutionEquation of a plane progressive wave is given by y=0.6sin2π(t−x2). On reflection from a denser medium, its amplitude becomes 2/3 of the amplitude of the incident wave. The equation of the reflected wave is
View SolutionThe equation of a transverse wave travelling along a string is y(xt)=0.3sin(200x−0.5t)
what is the equation of the reflected wave when it is reflected by
(i) A rigid boundary?
(ii) A free end?
View SolutionThe equation of a transverse wave travelling along +x axis is given by y=0.15 sin (1.57x-31.4t) where y and x are represented in m and t in s. Find the amplitude, frequency velocity and wavelength of the wave.
View SolutionWhich of the following equations represents as wave travelling along Y-axis?
View SolutionThe equation of a transverse wave travelling along x-axis is given by y = 10 sin π= (0.01 x- 2.0t) where y and x are represented in centimeters and, in seconds. Find the amplitude, frequency velocity and wavelength of the wave.
View Solution