An initially parallel cylindrical beam travels in a medium of refractive index μ(l)=μ0+μ2l, where μ0 and μ2 are positive constants and l is the intensity of the light beam. The intensity of the beam is decreasing with increasing radius.
The speed of light in the medium is
minimum the axis of the beam
the same everywhere in the beam
directly proportional to the intensity I
maximum on the axis of the beam
The correct Answer is:A
μ=μ0+μ2(I)
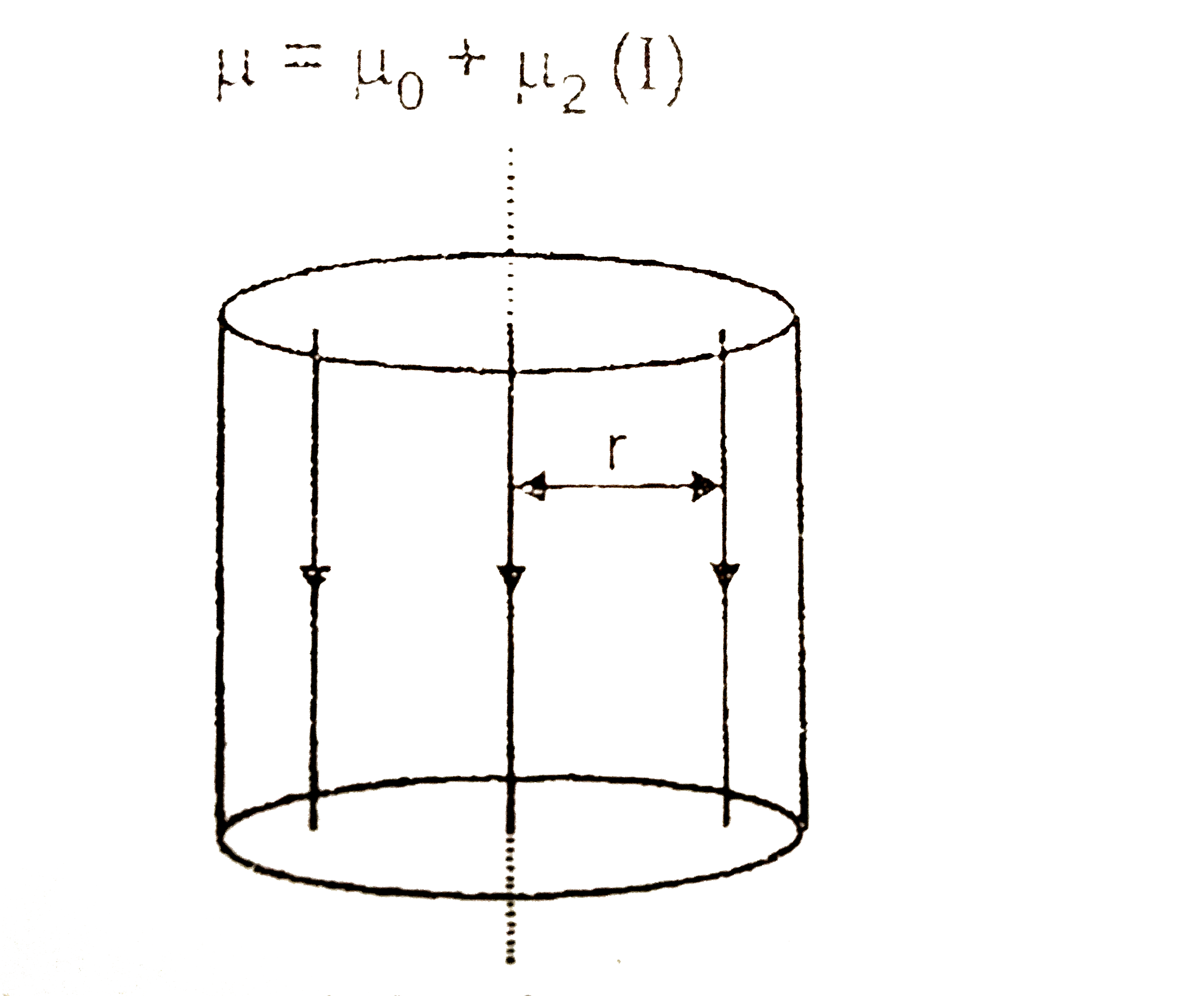
as it is given that intensity of beam is decreasing with increasing radius, and as I decreases μ also decreases.
Now by V=Cμ. Speed of light is minimum at axis of cylinder.
Topper's Solved these Questions
- WAVE OPTICSBOOK - RESONANCE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Exercise-3 (Part-3)
22 Videos
- WAVE OPTICSBOOK - RESONANCE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Advanced Level Problems
8 Videos
- WAVE OPTICSBOOK - RESONANCE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Exercise-3 (Part-1)
11 Videos
- WAVE ON STRING BOOK - RESONANCE ENGLISHCHAPTER - WAVE ON STRING EXERCISE - Exercise- 3 PART II
7 Videos
Similar Questions
An intially parallel cyclindrical beam travels in a medium of refractive index μ(I)=μ0+μ2I, where μ0 and μ2 are positive constants and I is intensity of light beam. The intensity of the beam is decreasing with increasing radius.
Answer the following questions :
The speed of light in the medium is
View SolutionAn intially parallel cyclindrical beam travels in a medium of refractive index μ(I)=μ0+μ2I, where μ0 and μ2 are positive constants and I is intensity of light beam. The intensity of the beam is decreasing with increasing radius.
Answer the following questions :
As the beam enters the medium, it will
View SolutionAn intially parallel cyclindrical beam travels in a medium of refractive index μ(I)=μ0+μ2I, where μ0 and μ2 are positive constants and I is intensity of light beam. The intensity of the beam is decreasing with increasing radius.
Answer the following questions :
The initial shape of the wavefront of the beam is
View SolutionIf a convex lens of refractive index μl is placed in a medium of refractive index μm Such that μl>μm>μair then
View SolutionRefractive index mu is given as μ=A+Bλ2, where A and B are constants and lambda is wavelength, then dimensions of B are same as that of
View SolutionRefractive index mu is given as μ=A+Bλ2, where A and B are constants and lambda is wavelength, then dimensions of B are same as that of
View SolutionIf light travels a distance of 5 m in a medium of refractive index μ, then equivalent path in vaccum it would travel in same time is
View SolutionIf ε0 and μ0 are respectively the electric permittivity and the magnetic permeability of free space and ε and μ the corresponding quantities in a medium, the refractive index of the medium is
View SolutionIf c is velocity of light in vacuum and v is the velocity of light in a medium of refractive index μ, then
View SolutionRefractive index of the material of concave lens is μ. The lens is immersed inb a medium of refractive index μ1(μ1>μ). A parallel beam of light is incident on the lens. Draw a diagram to show the path of emergent rays.
View Solution
