Find the radius of circle of illuminance, if a luminous object is placed at a distance h form the interface in denser medium.
To find the radius of the circle of illuminance when a luminous object is placed at a distance h from the interface in a denser medium, we can follow these steps:
1. Understanding the Setup:
- We have a luminous object in a denser medium with refractive index μd and a rarer medium with refractive index μr.
- The luminous object is placed at a height h above the interface between the two media.
2. Identifying the Critical Angle:
- The critical angle θc can be determined using Snell's Law, which states:
μdsinθc=μrsin90∘
- Since sin90∘=1, we can simplify this to:
sinθc=μrμd
3. Finding the Radius of the Circle of Illuminance:
- The radius r of the circle of illuminance can be determined using the relationship involving the tangent of the critical angle:
tanθc=rh
- Rearranging gives:
r=htanθc
4. Substituting for tanθc:
- From the relationship between sine and tangent:
tanθc=sinθccosθc
- We already have sinθc=μrμd. To find cosθc, we can use the identity:
cos2θc+sin2θc=1
- Thus:
cos2θc=1−(μrμd)2
- Therefore:
cosθc=√1−(μrμd)2
5. Putting It All Together:
- Now substituting back into the expression for tanθc:
tanθc=μrμd√1−(μrμd)2
- Substituting this into the equation for r:
r=h⋅μrμd√1−(μrμd)2
- Simplifying gives:
r=hμrμd√1−(μrμd)2
Final Result:
The radius of the circle of illuminance is given by:
r=hμrμd√1−(μrμd)2
- We have a luminous object in a denser medium with refractive index μd and a rarer medium with refractive index μr.
- The luminous object is placed at a height h above the interface between the two media.
- The critical angle θc can be determined using Snell's Law, which states:
μdsinθc=μrsin90∘
- Since sin90∘=1, we can simplify this to:
sinθc=μrμd
- The radius r of the circle of illuminance can be determined using the relationship involving the tangent of the critical angle:
tanθc=rh
- Rearranging gives:
r=htanθc
- From the relationship between sine and tangent:
tanθc=sinθccosθc
- We already have sinθc=μrμd. To find cosθc, we can use the identity:
cos2θc+sin2θc=1
- Thus:
cos2θc=1−(μrμd)2
- Therefore:
cosθc=√1−(μrμd)2
- Now substituting back into the expression for tanθc:
tanθc=μrμd√1−(μrμd)2
- Substituting this into the equation for r:
r=h⋅μrμd√1−(μrμd)2
- Simplifying gives:
r=hμrμd√1−(μrμd)2
The radius of the circle of illuminance is given by:
r=hμrμd√1−(μrμd)2
Topper's Solved these Questions
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-1
141 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-2
62 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Advance level Problems
35 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - HIGH LEVEL PROBLEMS
16 Videos
Similar Questions
A convex mirror has its radius of curvature 20 cm. Find the position of the image of an object placed at a distance of 12 cm from the mirror.

View SolutionFind the position, magnification and nature of the image of an object placed at a distance of 8 cm from a convex mirror of radius of curvature 24 cm.
View SolutionThe focal length of objective and the eyepiece of a compound microscope are 1cm and 5cm. An object is placed at a distance of 1.1 cm from the objective and if the final image is formed at infinity the magnifying power and distance between the lenses are respectively
View SolutionA thin equiconvex glass lens (μg=1.5) is beign placed on the top of a vessel of height h=20cm as shown figure. A luminous point source is beign placed at the bottom of vessel on the principal axis of the lens. When the air is on both the side of the lens the image of luminous source is formed at a distance of 20cm from the lens out side the vessel. When the air inside the vessel is being replaced by a liquid of refractive index μl the image of the same source is being formed at a distance 30cm from the lens outside the vessel. Find the μl.
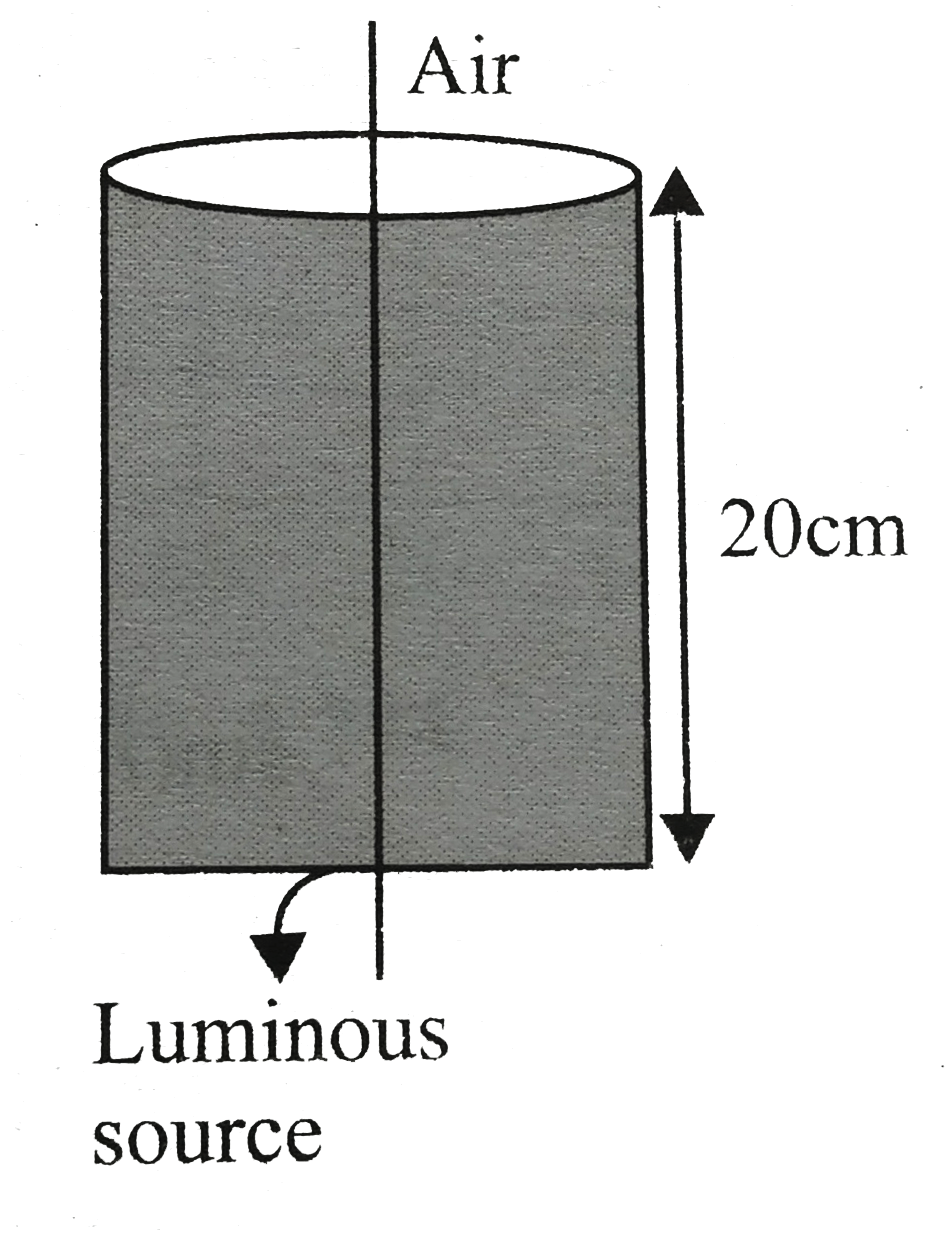
View SolutionAn image is formed at a distance of 100 cm from the glass surface with refractive index 1.5, when a point object is placed in the air at a distance of 100 cm from the glass surface. The radius of curvature is of the surface is
View SolutionThe two lenses of a compound microscope are of focal lengths 2 cm and 5 cm. If an object is placed at a distance of 2.1 cm from the objective of focal length 2 cm the final image forms at the final image forms at the least distance of distinct vision of a normal eye. Find the magnifying power of the microscope.
View SolutionAssertion : Focal length of a convex mirror is 20 cm. If a real object is placed at distance 20 cm from the mirror, its virtual erect and diminished image will be formed.
Reason : If a virtual object is placed at 20 cm distance, its image is formed at infintiy.
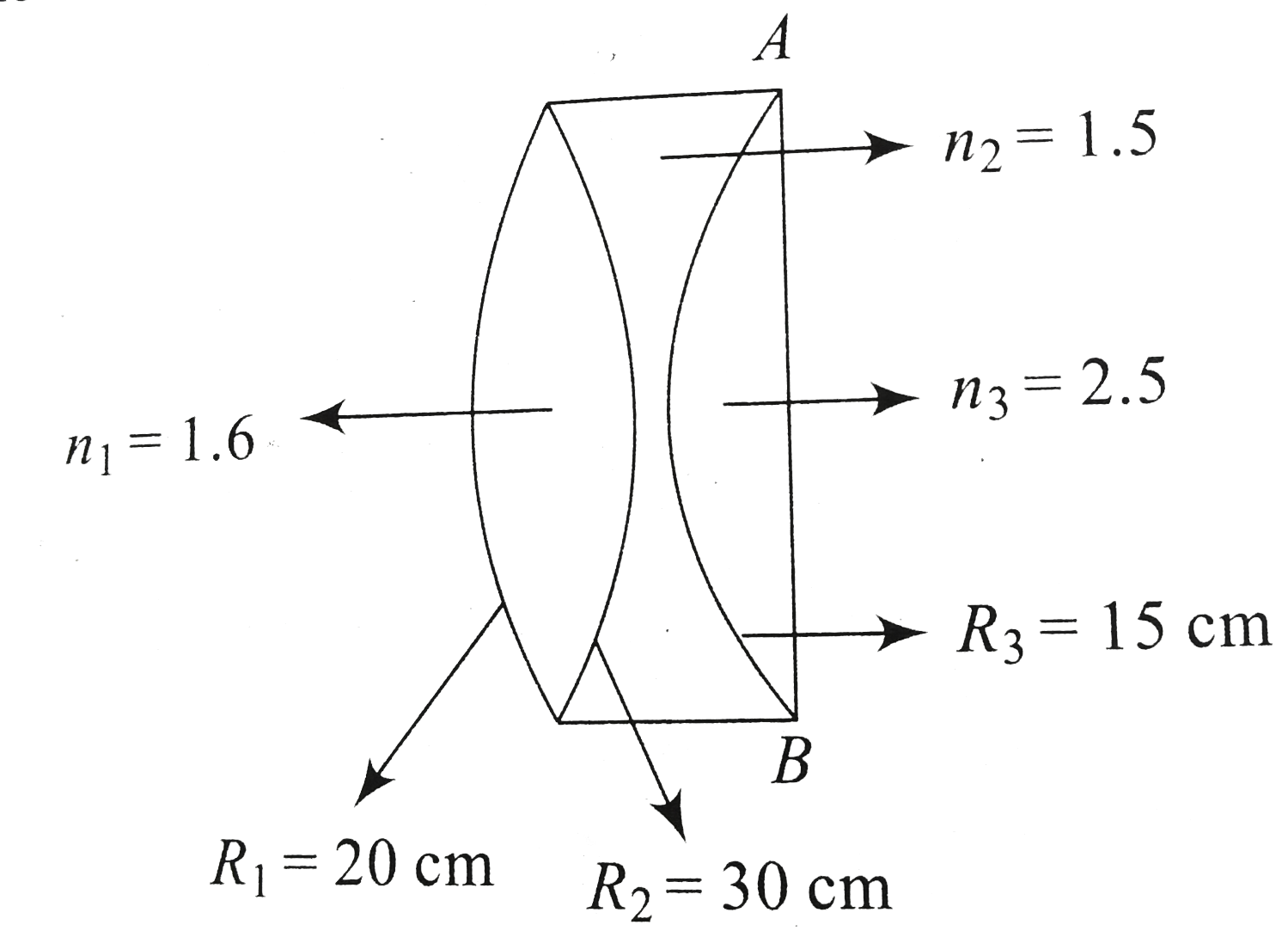
View SolutionA lens is made of three thin different mediums. Radius of curvature and refractive index of each medium is shown iin Figure., Surface AB is straight. An object is placed at some distance from the lens by which a real image is formed on the screen placed at a distance of 10cm from the lens. Find the distance of the object from the lens.
View SolutionA concave mirror having a radius of curvature 40 cm is placed in front of an illuminated point source at a distance of 30 cm from it. Find the location of the image.
View SolutionA convex mirror of radius of curvature 1.6m has an object placed at a distance of 1m from it. The image is formed at a distance of
View Solution
