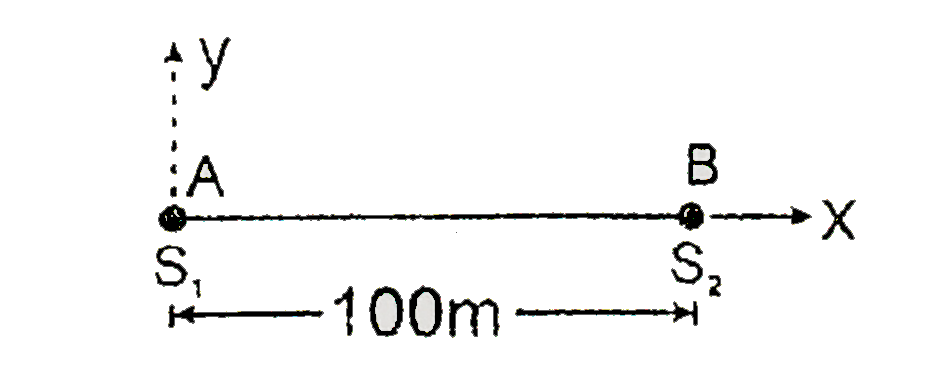
In the figure shown $A$ and $B$ are two ends of a string of length $100 m$ . $S_{1}$ and $S_{2}$ are two sources due to which point $' A'$ and $' B'$ oscillate in $' y'$ and $' z'$ directions respectively according to the equation $y = 2 sin (100 π t + 30^{\circ})$ and $z = 3 sin (100 π t + 60^{\circ})$ where $t$ is in $sec$ and $y$ is in $m m$ . The speed of propagation of disturbance along the string is $50 m / s$ . Find the instantaneous positions vector (in $m m$ ) and velocity vector in $(m / s)$ of a particle $' P'$ of string which is at $25 m$ from $A$ . You have to find these parameters after both the disturbance from $S_{1}$ and $S_{2}$ have reached $' P'$ . Also find the phase difference between the waves at the point $' P'$ when they meet at $' P'$ first time.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:Phase of difference at time $' t' = 30^{0}$ constant al-ways after thy meet at $' P'$

$y_{p} = 2 sin (100 π t + 30^{\circ})$
$y = 2 sin [100 π t (t - \frac{25}{50}) + 30^{\circ}] = 2 \subset (100 p t - 50 p + 30^{0})$
$z = 3 sin [100 π (t - \frac{75}{50}) + 60^{0}]$
$= 3 sin (100 π t - 150 π + 60^{0}) = 3 sin (100 π t + 60^{0})$
$\to r_{in mm} = x ˆ i + y ˆ j + z ˆ k$
$= 25000 ˆ i + 2 sin (100 π + 30^{0}) ˆ j + 3 sin (100 π t + 60^{0}) ˆ k$
$\to v = \frac{d \to r}{d t} = 2 \times 100 π cos (100 π t + 30^{0}) ˆ i + 3 \times 10 π cos (100 π t ˆ j + 60^{0}) ˆ k$
Phase difference at time $' t' = 30^{0}$ costant always after they meet at $' P'$ .

Updated on:21/07/2023

|

Class 12 PHYSICS WAVE ON STRING

Topper's Solved these Questions

Similar Questions

RESONANCE ENGLISH-WAVE ON STRING -Exercise- 3 PART I

Home
Ask Doubt
Profile