At high pressure suppose all the constant temperature curve varies linearly with pressure according to the following equation : Z=1+PbRT (R=2calmol−1K−1)
Plot at Boyle's temperature for the gas will be
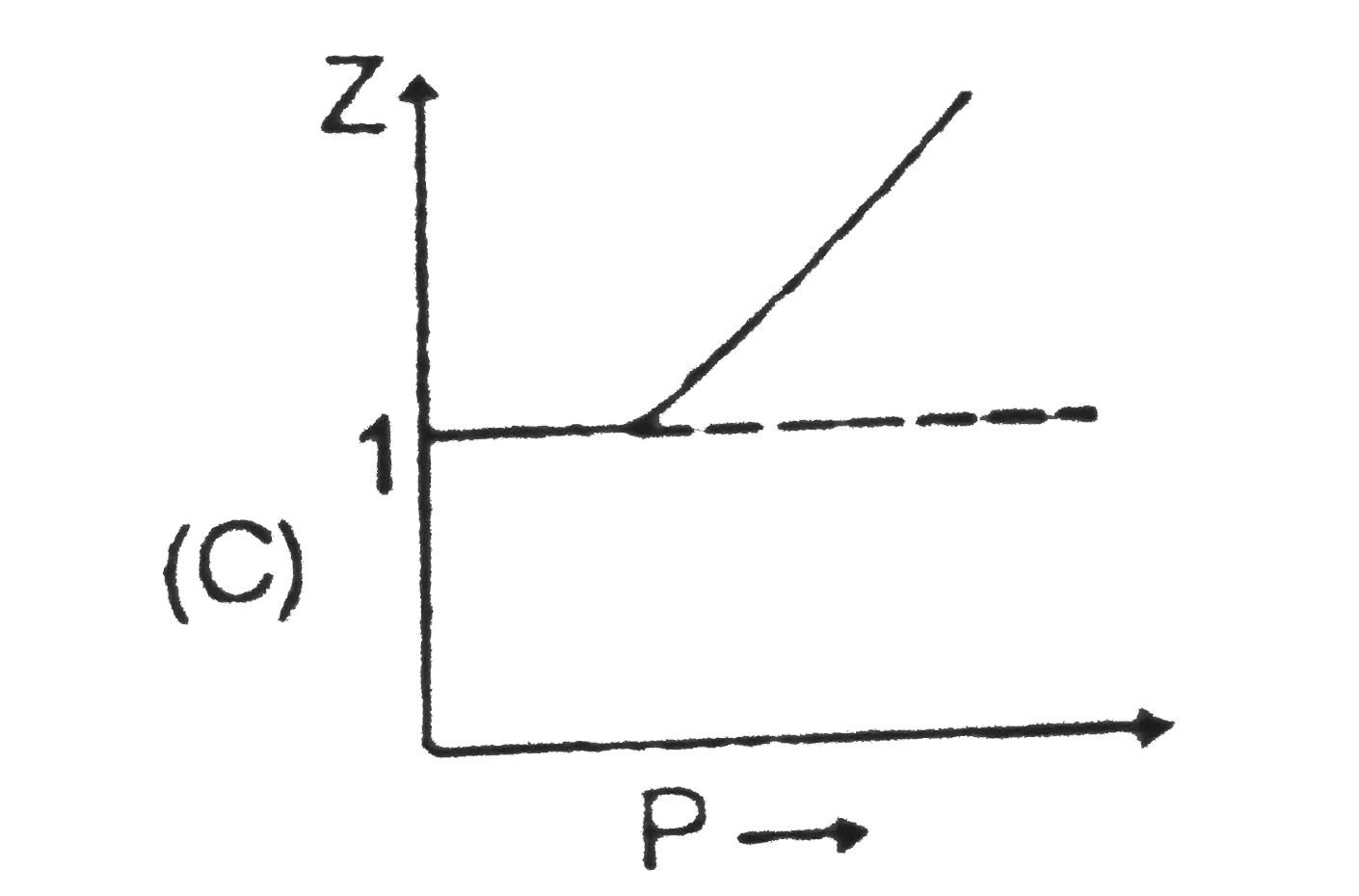
The correct Answer is:C
To solve the problem, we need to analyze the given equation and understand the implications of Boyle's temperature for a gas. Let's break it down step by step.
Step 1: Understand the Equation
The equation provided is:
Z=1+PbRT
where:
- Z is the compressibility factor,
- P is the pressure,
- b is a constant specific to the gas,
- R=2calmol−1K−1 is the universal gas constant,
- T is the temperature.
Step 2: Define Boyle's Temperature
Boyle's temperature (TB) is the temperature at which a real gas behaves ideally. At this temperature, the compressibility factor Z approaches 1 at high pressures.
Step 3: Analyze the Equation at Boyle's Temperature
At Boyle's temperature, we can set T=TB:
Z=1+PbRTB
At P=0 (the ideal gas limit), we have:
Z=1
As pressure increases, Z will increase according to the equation. The relationship indicates that as P increases, Z will linearly increase due to the term PbRT.
Step 4: Plotting the Graph
To plot the graph of Z versus P:
1. At P=0, Z=1.
2. As P increases, Z increases linearly based on the equation Z=1+PbRTB.
The graph will start at the point (0, 1) and will have a slope of bRTB.
Step 5: Conclusion
The plot at Boyle's temperature will be a straight line starting from the point (0, 1) and increasing linearly with pressure.
Final Answer
The plot at Boyle's temperature for the gas will be a straight line starting from Z=1 at P=0 and increasing linearly with pressure.
---
The equation provided is:
Z=1+PbRT
where:
- Z is the compressibility factor,
- P is the pressure,
- b is a constant specific to the gas,
- R=2calmol−1K−1 is the universal gas constant,
- T is the temperature.
Boyle's temperature (TB) is the temperature at which a real gas behaves ideally. At this temperature, the compressibility factor Z approaches 1 at high pressures.
At Boyle's temperature, we can set T=TB:
Z=1+PbRTB
Z=1
To plot the graph of Z versus P:
1. At P=0, Z=1.
2. As P increases, Z increases linearly based on the equation Z=1+PbRTB.
The plot at Boyle's temperature will be a straight line starting from the point (0, 1) and increasing linearly with pressure.
The plot at Boyle's temperature for the gas will be a straight line starting from Z=1 at P=0 and increasing linearly with pressure.
Topper's Solved these Questions
Similar Questions
Sketch shows the plot of Z v/s P for a hypothetical gas for one mole at three distint temperature.
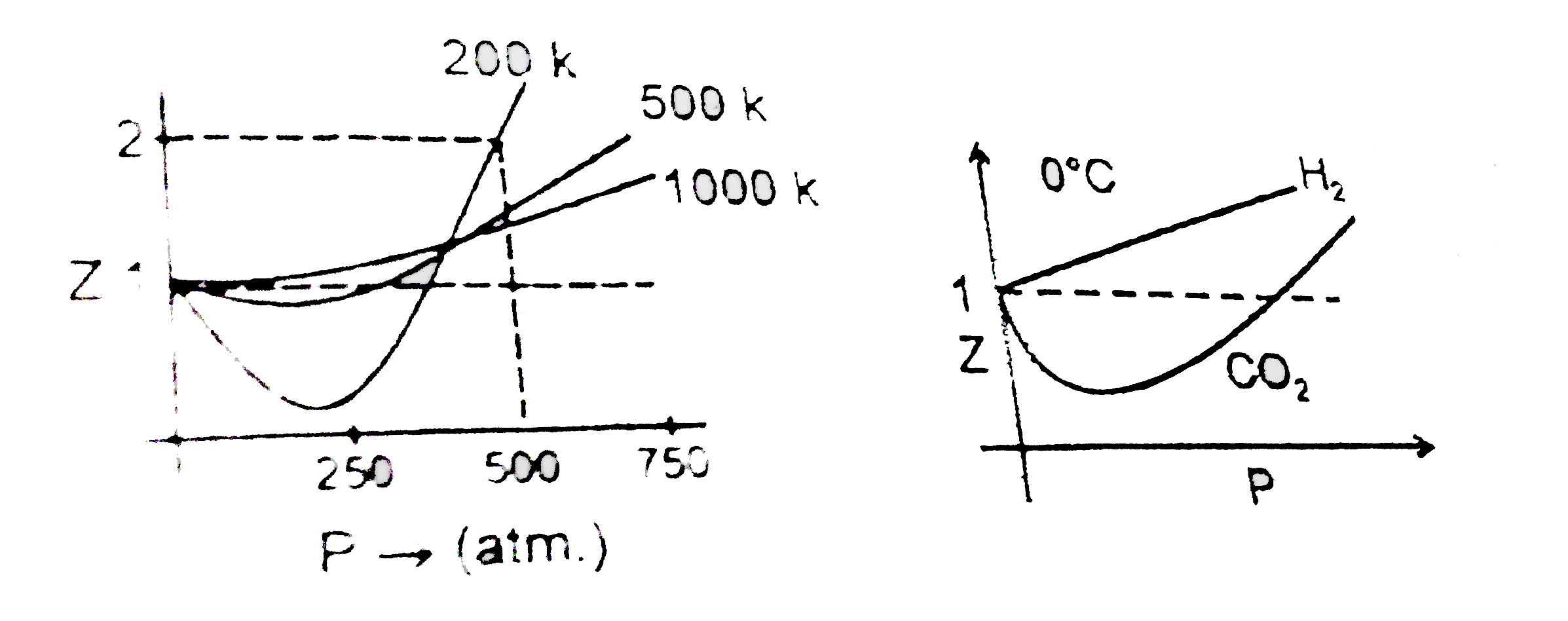
Boyle's temperature is the temperature at which gas shows ideal behaviour over a pressure range in the low pressure region.Boyle's temperature (Tb)=aRb.If a plot is obtained at temperature well below Boyle's temperature then the curve will show negative deviation, in low pressure region and positive deviation in the high pressure region. Near critical temperature the curve is more likely as CO2 and the temperature well above critical temperature curve is more like H2 at 0∘C as shown above.At high pressure suppose all the constant temperature curve varies linearly with pressure according to the following equation : Z=1+PbRT (R=2calmol−1K−1)
Which of the following is correct :
View SolutionSketch shows the plot of Z v/s P for a hypothetical gas for one mole at three distint temperature.
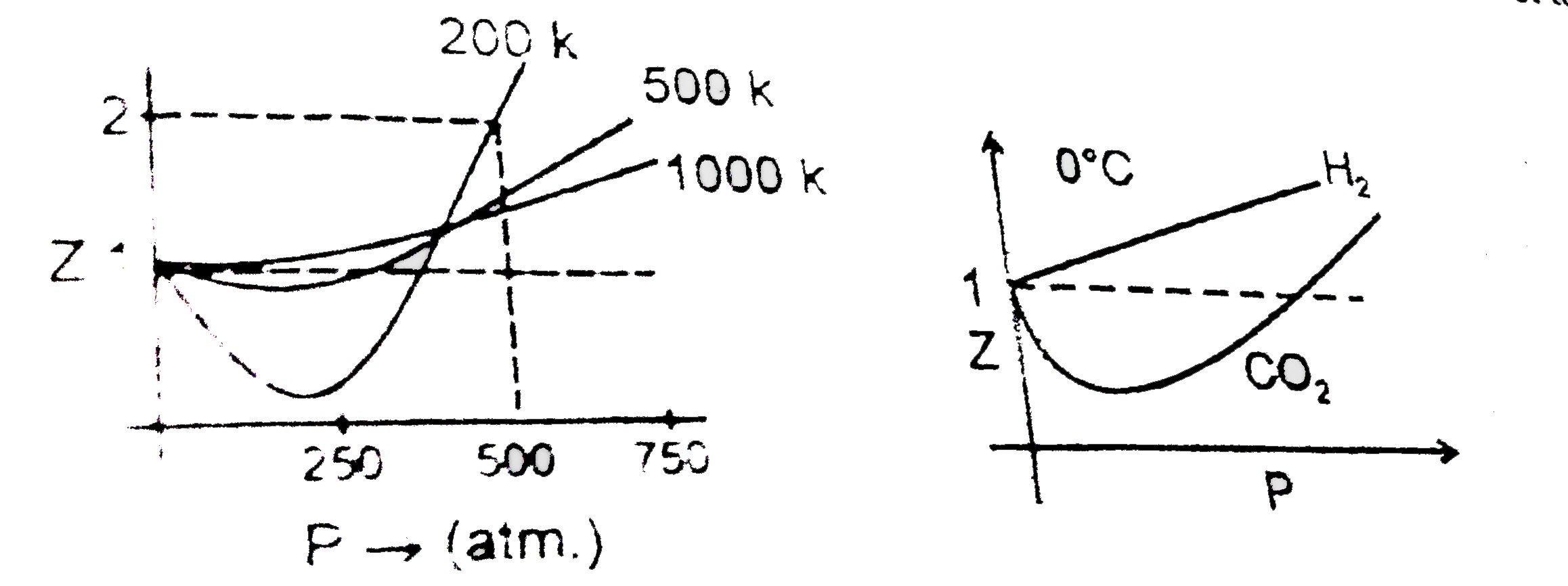
Boyle's temperature is the temperature at which gas shows ideal behaviour over a pressure range in the low pressure region.Boyle's temperature (Tb)=aRb.If a plot is obtained at temperature well below Boyle's temperature then the curve will show negative deviation, in low pressure region and positive deviation in the high pressure region. Near critical temperature the curve is more likely as CO2 and the temperature well above critical temperature curve is more like H2 at 0∘C as shown above.At high pressure suppose all the constant temperature curve varies linearly with pressure according to the following equation : Z=1+PbRT (R=2calmol−1K−1)
For 500 K plot value of Z changes from 2 to 2.2 if pressure is varied from 1000 atm to 1200 atm (high pressure ) then the value of bRT will be
View SolutionSketch shows the plot of Z vs P for 1 mol of a hypothetical gas at three distinct temperature.
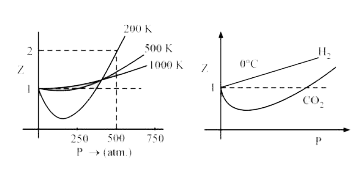
Boyle’s temperature is the temperature at which a gas shows ideal behaviour over a pressure range in the low pressure region. Boyle’s temperature (Tb)=aRb. If a plot is obtained at temperatures well below Boyle’s temperature then the curve will show negative deviation, in low pressure region and positive deviation in the high pressure region. Near critical temperature the curve is more like CO2 and the temperature well above critical temperature curve is more like H2 as shown above. At high pressure suppose all the constant temperature curve varies linearly with pressure according to the following equation: Z=1+PbRT(R=2calmol−1K−1)
For 500 K plot the value of Z changes from 2 to 2.2 if pressure is varied from 1000 atm to 1200 atm (high pressure) then the value of bRT will be :
View SolutionSketch shows the plot of Z v/s P for a hypothetical gas for one mole at three distint temperature.

Boyle's temperature is the temperature at which gas shows ideal behaviour over a pressure range in the low pressure region.Boyle's temperature (Tb)=aRb.If a plot is obtained at temperature well below Boyle's temperature then the curve will show negative deviation, in low pressure region and positive deviation in the high pressure region. Near critical temperature the curve is more likely as CO2 and the temperature well above critical temperature curve is more like H2 at 0∘C as shown above.At high pressure suppose all the constant temperature curve varies linearly with pressure according to the following equation : Z=1+PbRT (R=2calmol−1K−1)
In very high pressure region if Z v/s P is plotted at 1200 K for the above gas then it will have greatest slope.
View SolutionAt a constant temperature a gas is initially at 2 atm pressure. To compress it to 1/8th of its initial volume, pressure to be applied is
View SolutionAt constant temperature, the effect of change of pressure on volume of a gas was as given below

Plot the following graphs :
P vs 1/V
View SolutionAt constant temperature, the effect of change of pressure on volume of a gas was as given below

Plot the following graphs :
P vs V
View SolutionThe volume of 1 mol of a gas at standard temperature and pressure is .
View SolutionAt constant temperature, the effect of change of pressure on volume of a gas was as given below

Plot the following graphs :
PV vs P.
View SolutionThe change in the magnitude of the volume of an ideal gas when a small additional pressure ΔP is applied at a constant temperature, is the same as the change when the temperature is reduced by a small quantity ΔT at constant pressure. The initial temperature and pressure of the gas were 300 K and 2 atm. respectively. If |ΔT|=C|ΔP| then value of C in (K/atm) is __________.
View Solution
