A reflecting surface is represented by the equation
, where . A ray of light travelling horizontally becomes vertical after reflection with the surface. The co-ordinates of the point where this ray is incident is.
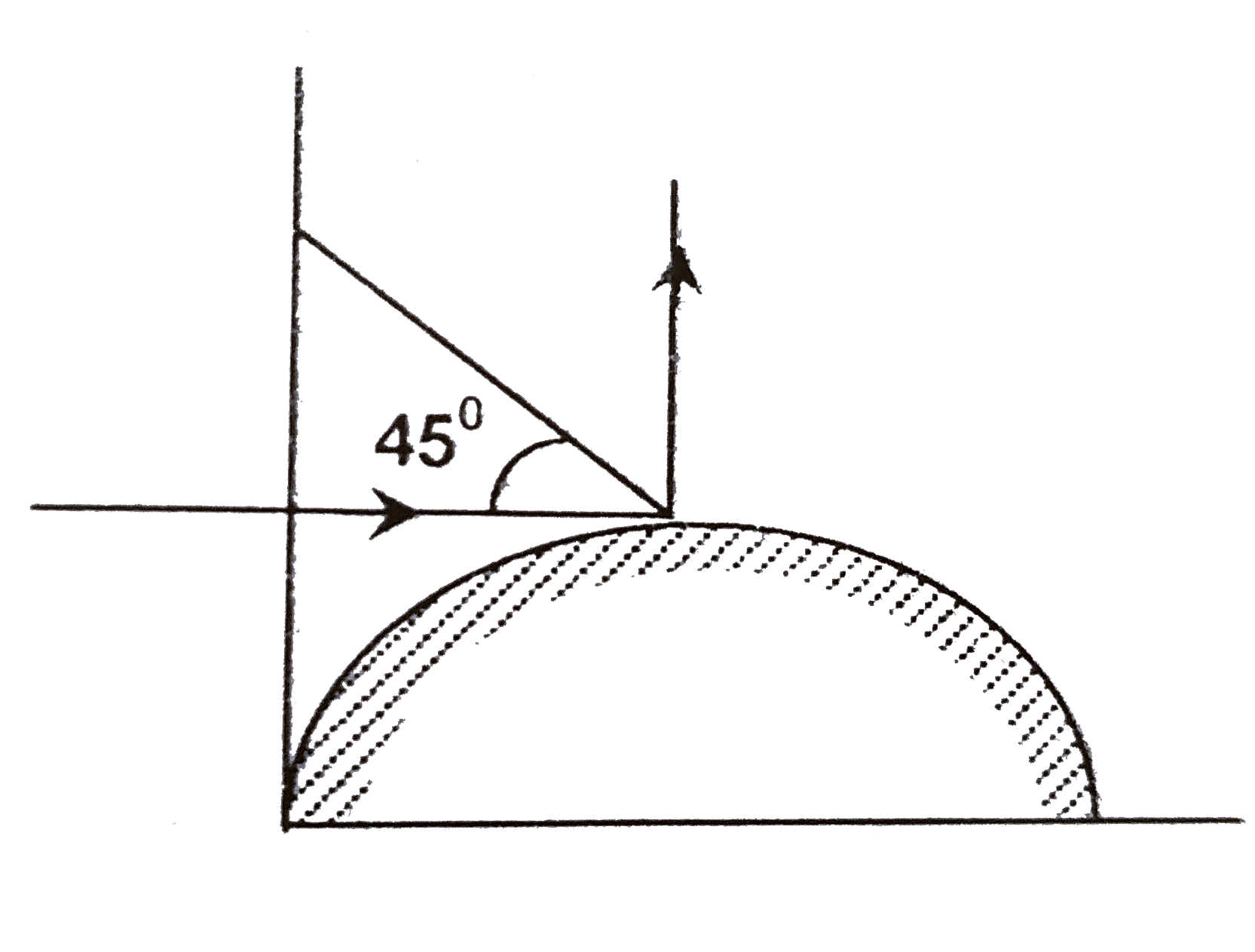
The correct Answer is:B
Topper's Solved these Questions
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PART - II PHYSICS
Explore 106 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Advanced Level Problems
Explore 13 Videos
- TEST SERIESBook:RESONANCE ENGLISHChapter:TEST SERIESExercise:PHYSICS
Explore 130 Videos
Similar Questions
A refracting surface is represented by the equation x2+y2=a2. A ray travelling in negative x-directed towards positive y-direction after reflection from the surface at point P. Then co-ordinates of point P are
View SolutionSolve the equation (sinx+cosx)1+sin2x=2 , when −π≤x≤π .
View SolutionIf the equation of mirror is given by y=2/πsinπx(y>0,0≤x≤1) then find the point on which horizontal ray should be incident so that the reflected ray become perpendicular to the incident ray
_E01_073_Q01.png)
View SolutionSolve the equation for 0≤x≤2π.
sinxcosx=0
View SolutionSolve the equation for 0≤x≤2π.
2sinx−1=0
View SolutionThe number of distinct real roots of the equation √sinx−1√sinx=cosx(where0≤x≤2π) is
View SolutionThe area bounded by the curves y=sin−1|sinx|andy=(sin−1|sinx|)2, where 0≤x≤2π, is
View SolutionSolve the equation for 0≤x≤2π.
2sin2θ=3cosθ
View SolutionSolve 2cos2x+sinx≤2, where π/2≤x≤3π/2.
View SolutionIf a ray travelling the line x = 1 gets reflected the line x+y=1 then the equation of the line along which the reflected ray travels is
View Solution